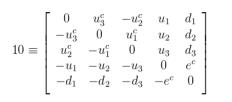2
$\begingroup$

For example, in this paper on page 21 the authors write the vev that breaks $SO(10)$ to $SU(4)\times SU(2) \times SU(2)$

$$ <54>= 1/5 \cdot diag( -2,-2,-2,-2,-2,-2,3,3,3,3) \omega_s$$

where $\omega_s$ denotes the scale.

What do the authors mean by this?

The Higgs field or Higgs fields that develop a vev are elements of the $54$ representation of $SO(10)$. Because $10 \otimes 10 = 1_s \oplus 54_s \oplus45_a$, we can write each element of $54$ as a $10 \times 10$ matrix.

This is similar how one determines the particle content of the $10$ representation of $SU(5)$, written as $5 \times 5$ matrix, by using $5 \otimes 5 =10 \oplus \ldots$.

The quantum numbers of the 10 inedpendent fields $\in$ 10 are given by

$$ QN(10_{ij})= QN(5_i) + QN(5_j) ,$$ where $10_{ij}$ denotes the $ij$ element of the $5 \times 5$ matrix.

This yields

enter image description here

Completely equivalently we can determine the quantum numbers of the Higgs fields in the $54$ representation of $S0(10)$ and write them in a $10 \times 10$ matrix.

How does $ <54>= 1/5 \cdot diag( -2,-2,-2,-2,-2,-2,3,3,3,3) \omega_s$ tell me which of these $54$ Higgs fields gets a vev?

Improve this question
$\endgroup$
4
  • $\begingroup$ The expression you write gives a traceless symmetric $10 \times 10$ matrix, which hence sits in the $54$ of $SO(10)$. $\endgroup$
    – Olof
    Commented Jul 31, 2015 at 15:45
  • $\begingroup$ @Olof yes I agree with you... But then what does the vev= diag(... mean? $\endgroup$
    – jak
    Commented Jul 31, 2015 at 15:49
  • $\begingroup$ it means that the vacuum expectation value is chosen to be proportional to that particular diagonal matrix $\endgroup$
    – Olof
    Commented Jul 31, 2015 at 19:30
  • $\begingroup$ @Olof Yes, again I agree, but my problem remains. For concreteness let's consider a simplified setup. A computation similar to $QN(10_{ij})= QN(5_i) + QN(5_j) ,$ which yields for example the 10 of $SU(5)$ as quoted in the OP, yields a matrix for the Higgs bosons. For example, in a $2 \times 2$ case: $ \begin{pmatrix} A & B \\ C& D \end{pmatrix} $, where $A,B,C,D$ denote four different Higgs fields. Now given a vev = $diag(1,-1)w$, does this mean the Higgs field $A$ gets a vev $w$ and the Higgs field $D$ gets a vev $-w$?. $\endgroup$
    – jak
    Commented Aug 1, 2015 at 4:58

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.