While reading this article by Abbot on the background field method, in Fig 5. on page 38 (page 6 in the pdf file), we can see the relation between connected contributions to the two point function and the 1PI contributions
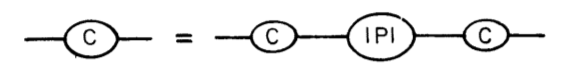
Of course, we can recursively insert this expression for connected diagrams to obtain a chain
C-1PI-C-1PI-C-1PI-C-1PI-C-1PI-C-1PI-C-1PI-C-1PI-C-1PI-C
which can be extended as much as we want.
Nonetheless, while reading this notes of Matthew Schwartz, in eq. 8 on page 3 we can see
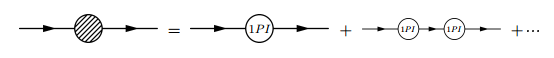
where only 1PI-s are considered.
Are both this expressions equivalent? if so why?
