In an attempt of reducing the eddy currents in a conductor, such as having gaps to break the induced eddy currents, would that by any chance change the conductor's orientation from series to parallel?
An example, from the following diagrams:
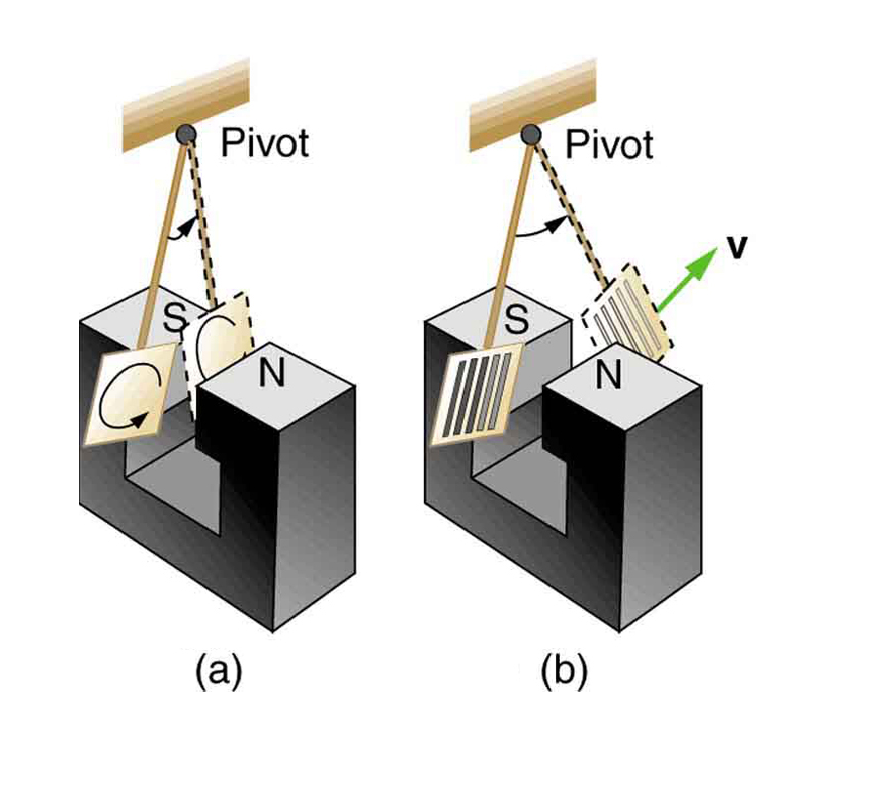
Imagine both diagrams we're connected to a separate circuit somehow. Diagram(a) is a complete conductor inducing high eddy currents, also a series layout with respect to the circuit. However, if diagram (b) was connect to a circuit, would the conductor be oriented to a parallel circuit? Diving out the input current flow all around the conductor or is it still in series, regardless of the air gaps?
I guess, this goes deeper into my understanding of parallel/series circuit, I would understand that it's usually the components(resistors/diodes/inductors/ etc...) that are connected either in series, or parallel to a circuit. But what about a single individual wire, could it be broken down into the same concept?
Wires usually are in series, one whole piece of conductor, when it brakes into branches that would indicate a parallel circuit being formed, but what about the conductor in diagram(b) then?
A bit, odd comparing a single wire to a whole circuit but I hope the question(s) are clear enough.
