0
$\begingroup$

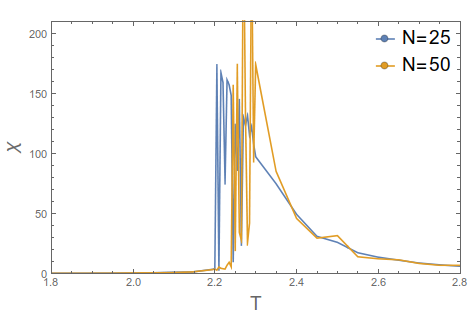
I'm trying to simulate Ising model using metropolis algorithm. Boundary conditions are periodic. I know how the algorithm works and I have written the code myself. Everything works as it should except around critical temperature.

I started my calculation at temperature T=8 (Boltzmann constant is 1). And then decreased the temperature in steps by 0.05. At T=2.35 I shortened the step to 0.005 till T=2.2 and after 2.2 the step was again 0.05. At each temperature I waited 15 million flips before I started sampling magnetization. Then I flipped the spins 25 million times and every 50th flip I sampled magnetization. This is necessary in order to have statistically uncorrelated samples. Btw, I am using Mersenne twister from gsl to generate random numbers.

Still around critical temperature the algorithm does not work good enough. Magnetization is jumping up and down. I don't really understand why that is, because I think I took enough samples to average out extremes. If someone can help me, I'll be really happy. Below are pictures of magnetization, susceptibility and specific heat.

enter image description here

enter image description here

enter image description here

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ You're simply not averaging over enough runs. $\endgroup$
    – lemon
    Commented Apr 17, 2015 at 19:19
  • $\begingroup$ The algorithm is taking quiet a long time to finish, so I can't really increase the number of samples by a lot. Now I'm sampling magnetization after 25th flip of spin, and I also increased 25 million to 35 million flips when sampling and it doesn't seem to help. Another question I have is, whether do I have to average absolute value of magnetization or no. Now I just sum magnetization without changing the sign to +, around critical temperature it seem to be jumping from - to +. The graph above shows absolute of average magnetization. $\endgroup$
    – Jur
    Commented Apr 17, 2015 at 20:26
  • $\begingroup$ From figure 1 and especially figure 2 I can see you are incorrectly sampling magnetization. Are you using some sort of cluster algorithm i.e. Wolf algorithm ? You should sample the absolute value of magnetization, since it can change sign. P.S. From figure 3 it can be seen you are correctly sampling energy. $\endgroup$
    – user78758
    Commented Apr 25, 2015 at 21:06
  • $\begingroup$ Those graphs are actually from one single run of algorithm. I sampled <E>, <E^2>, <M> and <M^2> and then calculated different things. After I started sampling absolute value of magnetization things got better, however, the lines are still not smooth - still some ups and down occur at critical temperature. I tried wolff algorithm and (for me) it works a lot better than metropolis. $\endgroup$
    – Jur
    Commented Apr 27, 2015 at 7:34
  • $\begingroup$ This is called critical slow down. One way to get better statistics and speed up the simulation is the Swendsen-Wang algorithm for flipping clusters of spins rather than individual spins. $\endgroup$
    – ZachMcDargh
    Commented May 11, 2015 at 14:01

1 Answer 1

Reset to default
1
$\begingroup$

What you are observing is the critical slowdown - getting to the equilibrium near to the critical temperature is very hard because of huge fluctuations in the system.

Therefore, as you are not averaging over enough runs, it is no wonder that you are getting very large deviations from the (expected) average values. This problem is less severe for more sophisticated algorithms, e.g. the Wolff algorithm, as they allow for large (for example, nonlocal) differences of states in adjacent time steps.

There are many references on critical slowdown online; Googling for "critical slowdown ising" returns videos of lectures, lecture notes, as well as research papers.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.