1
$\begingroup$

In cars as well as bicycles, when we are on a lower gear, the driving wheel (the one on the wheels) has a bigger radius compared to when on a higher gear.

So on a lower gear the bike/car would move lesser compared to the higher gear for the same no. of revolutions of the engine.

Now my question is, why does that fact make it easier to go on a uphill on a smaller gear? Does that prevent slipping? Beacuse torque applied is more? Or what?

Can someone explain with a free body diagram?

Improve this question
$\endgroup$
1
  • $\begingroup$ It's all about power. Lower gears require more revolutions (and therefore less energy per revolution) to achieve the same distance. So the power requirement is less. $\endgroup$
    – lemon
    Commented Feb 26, 2015 at 8:28

5 Answers 5

Reset to default
2
$\begingroup$

The power input is roughly constant (that of a car is dictated by the total engine power while for a bicycle it depends on the user). The gear or similar tools adjusts the mechanical advantage so that a low gear will express the engine power in force rather than speed (recall that power is force times speed). On higher gears the force is traded in for speed.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So, at higher speeds the bicycle will slip on an uphill, but at lower speeds and more torque, it wont? That is why we use lower gears? To avoid slipping? $\endgroup$
    – Black Dagger
    Commented Feb 26, 2015 at 10:14
  • $\begingroup$ assuming you have the strength to pedal uphill with a high gear ratio, slipping could happen. But, like a car, you might fail to even start moving (in a car the engine would go off, or if you manage to keep it "alive" it might burn). So in principle you can start moving uphill with a low gear ratio and then gear up when necessary. $\endgroup$
    – Phoenix87
    Commented Feb 26, 2015 at 10:30
2
$\begingroup$

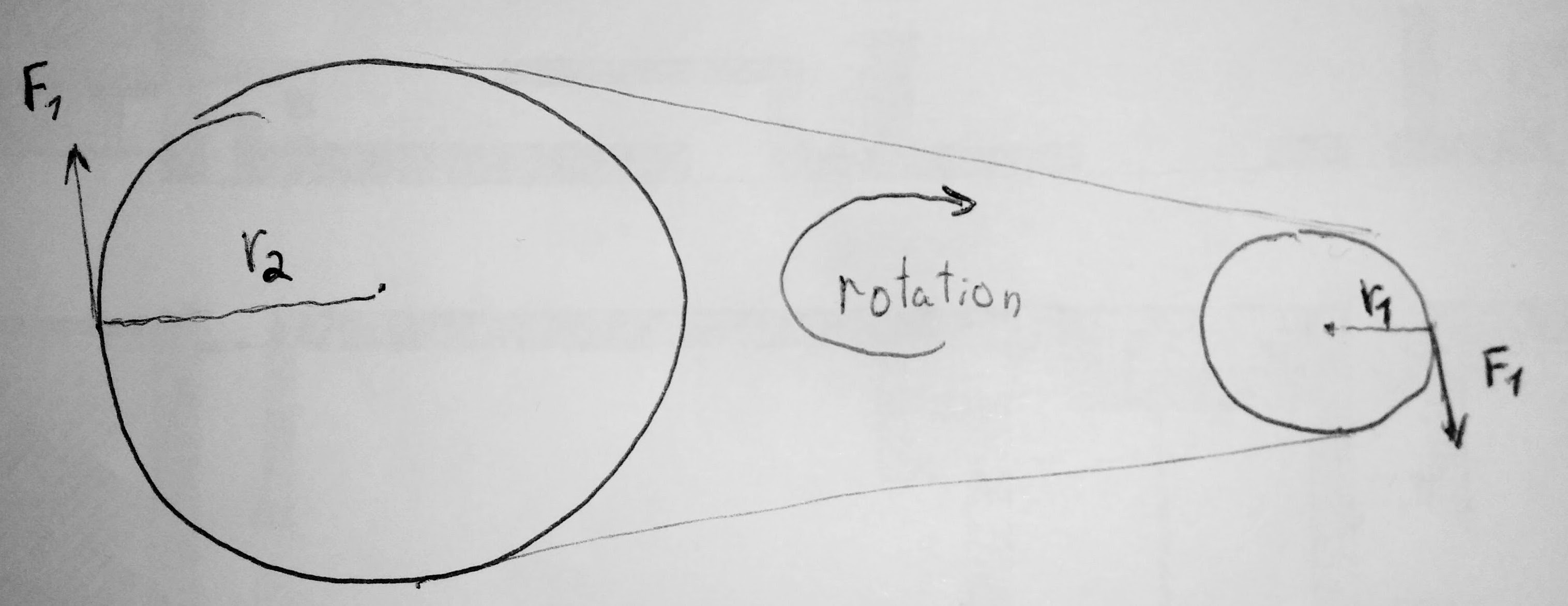
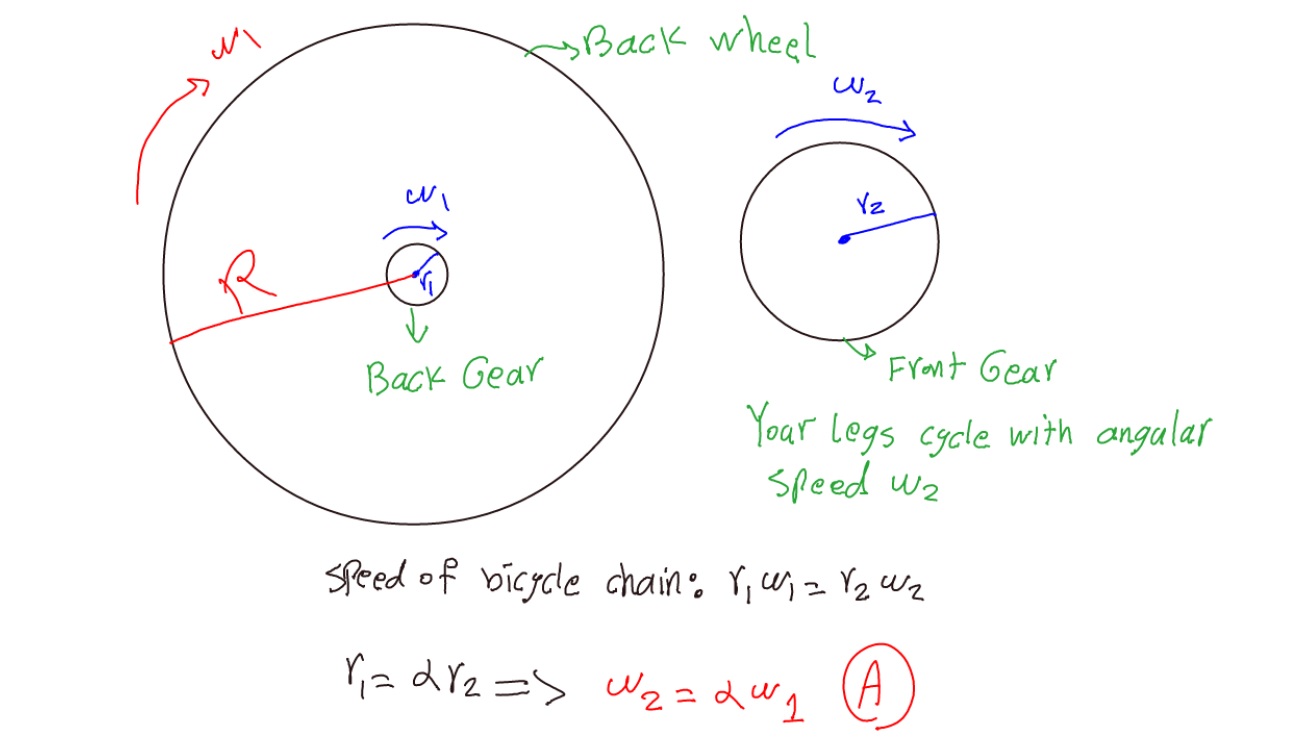
It has to do with how the torque is transmitted from the pedal gear and the wheel gear. The chain transmits the force equally, but the change in radius produces a change in torque.

So if the wheel and the pedal gears are similar in radius, the behavior is similar to riding a mono-cycle: all torque applied is transmitted unchanged.

The opposite case is when the wheel gear is smaller radius: the torque applied is reduced in the wheel.

Now, you may wonder: "Then what is the point of having gears that reduces the effect of the force applied?"

The thing is that both are linked by the chain, and this also links the rotational speeds of the gears, so if the gears were the same radius all the time, the faster you would go means you would have to rotate much faster, to increase your linear speed. This situation is much better when the radius of the wheel gear is smaller, since small angular rotation in the pedal gear, multiply and generate higher revolutions in the smaller one. Hence, you can make it go faster maintaining a normal pedal rotation for you.

Check out the image that illustrates $\tau_1 / r_1 = F_1 = F_2 = \tau_2 / r_2$. But always bare in mind that $\omega_1 = \omega_2$.

Improve this answer
$\endgroup$
-1
$\begingroup$

I think we should focus on hike of potential energy within us during climbing through a ramp. That`s why, when we climb either by cycle or walking, we feel it bit laborious. So, taking higher gear ratios, will increase the velocity definitely but it will take more effort making it MORE laborious than usual.

I am sharing a link of a video tutorial with numerical explainaion how gear ratios effects on speed:-
click here to watch it.
N.B.-Same thing(lower gears) must be maintained when driving a motor-vehicle uphill. Otherwise,the engine can be stalled or permanent damage can take place.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Hi Dwiparna Datta and welcome to Physics.SE! Whilst this may theoretically answer the question, it would be preferable to include the essential parts of the answer here, and provide the link for reference. $\endgroup$
    – Gonenc
    Commented Aug 4, 2015 at 9:46
-2
$\begingroup$

Here is my answer. I think it's self explanatory enough:

enter image description here enter image description here enter image description here

In the last sentence there must be a minor correction: If someone keeps omega1 constant, then for the same speed they have to cycle faster so they can maintain the same power. If someone keeps omega2 constant, then bigger alpha mean less forward speed but less power too.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Don't you bother to explain the down-vote at all! I wish it was obligatory to put a comment for down-vote. $\endgroup$
    – MOON
    Commented Feb 26, 2015 at 13:19
  • $\begingroup$ your equation A, doesn't seem to make sense. $\endgroup$
    – Black Dagger
    Commented Feb 27, 2015 at 8:00
  • 1
    $\begingroup$ @BlackDagger The linear speed of bicycle's chain is the same all over it and it is equal to the linear speed of its contacts points with the two gears otherwise it would slip on the gears. The linear speed of contact point on two gear must be the same. In equation A I just defined alpha as the ratio of those two radius. $\endgroup$
    – MOON
    Commented Feb 27, 2015 at 9:07
  • $\begingroup$ Why isn't it obligatory to leave a comment when some people down vote? This just disappoints me to contribute to this section of website. $\endgroup$
    – MOON
    Commented Mar 23, 2015 at 9:21
  • $\begingroup$ I downvoted due to the insertion of a picture referring your answer. You should really try to answer using LaTex. $\endgroup$
    – Autolatry
    Commented Aug 4, 2015 at 10:48
-4
$\begingroup$

Because of hp and torque. When in a low gear, the rpms are higher and the hp and torque kicks in at higher rpms.

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ You are not confined to few characters here, it is allowed to actually write out abbreviations and explain stuff instead of merely stating it. $\endgroup$
    – ACuriousMind ♦
    Commented Mar 22, 2015 at 23:51

Not the answer you're looking for? Browse other questions tagged or ask your own question.