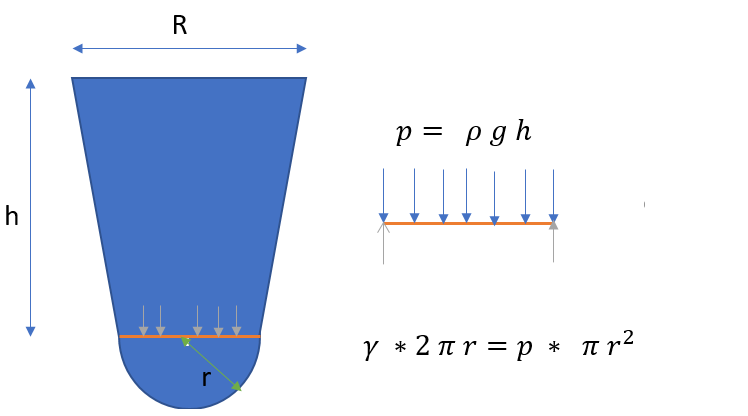
In a previous question, the following is answered in a general sense:
Assume I have a inverse cone which holds 200ml water. I am going to cut the tip of the cone to create a small hole. How to calculate the maximum radius of the hole that the water will still stay in the container ?
However, what is not clear to me is whether the material of the vessel matters. The surface tension of water IN AIR is used in the answer $\left(\gamma \approx 7.3{\times}{10}^{-2}\,\frac{\mathrm{N}}{\mathrm{m}}\right)$; however, I would think the surface tension and contact angle between the water and the cup have an effect.
For example, if I have a one cone cup that is hydrophobic and one cone cup that is hydrophilic, will the hole size necessary to stop the water dripping out be the exact same?