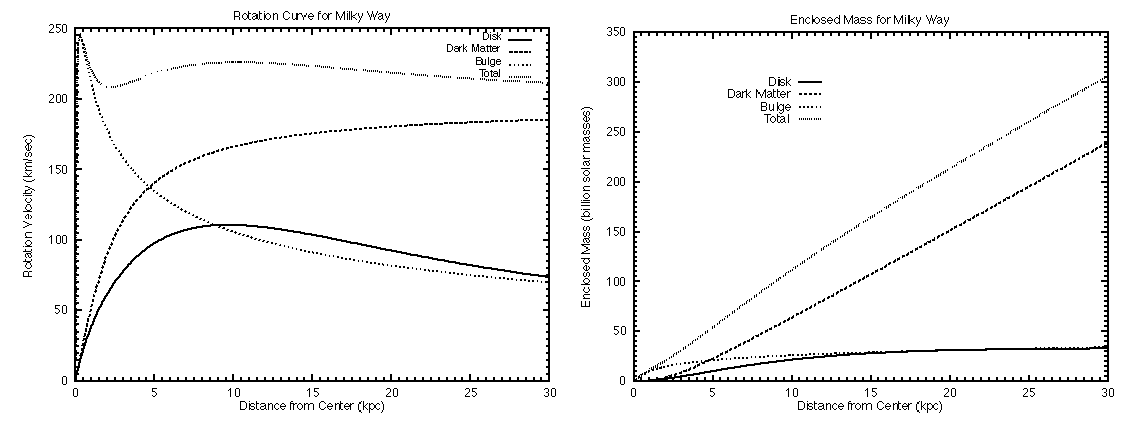
I am putting together a toy program which shows how stars move around in the galaxy.
To run the simulation I need to know strength of the Milky Way's gravitational field at any location in it. I'm looking for a model (e.g. a collection of uniformly dense planes/rods) rather than a database of potentials.
Where can I get such a model?
I could simply construct an infinite plane of uniform density, but is that good enough? This is only a toy so I'm looking for something which preserves integrity of the overall shape and statistics of the galaxy, rather than worrying about the specific location of any particular star.