As a first step, it would be good to review what this multipole moment $l$ means. The CMB is of course coming at us from a spherical shell around us. We therefore see a spherical projection, and we would like to quantify this much like we decompose a plane wave in trigonometric functions.
To do this on a sphere, we use spherical harmonics instead. These functions are the eigenfunctions of the angular part of the Laplace equation, and you might know them from quantum mechanics in which we use them to solve the three dimensional Schrodinger equation for e.g. the H-atom. They satisfy, for instance, the orthonormality requirement and can be used much like trigonometric functions in real space.
$l$ is a lable for the spherical harmonics, and can be compared to the wave number in plane waves: it is a measure (inversely proportional to) the size of perturbations. Like the wavenumber is inversely proportional to wavelength.
You are quite right that sound waves are involved: Just like sound travelling as density waves in air here on Earth, the photon-baryon fluid (which were coupled before decoupling) are influenced by such waves. Some of these with a particular wavelength (which, remember, we will see as an angular size on the sky) may be caught at a extremum, such that we see a lot of structure at these particular scales.
Whether a scale will be caught at a maximum or not, depends on its oscillation time until recombination. When atoms recombine, photons will not collide frequently anymore and these plane waves cease to exist.
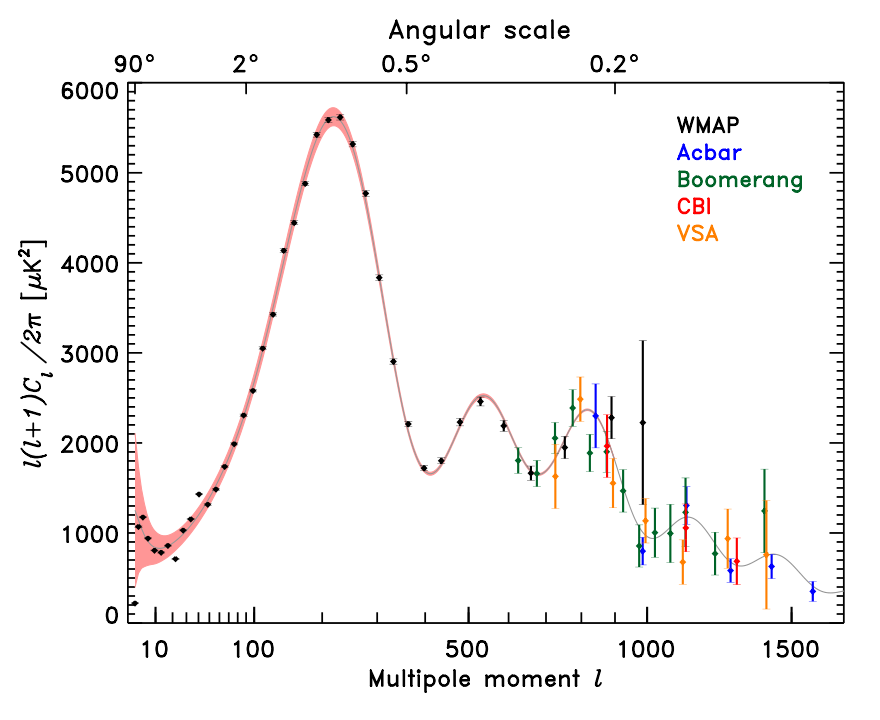
Now, to finally answer your question: how does the spatial curvature of the universe fit into this mix? The first peak corresponds to the largest scale that has been able to reach a maximum at the time of recombinations. This is related to the horizon size at the time: scales that have not entered the horizon have not started oscillating yet (because that is the meaning of the cosmic horizon: scales larger than this cannot 'communicate'.
Now, since we know fairly precisely how long after the big bang decoupling occured and so on, we can calculate fairly precisely how large the horizon should be at this time and this should correspond to a very well-defined angular scale in the sky, if the universe was Euclidian (spatially flat). However, if the space is in fact negatively (positively) curved, the projected size of the first peak (the horizon size at decoupling) would be smaller (bigger) than expected: a direct indication and measure of curvature effect!
You can compare this to a magnifying glass: if you observe a bug to be x-times as big as its (known) size, you can calculate the magnifying power of the glass -- in this analogy, the magnifying power is comparable to the spatial curvature of our universe.
Hope this helps!