1
$\begingroup$

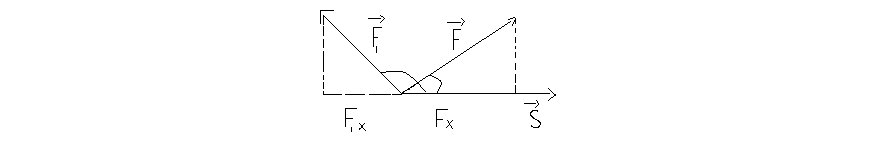
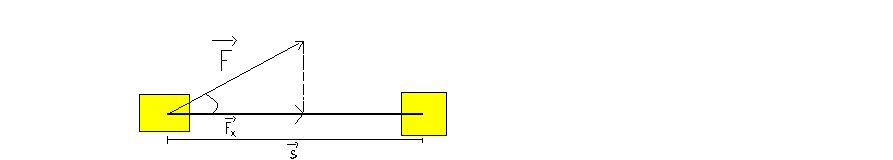
When we get angle > 0, the x-component of force is along the direction of displacement and so their product is called Work. So the x-component of force is said to have direction of the respective displacement? We see that when angle is obtuse between force and displacement vector the force vector is not itself opposite to the displacement vector but we get negative work. Is it due to the negative direction of the x-component of force then? Are the components of force also vectors? The second diagram is from my text-book.

enter image description here

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Angle, between $\vec{F}$ and $\vec{s}$, being greater than $\frac{\pi}{2}$ implies that the $x$-component of $\vec{F}$ is opposite the direction of $\vec{s}$, at least in the case you describe here. So yes. $\endgroup$
    – Samama Fahim
    Commented Dec 20, 2014 at 9:24

2 Answers 2

Reset to default
1
$\begingroup$

If $\theta$ is obtuse then $\cos\theta$ is negative and thus, $W=\vec{F} \cdot \vec{s}=Fs \cos\theta$ is negative.

Improve this answer
$\endgroup$
0
$\begingroup$

Yes.

Work $W$ is found as:

$$W=\vec{F} \cdot \vec{s}=Fs \cos(\theta)$$

The angle will from the dot product take care of the sign for you. A perpendicular force e.g. is not doing any work, since $\cos(90^\mathrm{o})=0$. When $\theta < 90^\mathrm{o}$, $\cos(\theta)>0$, and when $\theta > 90^\mathrm{o}$ you change sign, $\cos(\theta)<0$.

Remember that the angle is measured from the direction of the displacement.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.