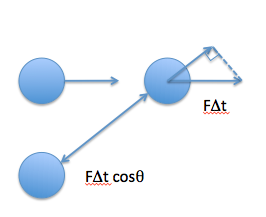
Let us consider a system of 2 identical spherical bodies connected by a massless string that is taut. If one body is placed at the origin then the other is placed at some coordinate $(x,y)$. The string that connects these bodies makes an angle $Z$ with the $x$ axis. Now consider another identical sphere that moves with some velocity $V$ parallel to $x$ axis such that it will eventually collide with the body placed at $(x,y)$. The collision is elastic.
Clearly in this case the string exerts some impulse that has an horizontal component. My questions are as follows:
- does impulse act on both the bodies connected by the string? Is it the same amount?
- Momentum cannot be conserved for the two colliding spheres as there is horizontal impulse?
- What is the goal of impulse? (like friction opposes motion of the body in contact always what does this impulse do?)