This electromagnetic wave diagram doesn't make sense to me

Question

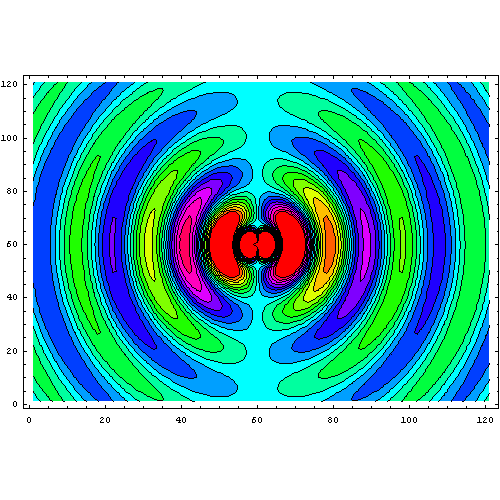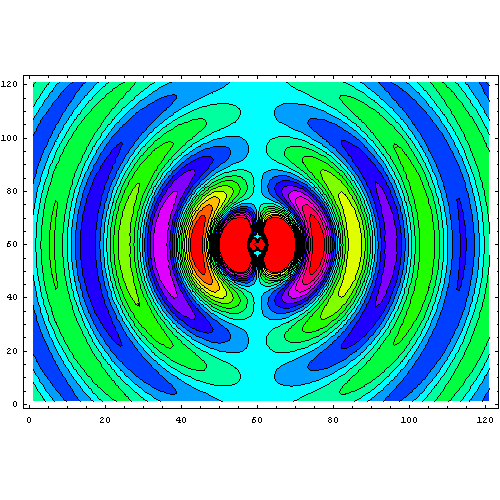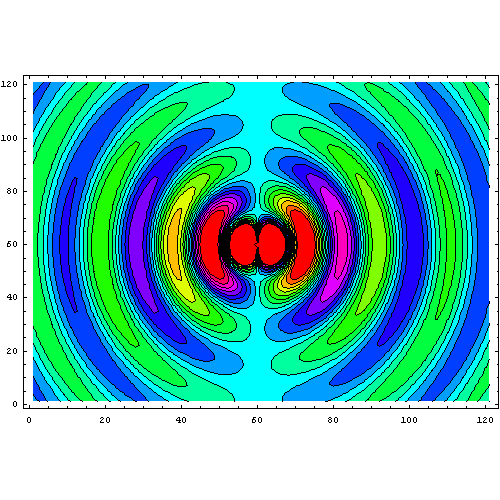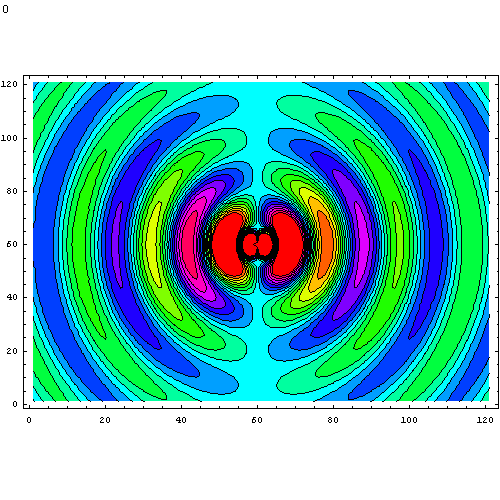
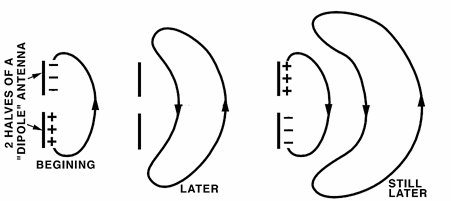
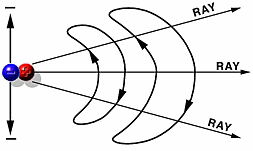
I am attempting to learn about electromagnetic waves. From my understanding, when two particles are a distance away from eachother, and one vibrates up and down, the stationary particle will experience a changing electric force that takes the form of a wave (in the sense that it goes up and down harmonically).

I've made the assumption that a stationary particle closer to the vibrating particle will experience a larger change in electric force than a particle that is further away. I think this because a closer particle will see a larger angle between the max and min of the vibrating particle's displacement. I've illustrated how I see it in the following picture:

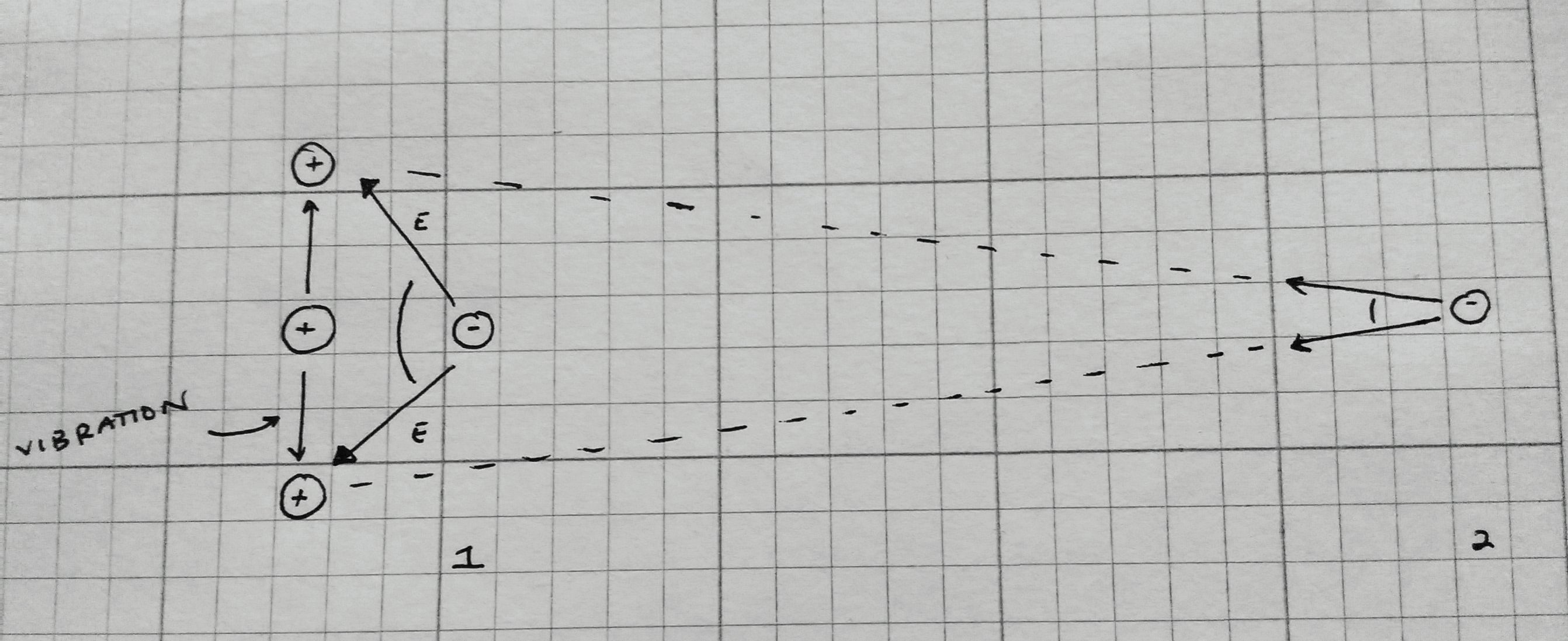
The positive charge vibrates up and down. Stationary particle 1, since it is close, experiences a dramatic change in the electric field, while particle 2 experiences a smaller change (The two electric force vectors ive drawn on each stationary particle represent the electric forces experienced when the positive charged has reached its max and min displacement).

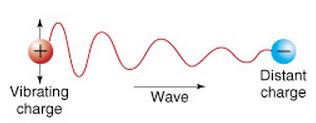
This all makes sense to me, until I saw the following diagram:

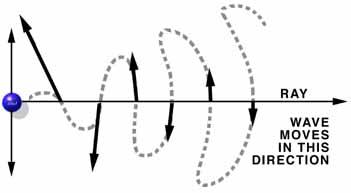
Assuming the wave represents the direction of the electric force experienced by the stationary particle as the positive charge vibrates, then it doesnt seem to make sense to me at all. Why would the "angle" increase the further away you are from the charge?

I later ran into this diagram, which agrees much more with my illustration:

Are the waves in these two diagrams representing different things? Is one of them wrong? Where am I mistaken?

Answer 1

I believe it is a good approach to use your intuition and real physics to guess how the effects should work. However, electromagnetism is the place where you start to need to understand the fields and other less tangible concepts.

All the pictures you show describe the situation from a point of view where your distance $d$ from the oscillating charge is much larger than the extent of the vibration $l$. I.e. $d \gg l$. I have found an applet which shows the field lines around an oscillating charge. Near the charge the radiation has quite messy directions.

However, once we are at $d\gg l$, the radiation gets less messy and we can understand the radiation as coming basically from a point. The result is basically dipole radiation with an overall electrostatic field. Why so? Dipole radiation comes from the oscillation of two opposite charges, an oscillating (+,-). However, if we were to put two static (+,+) over them then the result would be approximately an oscillating (2+,0). So if we put the field of a dipole and a static charge over each other, we get approximately the field of an oscillating single charge.

But we know exactly how the electrostatic field looks like, it always points to the center and falls of as $1/d^2$. Here is an image of magnetic field lines of dipole radiation:

The colors try to show both the sign and the magnitude of the field. Red means very strong field and cyan means weak field. Yellow and magenta/purple show the sign. The electric field looks similar - it is also tangent to the sphere of constant $d$ but is also perpendicular to the magnetic field.

Now let us add the electrostatic field which always points inwards and falls of as $1/d^2$ to the dipole radiation. The dipole radiation never points inwards, it is always tangent to the sphere of constant $d$. Furthermore, the dipole radiation falls of as $1/d$. So for large $d$, the term $1/d^2$ will be very small compared to the $1/d$ dipole term. Thus for large $d$, the dipole radiation dominates and the electric field is basically tangent to the sphere of constant $d$. On the other hand, for $l \ll d \ll 1$ the $1/d^2$ term will be much stronger and the field will tend to point much more inwards due to electrostatic forces. This is exactly what your second diagram shows.

So the effect in the second diagram is all a consequence of including the electrostatic attraction which is still there, but for large directions it becomes negligible compared to the electromagnetic wave.

Answer 2

Unfortunately I have not met the reputation threshold to post comments, so I'm resorting to providing an answer.

I would assume this would have to do with a common misconception you may have of an electron being a billiard-ball-like spherical ball of matter traveling along a wave. This is not quite accurate, though you may very well already be aware of this.

Saying that the wave motion of that image is accurate, but saying that the electron follows that path is not. Rather it is showing the electric field band that extends infinitely. Here is a much better image more accurately depicting what is fundamentally going on.

Answer 3

Radiation from an antenna occurs because of the fact the medium of transmission, including space and vacuum have permeability and permittivity which are related to the inductive and capacitive effects and so they can store both electrical and magnetic energy. As an analogy, one can take the medium to be a rope with mass and at one end, a man lifts the rope slowly and the rope close to him is elevated and the mass particles in the rope attain potential energy due to the height raised. Then if the man SUDDENLY" ACCELERATES his hand down faster than the mass inertia of the rope his hand will accelerate down faster than the mass of the rope which was elevated at some distance and so a wave will proceed to move along the line. With a dipole, both ends will excite the close environment with an electric field and so the capacitive effect of the close medium will charge up with a VOLTAGE then if the two dipole ends start reversing their polarity, the close previously charged capacitors cannot return the charge to the antenna as the antenna accelerated its two ends faster than the stored energy in the medium could do. Similar parallel polarities of the E field will push each other away and while the antenna will proceed with its ACELLERATIONS the distant E field will try to collapse causing a build-up of the MAGNETIC field to try and hold it, but eventually, all the energy previously stored in the launched E field will be transferred to the Magnetic field till the whole E field collapses and then when the magnetic field will start collapsing the electric field will build up in the opposite direction field will build. The rate of change of one field will decide the magnitude of the other. Unfortunately, there is an EM diagram that has been going around in books and publications for a long time and I believe it is wrong in its depiction of the E/M wave as it suggests that the Poynting power vector oscillates> Many people misinterpret the rate of change of a rotating vector with the rate of change of a pulsating polarised vector. Their mistake is that they show a translated polarised E/M diagram and they ROTATE IT which is absolutely wrong, In a polarised pulsating field the rate of change is not in the rotation but in the translation and pulsating of the polarised magnitudes.