I have already seen this question but I am not very familiar with the group theoretical framework of the Standard Model and was hoping for a more diluted answer.
So I have come across this slide:
which says that the different phases in the CKM matrix for quarks and antiquarks results in CP violation...
First off, why? how?
Secondly, does it explain CP violation fully, or is the experimentally measured CP violation greater/lower than this?
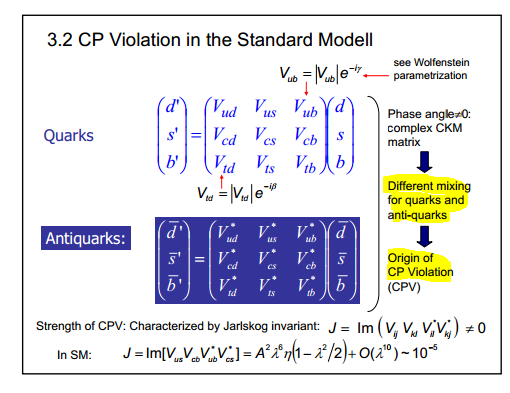
This is a very tricky topic and I can only expose a very simplified argument but that will hopefully enlighten you enough.
The important point to understand is that, when dealing with an actual process, the fact that the electroweak interaction violates CP is typically not enough to give an observable effect. Let me try to explain. Consider the decay $M\to f$ where $M$ is a particle such as a Kaon, a $D$ meson, etc and where $f$ may collectively denote several particles. We then consider its $CP$ conjugate $\bar{M}\to\bar{f}$. So the bar means that we have taken the anti-particles and reversed momenta. We can then write the amplitude for these two processes: $\bar{A}_\bar{f}$ for $\bar{M}\to\bar{f}$ and $A_f$ for $M\to f$. Those are just notations (they are those used in the Particle Data Group review iirc): the bar over $A$ does not mean to suggest a priori a complex conjugation, even though as we will see this may just be the case. We measure only the modulus square of amplitudes, so the measurement of a CP violation boils down to find that $|\bar{A}_\bar{f}|\ne|A_f|$. Experimentally, the following ratio is preferred:
$$\mathcal{A}_f=\frac{|\bar{A}_\bar{f}|^2 - |A_f|^2}{|\bar{A}_\bar{f}|^2 + |A_f|^2},$$
which is then to be compared to 0.
Typically, the amplitudes will be the sum of several contributions, each potentially with its own behaviour under $CP$. Let's consider only 2 components to keep it simple:
$$A_f = A_{f,1} + A_{f,2}.$$
Now comes the essential point: if the phases of $A_{f,1}$ and $A_{f,2}$ come only from the CKM matrix, then the CP conjugate process will result in the complex conjugate, for the very reason highlighted on the slide you quoted.
$$\bar{A}_\bar{f} = A_{f,1}^* + A_{f,2}^*.$$
Clearly, $|\bar{A}_\bar{f}| = |A_f|$ and no CP violation is observed! How come we have observed it then, in the decays of Kaons, $D$ mesons and $B$ mesons? That is because the phase of $A_{f,1}$ and $A_{f,2}$ does not come only from the CKM matrix. There are other contributions coming from QCD. So actually, we have
$$A_f = |A_{f,1}|e^{i(\phi_1+\delta_1)}+|A_{f,2}|e^{i(\phi_2+\delta_2)}$$
where the $\phi$'s are still the phases coming from the CKM matrix (they are therefore called weak phases) but we have now the $\delta$'s coming from QCD (they are therefore called the strong phases). Since QCD is CP invariant, we have now
$$\bar{A}_\bar{f} = |A_{f,1}|e^{i(-\phi_1+\delta_1)}+|A_{f,2}|e^{i(-\phi_2+\delta_2)}.$$
We have taken the complex conjugate of the part with the weak phases but left the part with the strong phases intact. A bit of tedium will then give you that
$$\mathcal{A}_f \propto\sin(\delta_2-\delta_1)\sin(\phi_2-\phi_1).$$
So to observe $CP$ violation we need that both $\delta_2 \ne \delta_1$ and $\phi_2\ne\phi_1$. The former should not come as a surprise since the $\delta$'s are equal, we can factorise the same $e^{i\delta}$ in both $A_f$ and $\bar{A}_\bar{f}$, and it therefore disappear when taking the modulus, leaving the situation exactly as if there were no strong phases, and we have seen that there is no CP violation to be observed then.
Physically this means that we need
The problem, however, is that the difference of strong phases $\delta_2-\delta_1$ include non-perturbative hadronic contributions which are from hard to impossible to compute. There are cases, though, where they can be deduced from other processes and then transferred in this analysis.
I must finish with a caveat: I have oversimplified and lied by omission by brushing under the carpet some important aspects so that my answer could be reasonably self-contained and pedagogical (or so I hope!). That gives you the gist of it at least but you will need to learn way more if you want to actually work on the subject.
different phases in the CKM matrix for quarks and antiquarks results in CP violation... First off, why? how?
This is because if the mixing matrix for the quark fields is $V$, then that for the antiquarks is $V^*$.
Secondly, does it explain CP violation fully, or is the experimentally measured CP violation greater/lower than this?
The general parametrization of CKM matrix doesn't predict any value of $\delta$. The CP-violation has to be measured experimentally. To predict it theoretically, you must have some model for the quark mass matrix.
In the standard model of electroweak interactions, the CP violation appears as a complex phase in the CKm matrix. Such a complex phase can be ignited by breakdown of SN permutation symmetries as demonstarted in our recent reasearches arXiv:1403.3482 [hep-ph], 1902.08749 and 2010.08245.
