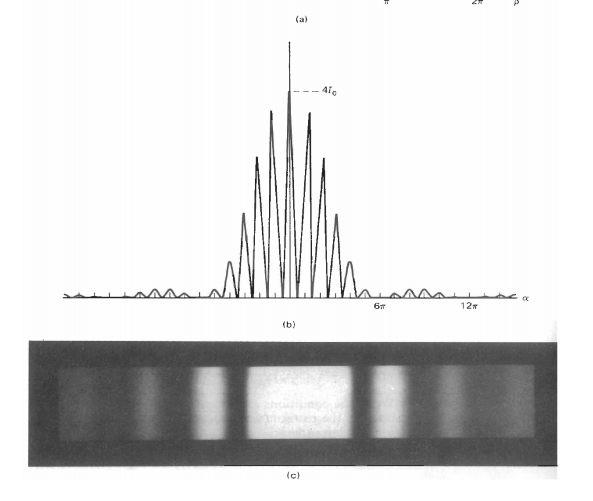
I was taught in class that the double slit diffraction pattern would always have bright fringes of same length. We derived the formula: $y=m(\lambda)(L)/d$, which also shows this equal distance. I look at these pictures now, and I see a central maximum with almost twice the largeness of other fringes. What is going on?
I think maybe it's just a bad photo. The double slit diffraction pattern is a convolution of the pattern from a pair of delta functions with that of a single slit. i.e. You have the regularly spaced double slit pattern, but this is modulated by the pattern produced by a single slit of width equal to that of the slits used for your double slit experiment. In the photo you show it looks like maybe poor resolution/focus/contrast has lost the distinction of the finer double slit fringes?
The photo below from the wikipedia page on "Introduction to quantum mechanics" shows this more clearly.
Or maybe, as others have said, it is just a photo of a single slit diffraction pattern put there in error
You are right. In the interference pattern produced by two narrow slits (e.g young's double slit experiment), the width of all the fringes (bright, dark and central) is same. The picture you have added is not of double slit experiment, it is of single slit experiment.
The first picture looks like a theoretical curve of whatever, the photo is from a single-slit experiment.
Have a look on this photo, where you can see that a double-slit has fringes with swelling intensity and dimensions.
If you go back to start from the easiest case you have to look on a edge. Behind an edge you see half the picture from your photo. Now take a seconds edge and start to move them closer together. At the end you get the "full" photo with decreasing intensities and different distances of the fringes to each other.
Depending on the wavelength, the color of the light and the distance from a double-slit plate to the observer screen you get different fringes on the screen (see my link below). At the end of the fringes pattern (on the left and on the right) you will see the typical fringes pattern of an edge (for monochromatic light from a point source).
Of course it is possible to arrange the slits in interaction with a light source as well (then more for a multi-slit), as you can see on the screen a homogenous pattern of fringes. But how artfully you do it, you ever will see on the left and on the right end of the patterns fringes with decreasing intensity and dimensions.
I'll catch the chance to expand my answer. Huygens priciple tells how a water wave expandes to a spherical wave into the space behind an edge. This wave fast goes to zero because the energy of the wave in the pointlike area at the edge expands in a bigger and bigger volume. The wave disappears. And if you imagine a line in the air above the wave you see that the maxima and the minima are moving. Take this in mind when we talk about fringes from light bended on an edge.
What happens with the light at an edge? It gets divided into some areas with continuos change from high intensity to zero and again to some intensity and at the end it disappear. This intensities don't move like the water waves. The light in the fringes does not disappear at some distance in the traveling direction. And this is OK because there is no medium for dissipation (if one do the experiment in vacuum of course).
So what is the difference between water waves and diffraction of light at an edge? Light is a lot of photons, and if you take monochromatic light, a lot of same energy photons and they interact with the electric field of the electrons of the edge. The last point one has to imagine is that the field they "built" together is quatitized. The fringes on the observers screen show how the field is quantitized.
