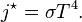From a related question (How does pressurized gas constantly push?), i asked myself this: How do gas molecules constantly bounce off each other without losing energy?
If you drop a ball, it bounces a bunch of times, but the height of each bounce gets shorter and shorter. Because it loses energy in each bounce.
So I don't see how a pressurized gas doesn't lose energy. As I understand it, pressure is a manifestation of lots of molecules bouncing off each other. So over time, shouldn't the pressure drop as the molecules lose speed? and eventually all the molecules will settle in a pile on the floor.
I think another way to phrase this is, how do elastic collisions not lose any energy in the exchange? My understanding of the 2nd law of thermodynamics is that some energy is always "lost" when it's converted from one form to another, or transferred from one object to another. I.e., no transfer/conversion of energy is ever 100% efficient.