Consider the following figure:
The surface is not friction-less. When the force $F$ is applied on mass A: Is the force the mass A applies on mass B larger, smaller or equal then the Force that B exerts on A?
When F vanishes, what is the force that A exerts on B?
I think that the answers are: 1. equal to-. 2. Don't know, It's quite confusing.
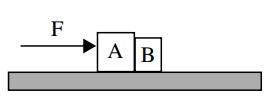
Yes, the force $A$ exerts on $B$ has to be equal to the one $B$ exerts on $A$. And when $F$ vanishes the forces between $A$ and $B$ vanishes too.
More specifically, if in the time $F$ is acting no movement occurs (i.e. $F$ does not overcome the total friction of $A$ and $B$) the only thing causing $A$ and $B$ to interact is $F$, so this action reaction forces between them has to vanish when $F$ disappears. Now, the balance of forces on $A$ tells us that $F$ opposes to the friction on $A$ and the reaction of $B$ on $A$, so the higher the friction on $A$, the smaller the reaction which in $B$ is balanced by its friction with the floor, and in this case the frictions seem different due to the difference in masses, I think this is what causes your confusion.
But if there was movement, while $F$ is acting the balance in forces is the same as mentioned for the static case, and both $A$ and $B$ are being accelerated with different accelerations (since the accelerating forces are different: on $A$ is the resulting of $F$, $F_r^A$ and $F_{BA}$, while on $B$ is the resulting from $F_{AB}$ and $F_r^B$). But their velocities are the same in every moment, so when $F$ disappears they are static in a reference frame moving along with them, and the vanishing of their action-reaction is compatible with the static case.
