Conservation of momentum but not kinetic energy in inelastic collisions

Question

In inelastic collisions, the kinetic energy of the system is not conserved but the momentum is.

Kinetic energy is: $0.5 \times \text{mass} \times \text{velocity}^2$. Momentum is: $\text{mass}\times\text{velocity}$.

I think that considering that mass is constant:

• if Ke must be different also the velocity of the centre of mass of the system must be different, after the collision. On the other hand:

• if the momentum of the system is conserved, the velocity of the centre of mass of the system cannot be different.

So, how can there be a change in kinetic energy of the system if there is no change in momentum? $mv = m_1v_1$

Answer 1

A simple counterexample:

Imagine two particles with opposite direction and equal speed. The center of mass does not move, yet the kinetic energy of the system is non-zero.

Now let both particles come to rest (by friction, hitting a wall, whatever). The kinetic energy is now zero, and total momentum has been conserved, while energy is not.

The crucial point is that kinetic energy depends on the square of velocity, $E_\mathrm{kin} = \frac{1}{2}mv^2$, and so is always positive - it cannot "cancel out" as momentum does, so momentum $\vec p = m \vec v$ can perfectly be conserved while the kinetic energy changes if the terms with "positive" and "negative" sign decrease or increase in a fashion that keeps the total momentum constant.

Answer 2

if mass is assumed to be constant, the velocity of the centre of mass of the system has to be different after the collision for the kinetic energy to be different.

However, if the momentum of the system is conserved, the velocity of the centre of mass of the system should remain the same.

1) mass is not constant and velocity is different: in a completely inelastic collision the two objects (A: m =1, B m = 2) stick together and mass becomes A+B = M = 3

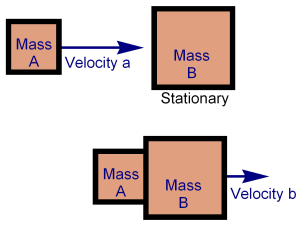
Suppose that $v_a = 6m/s$ and $v_b = 0 \rightarrow E_k = 0.5 * 6^2 = 18, p_a = 1 * 6 = 6, v_{cm} = p/M = 2$

After collision velocity would be anyway lower as KE should be distributed among more mass, but some KE is lost in the crash. How much?

Momentum is conserved: $ p_{ab} = 6$ , from this datum you can calculate its velocity: $$v_{ab} = v_{cm}= \frac{6}{3} = 2$$ and $E_k = 0.5 * 2^2 *3 = 6 \rightarrow E_a = 2 + E_b = 4$.

Some energy has been transferred to B, but two thirds of the energy have been lost.

Velocity of center of mass is the same, although KE has changed.

Please note that momentum is conserved because we are assuming that on the surface of contact there is no friction.

...how can there be a change in kinetic energy of the system if there is no change in momentum?

A change of KE without a change of momentum is not only possible but very frequent, because as you noted p = mv momentum varies linearly and KE quadratically. You can get the same product by a wide range of factors: 6 = 6*1, = 3*2, = 2*3, = 1*6, = 0.5*12, etc., different factors give same momentum

All these factors give same values for m*v, but as the figure for v must be squared, you get all different values between momentum and energy, therefore the same factors give momentum = 6, but KE =3, =6, =9, =18, =72, etc, same momentum corresponds to many different values of KE

I hope this clarified all your doubts

Answer 3

... if the momentum of the system is conserved, the velocity of the centre of mass of the system should remain the same.

True.

... the velocity of the centre of mass of the system has to be different after the collision for the kinetic energy to be different.

False.

So, how can there be a change in kinetic energy of the system if there is no change in momentum?

Each particle changes momentum during the collision - let's examine how that works.

Before the collision, break the velocity of each particle in two parts (with vector subtraction) - one that travels with centre mass and one that travels towards centre mass. After the collision, the centre mass continues, but the inward momentum (and its contribution to kinetic energy) are gone. Does that make sense?

Answer 4

In an inelastic collision, some of the energy is absorbed by the colliding bodies - this is why you cannot use conservation of energy to calculate the resultant velocities of the bodies involved - you don't know how much is absorbed. But you do know that momentum is conserved, and assuming that the bodies remain intact (no pieces are separated from the body), then you can just use MV.

Answer 5

You are making a basic conceptual error! You considered the center of mass (COM) of the system to be a single particle and therefore you thought that the kinetic energy (KE) of COM: $$KE_{COM} = \frac{m_{tot} v_{COM}^{2}}{2}$$ But KE of COM is not that. It is rather: $$ KE_{COM} = \frac{m_{1} v_{1}^{2}}{2} + \frac{m_{2} v_{2}^{2}}{2} + \frac{m_{3} v_{3}^{2}}{2} +...$$ You only know that: $$m_{tot} v_{COM} = m_{1} v_{1} + m_{2} v_{2} + m_{3} v_{3}...$$ So in a perfectly inelastic collision due to lack of any external force on system the momentum of COM remains conserved and the velocity of COM remains constant but the KE differs as some portion of the KE is transformed into elastic potential energy.