Do electrons flow only on the surface of a wire? [duplicate]

Question

Since the $\vec{E}$ field inside a "perfect" conductor is zero, do the electrons(the current) flow only on the outer surface? This has bothered me since I studied electromagnetism.

Thank you for your time.

Answer 1

A simplified picture for DC circuits is as follows: A charge develops at the surface of the wire, having a gradient along the length of the wire. This surface charge distribution causes an electric field within the wire, pointing along the wire, and having a uniform cross section. This field accelerates the charge carriers in the bulk of the wire. The current is uniform in the bulk, not restricted to the surface.

However, it's not that simple. There might be fields due to external sources, such as the battery that energizes the circuit. A more detailed analysis shows that the surface charge distribution that develops is not uniform. It adjusts in such a way that the net field, that is the field due to the surface charge plus all external fields, is uniform within the wire. The resulting current density is uniform in cross section.

It's important to realize that this system is not in equilibrium. Charges are being added at one end of the wire and removed at the other. Fields can exist in a non-equilibrium conductor.

Bruce Sherwood, co-author of the textbook Matter and Interactions has created a couple of simulations showing these things. The first shows how a uniform surface charge gradient creates a uniform electric field. The second shows electric fields in various situations involving conductors and wires. The first two, "Polarized Block" and "Simple circuit", are particularly instructive. Make selections using the buttons on the bottom. The resulting arrows represent different electric fields.

In "Simple Circuit", if you select the field due to surface charge only, you will see that the field due only to the surface charge is not uniform. But if you select net field, you will see that the net field is uniform.

You'll have to play with the second one for a while to understand what it's showing you. I think it's worth the effort.

Your web browser must support WebGL to see these ... try Firefox. Chrome works for me sometimes.

Answer 2

I'm leaving an answer because the following intuition needs some proper debunking:

Since the E field inside a "perfect" conductor is zero, do the electrons(the current) flow only on the outer surface?

The logic is perfectly clear, and applied totally incorrectly in this case. I think most people would start with this approach, but more careful consideration shows that it's irrelevant.

The conclusion that charge is located entirely on the surface of a conducting wire arises when using the assumptions that:

1. The wire has a net charge
2. There is a fully steady state situation

Both of these are completely misplaced assumptions regarding a current-carrying wire. The wire may or may not have a net charge. Even if it did, it could be negative or positive. No matter what you assume, it should be clear that the charge has no impact on the ability to conduct charge and thus the effective resistance.

Why should this be true? Because current pushes electrons through which are in the conduction band. Unless you're using a truly insane voltage level, the charge does not significantly affect the total density of charge. The electric potential buildup is due to a marginal change in the balance of charge, which is tiny compared to the total population of conduction band electrons.

To be be painfully explicit about this, let us reflect that the Debye length quantifies the distance from the surface where you have notable charge buildup. This is determined by balancing the charge buildup effects with the electric field. This length is small, and it has nothing to do with current or AC frequency or anything like that. It is just the distance over which the surplus surface charge is smeared.

Here is an illustration of the Debye length:

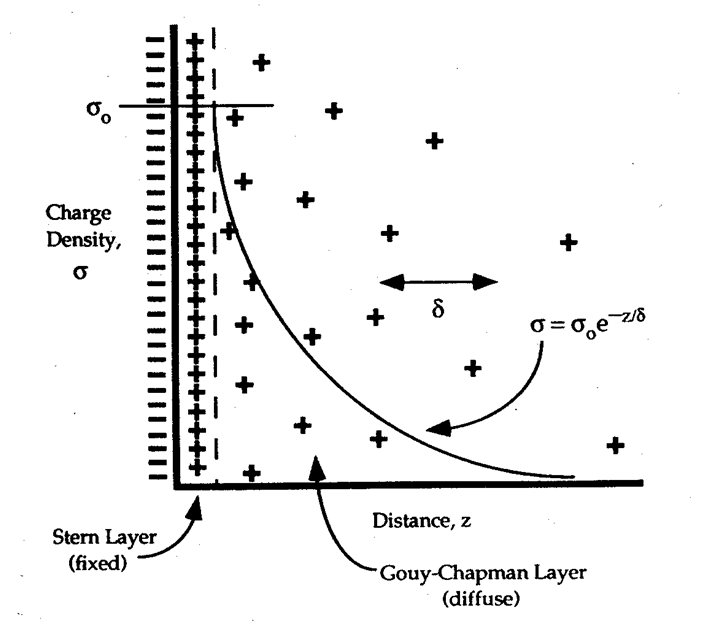
Compare to the skin effect, where the current is forced to flow in the regions of the wire closer to the surface. This is caused by a combination of eddy currents, an induced magnetic field, and the effect on the flow distribution by the changes in that electric field.

Here is an illustration of the skin effect:

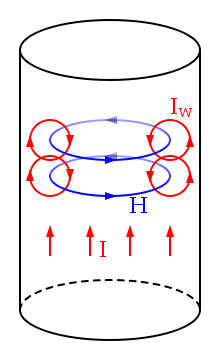
These are two very different things. If you get nothing else from my answer, you can still look at the two different diagrams and say "those use different physical concepts". The first one uses electrostatics and conduction band quantum effects. The second one uses eddy currents and changing magnetic fields.

It is an interesting and deceptive coincidence. So this is a good question. Nevertheless, it's a true coincidence.

Answer 3

No, current is supposed to flow through all parts of the conductor, although not necessarily with equal current density. If the conductor is perfect, the electric field vanishes but there may be non-zero current density inside. Surface component of current can be probably neglected for situations with stationary current.

Answer 4

This article explains how electrons flows in a wire. Basically, the penetration depth of the electrons in a conductor depends on the frequency of the varying electric field to which they are subjected.
For DC, electrons flow into the wire; as the frequency increases, the flow starts to move toward the surface.

Since the E⃗ field inside a "perfect" conductor is zero,

This is true for an isolated conductor.

Answer 5

Note that in a perfect conductor (zero resistance) nowhere can a static electric field be sustained. For real conductors, DC current flows evenly over the cross-section. But AC current flows mainly near the surface (the so-called skin effect). AC current can exist only on the surface of a perfect conductor.

Answer 6

A steady current cannot flow in a perfect conductor. In deriving Ohm's law to describe currents in conductors, we must assume that the conductor is "good" but has some resistivity. The electric field inside the conductor is not zero, but the force it produces on the flowing electrons is counteracted by the resistive drag force from the conductor. When a steady current has been established, the drag force is just big enough to cancel the force from the applied field. That's how you derive V = IR. (Note that if the conductor were perfect, R would be zero, so any applied voltage would produce an infinite current.)

In the case of superconductors, where the resistivity really is zero, you are right - any current must flow on the surface. In fact, if the superconductor is immersed in a magnetic field, just enough surface current will flow to cancel out the field so there will be no magnetic field inside the material either.