Has gravity ever been experimentally measured between two atoms?

Question

Has there been any experiments, or are there any references, demonstrating gravity between atoms? If so, what are the key experiments/papers? Or if not, what is the smallest thing that has actually experimentally been shown to be affected by gravity?

I don't know of specific papers demonstrating gravity between larger objects, but I can vaguely remember learning about them in my classical physics class as an undergraduate. However, I have never heard of experiments demonstrating gravity at atomic or subatomic levels.

I don't have a physics background so it's not obvious to me, so just looking to see the actual research/evidence behind it, so I can start to try to imagine how gravity works at a quantum level.

Answer 1

Groups in Seattle, Colorado, and perhaps others managed to measure and verify Newton's inverse-square law at submillimeter distances comparable to 0.1 millimeters, see e.g.

Sub-millimeter tests of the gravitational inverse-square law: A search for "large" extra dimensions

Motivated by higher-dimensional theories that predict new effects, we tested the gravitational $\frac{1}{r^{2}}$ law at separations ranging down to 218 micrometers using a 10-fold symmetric torsion pendulum and a rotating 10-fold symmetric attractor. We improved previous short-range constraints by up to a factor of 1000 and find no deviations from Newtonian physics.

This is a 14 years old paper (with 600+ citations) and I think that these experiments were very hot at that time because the warped- and large-dimensions models in particle physics that may predict violations of Newton's law had been proposed in the preceding two years.

But I believe that there's been some extra progress in the field. At that time, the very fine measurement up to 200 microns etc. allowed them to deduce something about the law of gravity up to 10 microns. These are extremely clever, fine mechanical experiments with torsion pendulums, rotating attractors, and resonances. The force they are able to see is really tiny.

To see the gravitational force of a single atom is obviously too much to ask (so far?) – the objects whose gravity is seen in the existing experiments contain billions or trillions of atoms. Note that the (attractive) gravitational force between two electrons is about $10^{45}$ times weaker than the (repulsive) electrostatic one!

Most of the research in quantum gravity has nothing whatever to do with proposals to modify Newton's laws at these distance scales. Indeed, gravity is the weakest force and it's so weak that for all routinely observable phenomena involving atoms, it can be safely neglected. The research in quantum gravity is dealing with much more extreme phenomena – like the evaporation of tiny black holes – that can't be seen in the lab.

Plots and links to new papers available over here (thanks, alemi)

Answer 2

Measure the gravitational attraction between two atoms? Heavens no. That's such a tiny, tiny attraction. The atoms will be attracted to themselves gravitationally, but only minutely. They'll be attracted gravitationally much more strongly to the Earth, to the lab setup and measuring equipment, to the buildings around the measuring equipment, and even to the snow on roof of the buildings.

What can be measured is the gravitational attraction between atoms and the Earth. Some atomic clocks depend on the fact that atoms are subject to gravity. Atomic fountain clocks such as NIST-F1 use lasers to juggle a stream of atoms of cesium. Lasers cool incoming atoms to near absolute zero and then toss the atoms up into a microwave resonant cavity. The atoms shortly fall back down. Lasers are used on these falling atoms determine if they have switched state. An atomic fountain wouldn't work if atoms weren't subject to gravity.

An important question that keeps popping up (pun intended) is "Does antimatter fall up?" Asking this question goes very much against the grain of the equivalence principle, so it's a bit of a fringe question. The ALPHA Collaboration nonetheless worked toward answering this question by attempting to measure the gravitational mass of trapped antihydrogen. The results so far haven't been all that definitive; they found the gravitational mass of antihydrogen to be somewhere between -65 times and +110 times the gravitational mass of hydrogen. A lot more work needs to be done to confirm or invalidate the equivalence principle using antihydrogen.

You can read the full article on this experiment at Charman, A. E., & ALPHA Collaboration. (2013). Description and first application of a new technique to measure the gravitational mass of antihydrogen. Nature Communications 4:1785.

Update, nine years after the fact, hat tip to @DhruvSaxena

And now physicists do know that antimatter does not fall up: Anderson, E.K., Baker, C.J., Bertsche, W. et al. Observation of the effect of gravity on the motion of antimatter. Nature 621, 716–722 (2023).

Answer 3

(Skip to the bottom for a list of classical and quantum-mechanical effects of gravitation that have been observed in subatomic particles; my attempt to explain quantitatively what it would take to measure atom-atom gravity got longer than I'd intended, and I haven't had time to shorten it yet.)

Let's suppose you want to measure the gravitational attraction between two charged particles, with masses $m_1,m_2$ and charges $q_1e,q_2e$. The classical potential energy between the two particles is $$ U = -\frac{Gm_1m_2 + \alpha\hbar c\, q_1 q_2 }{r} $$ with gravitational constant $G$ and inter-particle distance $r$; the dimensionless fine-structure constant $\alpha\approx 1/137$ is defined by the relation $\alpha\hbar c = e^2/4\pi\epsilon_0$. The remarkable thing about this system is the weakness of the gravitational force: the Particle Data Group tabulates $G/\hbar c \approx 6.7\times10^{-39} (\mathrm{GeV}/c^2)^{-2} $, so for electric and gravitational interactions to take place at the same scale between similar particles, they'd need to have a mass-to-charge ratio of $m/q \approx \sqrt{\alpha \hbar c/G} \approx 10^{18}\,\mathrm{GeV}/c^2$. A proton has a mass-to-charge ratio of $0.94\,\mathrm{GeV}/c^2$, and a heavy nucleus might have $m/q \approx 200\text{–}240\,\mathrm{GeV}/c^2$ — a completely different ballgame.

In the land of electroweak interactions, we also have an interesting but feeble force which we might like to study against the overwhelming background of the electromagnetic and strong interactions. There we have the advantage that electroweak interactions strongly violate a symmetry, parity, which the electromagnetic and strong interactions do not. There's a whole class of experiments which put a polarized beam on a target and rapidly flip the spin of the particles in the beam, looking for a parity-violating asymmetry in the interaction of the beam with the target. The state of the art for asymmetry experiments is part-per-billion sensitivity. It's as if I gave you an "unfair" coin which, if you flipped it a billion times, would give you one more tails that heads. There's a fundamental limit to these sorts of experiments, known as counting statistics: if you are expecting $N$ identical-but-uncorrelated things to happen in a particular time interval, you typically get $N\pm\sqrt N$. In order to measure an asymmetry of 10^–9, you're screwed by counting statistics unless you have at least 10¹⁸ events to compare; if you want "three-sigma significance" then you need another factor of $3^2=10$ more. Remember that a mole — a gram of neutrons, or two grams of molecular hydrogen, or 27 grams of aluminum, and so on up the periodic table — only contains 10²⁴ atoms. Confidently counting 10¹⁹ atomic interactions is no mean feat. It's doable, but typically takes about a decade of design work, a couple of years of data collection, and a couple of years of analysis.

This approach doesn't scale to gravitational interactions between charged atoms, for two reasons. The first is that the counting statistics is basically impossible, almost literally the square of the state of the art. If you wanted to look for a gravitational asymmetry in the scattering of lead ions, with $m/q=210$, you'd expect an asymmetry around $2\times10^{-16}$, and so you'd need somewhere around 10³² interactions — imagine thousands of tons of lead, examined one atom at a time. The second is that for an electro-gravitational asymmetry, the sign change is on the wrong term: rather than looking for a minute difference between two very similar events, you'd have to look for the same minute correction to like-charge and opposite-charge interactions. It's unlikely you could measure the two interactions with enough precision to be compared to each other. For instance, the rest mass of a Pb⁺ and Pb^– ion are different by five parts per million, just because the one has two fewer electrons than the other.

Looking for gravitational interactions between neutral atoms would be easier, but not millions of times easier. Neutral atoms may still have magnetic moments, and can electrically polarize each other at close approach; these effects are well-described, but not described at the part-per-million level. Plus, neutral atoms are harder to push around than ions. Any real atom-atom gravitational experiment would have to go through many orders of magnitude of currently-unexplored effects of residual electromagnetism before gravity became measurable.

What you can do is to measure the gravitational attraction between one subatomic particle and the rest of the Earth, the same way that my bathroom scale measures the attraction between my belly and the rest of the earth. There are only a handful of successful experiments in what I think of as "semi-quantum gravity," showing quantum-mechanical effects in a Newtonian gravitational potential:

• David Hammen's answer mentions the cesium fountain clock, in which a cloud of atoms is permitted to rise and fall under the influence of gravity, but that's essentially a classical effect. The cesium atoms rise and fall just like juggler's balls.

• Similarly, I consider the Pound-Rebka experiment a classical effect. While the detection process in that experiment was scattering gamma photons from iron nuclei, the gravitational effect is a frequency shift which is also described by classical electromagnetism combined with general relativity.

• The neutron interferometer experiment by Colella, Overhauser, and Werner (1975), and follow-on experiments, manifestly require both (newtonian) gravity and quantum mechanics. A horizontal beam of cold neutrons is divided and recombined by a single-crystal interferometer. The interferometer is rotated so that one outgoing beam is still horizontal, but vertically displaced. It costs the neutrons $mg \approx 100\,\mathrm{neV/m}$ to climb up the interferometer, so the neutrons that take the bottom path have ever-so-slightly less momentum, and therefore a different wavelength $\lambda=h/p$, than the neutrons that take the bottom path; this results in a shift in the phase of the interference pattern that depends on the angle between the interferometer and the horizontal. While the gravitational effect has been observed, it doesn't quantitatively match the prediction of the Schrödinger equation with a linear potential. Speculation in the community is that the interferometers (which are hand-sized, and weigh several ounces) twist when tilted, changing the spacing between the paths and introducing an additional phase shift.
  

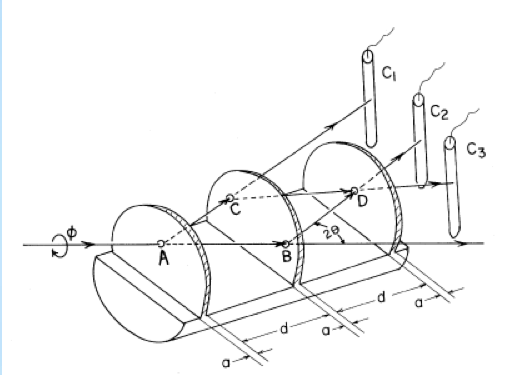
• Nesvizhevsky and collaborators (2002) (see also here or here) presented evidence that neutrons in a gravitational well may occupy only discrete bound states. They sent a horizontal beam of ultra-cold neutrons (total velocity ~ 5 m/s, vertical velocity quite small) through a narrow gap between a neutron mirror and a neutron absorber. When the gap was large, the neutrons could bounce off the mirror without touching the absorber and the transmission through the gap was large; when the gap was small, only neutrons with the smallest vertical velocities could shoot the gap without hitting the absorber. For gaps of a few tens of microns, the transmission shows evidence of becoming quantized: the transmission is zero up to a certain gap size, then steps up towards the continuum value as more bound states become available.
  

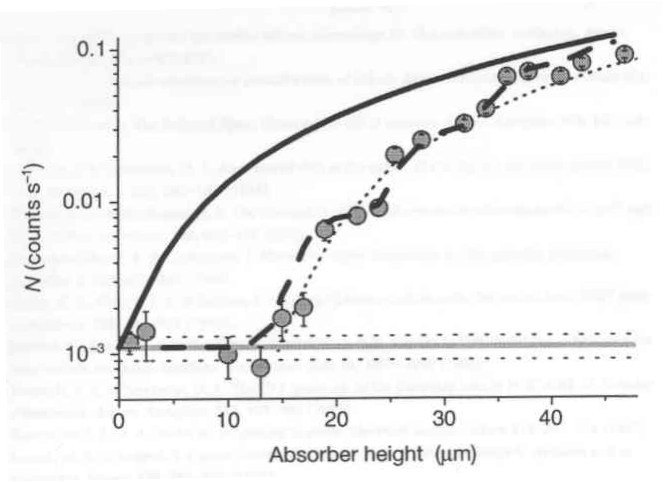
• Building on this work, Jenke et al. (2011) have used a vibrating table to drive transitions between gravitational bound states. Greene points out that this is the first experiment ever to drive a quantum-mechanical transition without using an electromagnetic field, using only the strong and gravitational forces.

Answer 4

It is currently possible to measure gravity between the single atom and the Avogadro number of atoms. The gravitational energy corresponding to the interaction of electron and proton at the distance of Bohr radius in term of h omega corresponds to omega = 10^-23 Hz and so 1 Hz will be obtained where the second mass is the Avogadro number of protons. This energy difference can be measured directly with so called Trojan wave packets in double Trojan cat states where two semi-classical states of the electron each moving on the circular orbit but in the opposite direction collide twice a period and interfere to the pattern. The interference pattern between two counter-rotating Trojan wave packets will shift as someone was rotating with 1 Hz above it and therefore readily measurable in delta ionization experiment. 1Hz resolution also corresponds to the best frequency resolution used in current atomic clocks that are electronic counters counting the number of microwave or optical oscillations between perfectly measured and defined atomic transitions.

---

RE: I am basically referring to the following paper http://journals.aps.org/pra/abstract/10.1103/PhysRevA.57.2239 which designs the quantum states of the neutral hydrogen atom to measure the weak including gravity effects on the single atom. The electron wave function is split into two components that more less look like the classical electrons. Those components have the angular momentum equal in the modulus but of the opposite sign. Because the phi part of the angular momentum wave function is the exp(m i phi) the collision of the packets twice the period of rotation of the each one amplifies the interference pattern around the circle that has 2 m bumps. The m=n and the higher the Rydberg state the better. When the gravity is applied against the atom in such a way that the left component accumulates different phase than the right one i.e. for example the other mass like neutron is in the plane of rotation and oscillates with the same frequency along the perpendicular to the line of phase accumulation (polarization) in time is of the order of the frequency G Mproton*me / r_0 / hbar where r_0 is of the order of the radius of the electron orbit. Because the linear Start effect on the circular state is 0 http://www.cqed.org/spip.php?article83 and therefore is 0 due to the normal gravity from the Earth or kilograms ball in the variation of this experiment one must use the Trojan wave packet and the excited Trojan wave packet to interfere as the cat superposition if they are to rotate around the same nucleus and one wants the mass relatively in the rest. Alternatively more difficult one electron cat states may be excited around two nuclei one-half of the electron around one and the second around the other and the gravitational Stark effect from the mass above the plane of the rotations can be used while it is placed above one of the nuclei or whatever implementation of the experiment described here http://journals.aps.org/pra/abstract/10.1103/PhysRevA.89.023607 can be imposed on the cat states of the single electron in the atom and other atoms or neutron instead of macroscopic masses and atom clouds. The interference pattern like in Michelson–Morley experiment starts to flow (shifts in time) like someone saw it being slowly rotating above such atom when the gravitational interaction is added. Now since there are sinus-like bumps in the wave function probability the ionization signal on short delta-like pulse is the function of time when the atom is subjected to the gravity of other atom or the Avogadro number of them. Since the number of bumps around the circle is 2 n the higher the quantum number the better. The best would be Rydberg atoms with some n= 1 million or above or giant atoms. Those would have some 50 meters of diameter. It the paper there are magnetic fields but this all applies to the gravity.