Uncertainty principle with two photons

Question

Imagine an experimental setup in which you have to measure the momentum and location of a particle. To measure it we know we will have to affect it, and the uncertainty principle would come into the picture, but I have a different setup. The classical setup is that you fire a photon to measure the location of the particle, but the particle will change its momentum due to the collision with the photon.

I decided to take two photons. I will shoot one photon from either side of the particle, so the effects of the two photons cancel each other, giving an accurate measurement. To understand this, see the picture below.

1. The classic experiment

   

2. My thought experiment

   

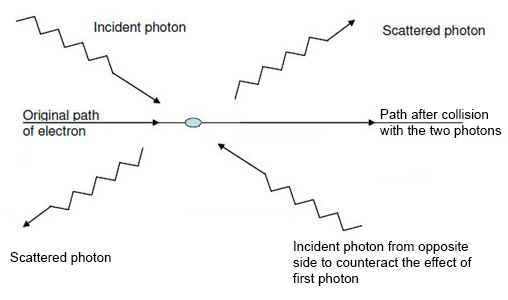
In the second experiment, we shoot a photon of the same energy as the first one and counteract the effect of the first photon, so the electron would continue on its original path. Please tell me where I am wrong.

EDIT

We will have to take multiple photons but equal from both sides and in opposite directions.

Answer 1

First of all, the uncertainty principle is more than just disturbance of observation.

From the Wikipedia article "Uncertainty principle":

Historically, the uncertainty principle has been confused with a somewhat similar effect in physics, called the observer effect, which notes that measurements of certain systems cannot be made without affecting the systems. Heisenberg offered such an observer effect at the quantum level (see below) as a physical "explanation" of quantum uncertainty.

It has since become clear, however, that the uncertainty principle is inherent in the properties of all wave-like systems, and that it arises in quantum mechanics simply due to the matter wave nature of all quantum objects. Thus, the uncertainty principle actually states a fundamental property of quantum systems, and is not a statement about the observational success of current technology. It must be emphasized that measurement does not mean only a process in which a physicist-observer takes part, but rather any interaction between classical and quantum objects regardless of any observer.

Now, you've drawn 'the' path of the electron as if the electron has a definite trajectory and that two photons of equal and opposite momentum interact with the electron at a definite location.

However, the state of definite position has maximum 'uncertainty' in momentum! Not only can there not be a definite trajectory but, if the electron is localized by an interaction, one cannot escape the inherent uncertainty of that localized state.

Answer 2

The problem with your set up is that you have ignored the quantum mechanical nature of the photons. The photons are subject to the uncertainty principle as well as the electron, and so there is no way to send in 2 photons with precisely the same momentum at precisely the same time, and we can't guarantee they will scatter of in precisely the same way. The setup you have described is, therefore, impossible.

Answer 3

What makes you think an electron reflects photons as you have drawn?

• Electrons scatter photons in any direction, although not uniformly. (Examples: Thomson scattering, Rayleigh scattering, X-ray crystallography)
• The electron may absorb the photon for an arbitrary period of time, changing momentum and thus position, then release a photon of a different wavelength. The electron may absorb one photon and release many photons. (Examples: Compton scattering, inelastic scattering)
• The photons could interact with each other and ignore the electron. (Examples: Delbrück scattering, additional two-photon physics)
• Even if you are lucky enough to have an interaction in which the electron and photons play along with your plan... Your experiment cannot distinguish which photon is which at the exit. They could have been "scattered horizontally" or "scattered vertically", leading to a great deal of position and momentum uncertainty in your system.

Answer 4

Your classic experiment 1) is quantum mechanical. Electrons and photons are elementary particles and interact individually as quantum mechanical entities.

The plot shows the elastic scattering of photon on electron, a computable process quantum mechanically thus the angular distribution is known. As we are talking quantum mechanics there is a probability for each angle where the electron may scatter that is given by the Klein-Nishina formula. So the individual photon-electron pair does not have a fixed angle which you could use in your thought experiment to counteract the effect on the electron's direction. ( always presuming you could time the photons to hit at the same delta(t) of Heisenberg's uncertainty principle) .

The thought set up 2) is impossible.

Answer 5

It has been said that your second experiment is impossible. For me it is possible but can't be used to predict the position of the electron and its momentum.

• First consider that your "classic experiment" is true. In this experiment, the important fact is that one can't obtain the momentum of the electron when the photon angle is measured.
• Now, consider your "thought experiment" with this modification: we send two photons as you said, but we are not able to let them hit the electron at the sime time. Instead they interact with the electron in two different times. And this difference in time is $\Delta t$ and can be arbitrarly small.
• Now, let's (reasonabely) suppose that if I compute the state of the final system for my "thought experiment", then it will depend by $\Delta t$. So, if I send $\Delta t\rightarrow 0$ then I obtain a prediction for your "thought experiment."
• In my experiment one photon hit the electron and then it works like the "classic experiment": the first photon scatter randomly and you can't infer the new momentum of the electron. The second photon will hit the scattered electron and will do the same thing as the first one: you can't obtain the new momentum of the elctron from this new photon either.
• This result does not depend on $\Delta t,$ so it should hold also in the limit $\Delta t=0.$ (that is your experiment)