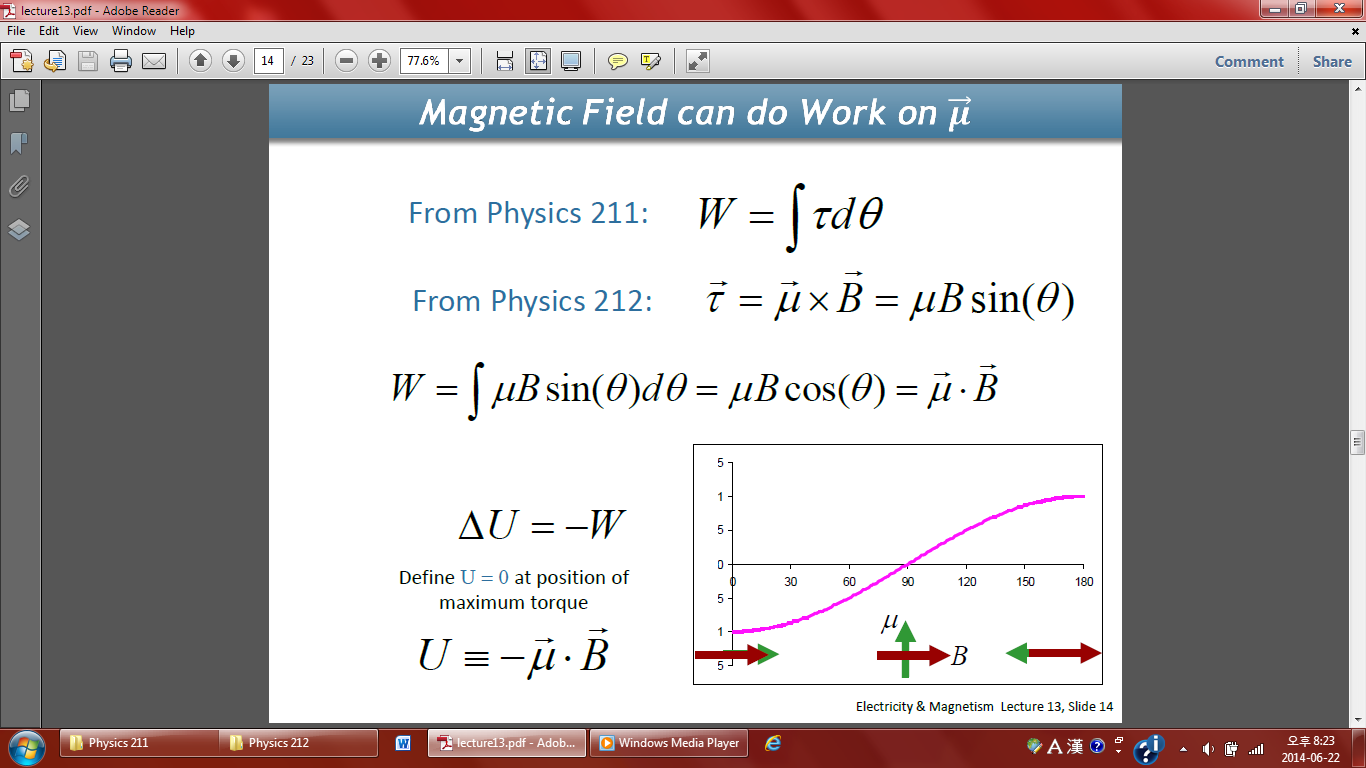
In the above slide, it reads that $W=\int\mu B sin(\theta)d\theta=\mu B cos(\theta)$.
But, I thought that $\int\mu B sin(\theta)d\theta=-\mu B cos(\theta)$ by some calculus calculation.
Can somebody explain more explicitly, please?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ Whoever you are, if you are reading my question, and want to get the correct answer, Read my last four comments written in the below answer. (You have to "click" the "Show 9 more comments" next to the "add a comment" to see those comments.) $\endgroup$– UserCommented Aug 10, 2014 at 0:42
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
14
This is an issue of not specifying the initial and final states (or whether we are calculating the work done by the magnetic torque, or the work required by an external torque to oppose the magnetic torque). In reality, what we should say is that if we start with a magnetic dipole $\vec{\mu}$ that is aligned with the magnetic field (so that $\theta=0$), WE must do work to rotate the dipole to a new angle $\theta$, by applying the torque opposite to the magnetic torque.
Let's look at the fact that zero potential energy is defined as when $\theta=\pi/2$. Then we can look at the work done by the magnetic field as it rotates from $\theta$ to $\pi/2$: $$W=\int\tau\,d\theta=\int_{\theta}^{\pi/2}\mu B\sin\theta'\,d\theta'=-\left.\mu B\cos\theta'\right|_{\theta}^{\pi/2}=\mu B\cos\theta$$ So that's where the negative sign went.
-
$\begingroup$ I'm not so sure why we define that the potential energy is zero when $\theta = \pi/2$. I thought we are deriving the potential energy from the work, not the work from the potential energy. Then, how do you know the potential energy is zero at $\theta=\pi/2$ even though we haven't derived the work done by the magenetic field on the magnetic dipole? $\endgroup$– UserCommented Jun 23, 2014 at 3:27
-
$\begingroup$ In general, the work needs to be defined from some starting position/angle to some final position/angle: $$W=\mu B(\cos\theta_i-\cos\theta_f)$$ Then we have $\Delta U=U_f-U_i=-W$, but we are free to choose the zero at any point we want since it's a potential energy (let's say $U=0$ when $\theta=\pi/2$). This gives the desired result, but of course you could choose any other zero point you wish. $\endgroup$ Commented Jun 23, 2014 at 4:02
-
$\begingroup$ Ok. So, we just define $U=0$ at $\theta=\pi/2$ without any reason, right? But then, why do you calculate the work done by the magnetic torque from $\theta$ to $\pi/2$ not from $\pi/2$ to $\theta$? It seems that you are calculating work done by an external force to oppose the magnetic torque, not by the magnetic torque which is opposite to the work done by an external force to oppose the magnetic torque. $\endgroup$– UserCommented Jun 23, 2014 at 19:07
-
$\begingroup$ Perhaps the easiest way to look at it is: it's the same problem with the physics vs. chemistry definitions of $W$ in the 1st Law of Thermodynamics; $\Delta U=Q\pm W$. The plus sign is used by chemists, who talk about $W$ being the work done ON the system in question, while physicists use $W$ as the work done BY the system. In this case, the system is the dipole, and the magnetic field exerts a torque ON and does work ON the dipole, so we should really use $\Delta U=+W$, in which case your calculus intuition gives you exactly what you'd expect. $U=0$ is then convention. $\endgroup$ Commented Jun 24, 2014 at 1:31
-
$\begingroup$ I think $\Delta U=+W$ is not true since if it is true, then, it would not consistent with the result above, $U=-W=-\mu\cdot B$... $\endgroup$– UserCommented Jun 24, 2014 at 3:13
$\begingroup$
$\endgroup$
First, we define the direction of $\vec{\theta}$ between $\vec{\mu}$ and $\vec{B}$ to be positive if $\vec{\theta}$ is coming out of the screen in the sense that $\theta$ is increasing with the right-hand-rule in the counter-clockwise direction.
Then, note that the torque $\vec{\tau}=\vec{\mu}\times\vec{B}$ makes the angle between $\vec{\mu}$ and $\vec{B}$ decrease in order to line $\vec{\mu}$ with $\vec{B}$. (Note that we are defining the angle $\theta$ between $\vec{\mu}$ and $\vec{B}$ to be the acute angle between them which results in $0\leq\theta\leq \pi/2$.) Therefore, the direction of the torque $\vec{\tau}$ on the dipole is Negative, in the sense that the direction of the torque vector $\vec{\tau}$ is the opposite direction to the angle vector $\vec{\theta}$.
Then, we choose $\theta_0=\pi/2$ to be the initial angle of the work done by the magnetic field on the dipole, in order to define $U=0$ at $\theta_0=\pi/2$.
Then, we have the work done by the magnetic field on the dipole as follows: $W=\int_{\theta_0}^{\theta}\vec{\tau}\cdot d\vec{\theta}=\int_{\pi/2}^{\theta}\vec{\tau}\cdot d\vec{\theta}=\int_{\pi/2}^{\theta}(-\tau d\theta)=\int_{\theta}^{\pi/2}\tau d\theta=\int_{\theta}^{\pi/2}\mu B\sin(\theta) d\theta=-\mu B\cos (\theta)|_{\theta}^{\pi/2}=\mu B\cos(\theta)=\vec{\mu}\cdot\vec{B}$.
Then, we have the potential energy function by the magnetic field on the dipole $U_{\mbox{magnetic field}}=-W=-\vec{\mu}\cdot\vec{B}$, in the sense that the function $U$ is defined to be zero at the initial angle $\theta_0=\pi/2$ which is the angle when the magnitude of the torque $|\vec{\tau}|=|\vec{\mu}\times\vec{B}|=\mu B \sin (\theta)$ is maximum as requried in the slide.