Finding the Work done

Question

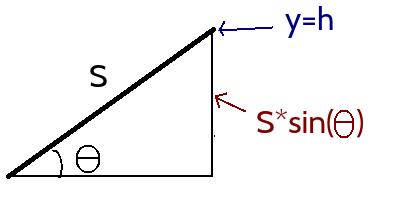
Find the work done for an object to slide down an inclined plane against the frictional force.

The answer my book shows is : $$ W = mg ({\mu}_k \cos \theta - \sin \theta) S $$ where S = displacement done by the object down the inclined plane and the other symbols have their usual meaning.

Well, when I saw the question first time, I thought we may find the Total Energy of the object at initial and final state and then find the change in Energies (Total) which will be equal to the Work done by the object. But, following this approach I came to the following expression (in bold) :

Total Energy at starting point, A = P.E = mgh

Total Energy at final point, B = K.E = $ \cfrac{1}{2} mv^2 = \cfrac{1}{2} m (2gS) = mgS$

So, Change in Energy of the object in sliding down the inclined plane from A to B is:

$$\textbf{Change in Energy} = mgS - mgh $$ The above expression looks far different from the answer given by book. Is my approach somewhere wrong? If it is right, then how can I simplify it further to get the answer similar to the book's one. Any help will be greatly appreciated.

Answer 1

Mechanical energy is not conserved since friction acts on the system. Also recall that the displacement done by the object down the inclined plane is not the difference in height.

The answer shown comes from an analysis of the forces that are involved in the movement of the object. Draw a body-diagram and you will get $mg(\mu_k \cos \theta - \sin \theta)$ as the magnitude of the force, and $S$ will be the displacement.

Answer 2

Well first off, I think you forgot that $S$ was the displacement along the incline. The object will slide down from $y=h$ to $y=h-(S\sin(\theta))$ (change in vertical displacement).

The only work done here is by friction and by gravity. We know gravity is doing positive work and that friction is doing negative work.

The work done by friction is simply the friction force exerted on the object by the incline (normal force), given by

$$W_f=-mg\cos(\theta)\mu_k S$$

The work done by gravity on the object is simple given by

$$\Delta\,PE = mg\left(y-\left(y-(S\sin(\theta))\right)\right) = mg(S\sin(\theta))$$

Add these two together and voilà,

$$mgS\left(\sin(\theta)-\cos(\theta)\mu_k\right)$$

But this is the work done on the object, so to get the work done by the object just multiply the whole thing by $-1$.