0
$\begingroup$

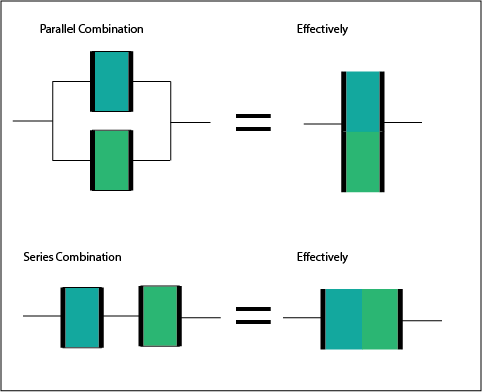
I can't seem to find any information about how parallel capacitors or capacitors in a series affect how much a capacitor can charge, not in my textbook or online. Here is the question I am trying to answer:

You have a capacitor that you will connect across a battery. If you wish to increase the total charge drawn from the battery, which of the following options will work? Choose all of the correct answers.

(a) Add a larger capacitor in series with the first.

(b) Add a smaller capacitor in series with the first.

(c) Add a larger capacitor in parallel with the first.

(d) Add a smaller capacitor in parallel with the first.

I would think that the charge $Q$ would increase as the capacitance $C$ increases according to $Q=CV$, and I know that $C$ increases with increased area, decreased separation, or a higher dialetric constant, but that has nothing to do with the problem. I think $C$ also is higher with parallel capacitors than capacitors in a series, but am unsure.

Improve this question
$\endgroup$
4
  • 2
    $\begingroup$ You almost have the answer in your question. Which arrangements increase the effective area of the overall capacitance? $\endgroup$
    – garyp
    Commented May 31, 2014 at 1:03
  • $\begingroup$ For parallel capacitors we are essentially combining the areas of all the capacitor plates when they are connected with conducting wire, and capacitance of parallel plates is proportional to area. But I don't know for series. Wouldn't a series combination increase the area as well? $\endgroup$
    – user47611
    Commented May 31, 2014 at 1:11
  • 1
    $\begingroup$ Good observation and excellent question. For the series combo, how about the effective capacitor plate separation? $\endgroup$
    – garyp
    Commented May 31, 2014 at 1:53
  • $\begingroup$ I'm going to be a spoilsport and just point you to Capacitors in Parallel and Series. This will show you how to calculate the total capacitance, and you'll also see what garyp has been hinting at. $\endgroup$
    – John Rennie
    Commented May 31, 2014 at 10:05

3 Answers 3

Reset to default
3
$\begingroup$

This image should help you understand.

enter image description here

As you can see parallel combination increases area but not plate separation. And series combination increases plate separation and not area.

Since you understand the rest of the required concept, I would not rid you of the joy of finding out the answer yourself!

Improve this answer
$\endgroup$
1
  • $\begingroup$ Great illustration - just to the point. $\endgroup$
    – Volker Siegel
    Commented Jun 5, 2014 at 13:45
0
$\begingroup$

both C & D are correct....Bcoz whenever you add capacitors of any value in parellel to a capacitor keeping other circumstances unchanged the potential drop across the original capacitor and thus the total charge across tha same capacitor remains unchanged...But i addition the battery will supply some amount of charge to the new one capacitor to charge it to the potential drop equal to that across the battery....So what ever be the value of the capacitor being added in parellel will pull more amout of charge from the battery as compared to the original situation...

Now in the case of the series connection of the capacitors the net capacitance will be always lesser than the original capacitance...And thus charge drawn from battery will also decrease...

Improve this answer
$\endgroup$
0
$\begingroup$

We know that $$Q=C_{eq}V$$

So if we will increase the value equivalent capacitance (i.e. $C_{eq}$) of the circuit then we will be able to get more charge from the battery.

As $$Q\propto C_{eq}$$

Hence I think option (C) will work as here adding a larger capacitor in parallel with otherwill increase the value of $C_{eq}$ as it's value will directly get added up to the value of other capacitor resulting in larger transfer value of $C_{eq}$ and hence larger value of $Q$.

 

Important Note: Above I had said that $Q\propto C_{eq}$. It's is of course true but also do keep in mind that it's converso (i.e. $\color{red}{C_{eq}\propto Q}$) is completely wrong. Hence $\require{enclose}\enclose{horizontalstrike}{\color{red}{C_{eq}\propto Q}}$

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.