I want to ask if anyone has gone through the derivation of the second equality in the following formula
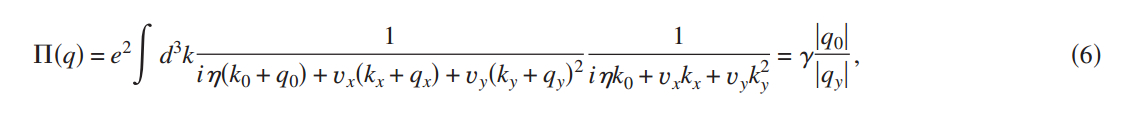
which comes from http://journals.aps.org/prb/abstract/10.1103/PhysRevB.80.165102.
I want to ask if anyone has gone through the derivation of the second equality in the following formula
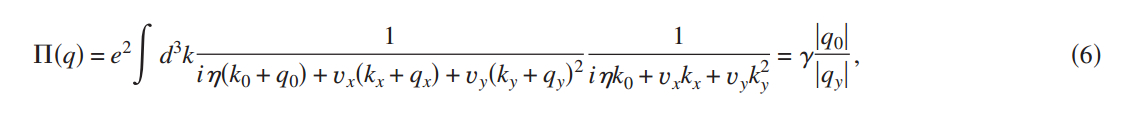
which comes from http://journals.aps.org/prb/abstract/10.1103/PhysRevB.80.165102.
Note that "$v_y$" is not velocity. Physically it is the local curvature of the Fermi surface. Also it is possible to treat one amongst the 4 constants (sic) $\eta, v_x, v_y, e$ as an overall scale and drop it from the action. Here I do this with "$v_x$", and write the propagator as $$ G(k)^{-1} = i\eta k_0 + k_x + C k_y^2,$$ where $\eta$ and $C$ are positive. In this convention the boson's self-energy is $$ \Pi(q) = e^2 \int \frac{d^3 k}{(2\pi)^3} ~ G(k+q) G(k).$$
It is convenient to change coordinates: $ (k_x, k_y) \mapsto (\epsilon_k, k_y)$, and integrate over $\epsilon_k$ using contour integration. This leads to $$ \Pi(q) = i e^2 \int \frac{dk_0 k_y}{(2\pi)^2} ~ \frac{\Theta(k_0 + q_0) - \Theta(k_0)}{i\eta q_0 + C q_y^2 + q_x + 2C q_y k_y}.$$ Next we integrate over $k_y$ using contour integration to obtain $$ \Pi(q) = \frac{e^2}{4 C |q_y|} \mbox{sign}(q_0) \int \frac{dk_0}{2\pi} ~ [\Theta(k_0 + q_0) - \Theta(k_0)] = \frac{e^2}{8 \pi C} \frac{|q_0|}{ |q_y|}.$$ It is important to note that the self-energy depends in a singular way on the curvature $C$.