I have read the explanation for this in several textbooks, but I am struggling to understand it via Archimedes' principle. If someone can clarify with a diagram or something so I can understand or a clear equation explanation that would be great.
$\begingroup$
$\endgroup$
2
asked Apr 30, 2014 at 14:14
-
3$\begingroup$ As an aside, I think it is important to point out that there should be enough liquid water in the container for buoyancy to take place. $\endgroup$– VendettaCommented Jun 18, 2019 at 17:24
-
$\begingroup$ Related: Does the sea level increase if an iceberg melts? $\endgroup$– VishnuCommented May 9, 2020 at 10:21
Add a comment
|
9 Answers
$\begingroup$
$\endgroup$
26
Good question.
Assume we have one cube of ice in a glass of water. The ice displaces some of that water, raising the height of the water by an amount we will call $h$.
Archimedes' principle states that the weight of water displaced will equal the upward buoyancy force provided by that water. In this case,
$$\text{Weight of water displaced} = m_\text{water displaced}g = \rho Vg = \rho Ahg$$
where $V$ is volume of water displaced, $\rho$ is density of water, $A$ is the area of the ice cube base and $g$ is acceleration due to gravity.
Therefore the upward buoyancy force acting on the ice is $\rho Ahg$.
Now the downward weight of ice is $m_\text{ice}g$.
Now because the ice is neither sinking nor floating, these must balance. That is:
$$\rho Ahg = m_\text{ice}g$$
Therefore,
$$h = \frac{m_\text{ice}}{\rho A}$$
Now when the ice melts, this height difference due to buoyancy goes to 0. But now an additional mass $m_\text{ice}$ of water has been added to the cup in the form of water. Since mass is conserved, the mass of ice that has melted has been turned into an equivalent mass of water.
The volume of such water added to the cup is thus:
$$V = \frac{m_\text{ice}}{\rho}$$
and therefore,
$$Ah = \frac{m_\text{ice}}{\rho}$$
So,
$$h = \frac{m_\text{ice}}{\rho A}$$
That is, the height the water has increased due to the melted ice is exactly the same as the height increase due to buoyancy before the ice had melted.
Edit: For completion, since it is raised as a question in the comments
Melting icebergs boost sea level rise, because the water they contain is not salty.
Although most of the contributions to sea-level rise come from water and ice moving from land into the ocean, it turns out that the melting of floating ice causes a small amount of sea-level rise, too.
Fresh water, of which icebergs are made, is less dense than salty sea water. So while the amount of sea water displaced by the iceberg is equal to its weight, the melted fresh water will take up a slightly larger volume than the displaced salt water. This results in a small increase in the water level.
Globally, it doesn’t sound like much – just 0.049 millimetres per year – but if all the sea ice currently bobbing on the oceans were to melt, it could raise sea level by 4 to 6 centimeters.
-
10$\begingroup$ @MattThrower But that illustrates that ice is less dense than water, which is why ice is buoyant to begin with... But ice will only displace a volume of water equivalent to the volume of ice that is below the water level... They cancel each other out? $\endgroup$ Commented Apr 30, 2014 at 17:49
-
4$\begingroup$ Aha, so they counter balance perfectly? I had no idea. That's the danger of knowing more chemistry than physics :) $\endgroup$– Bob TwayCommented May 1, 2014 at 7:58
-
3$\begingroup$ So, does this mean melting polar ice caps shouldn't effect sea levels, those floating on the sea anyhow? $\endgroup$– JodrellCommented May 1, 2014 at 8:18
-
3$\begingroup$ @Jodrell, the case for polar ice caps is different, since the icecap will melt to form fresh water, where as the surrounding ocean water is salty (differing densities). The above analysis only applies if the floating solid melts to form the same liquid initially supplying buoyancy force. $\endgroup$– KenshinCommented May 1, 2014 at 8:48
-
3$\begingroup$ @Jodrell: It's the big sheets of ice sitting on Greenland and Antartica that everyone worries about. $\endgroup$– user5174Commented May 1, 2014 at 8:48
$\begingroup$
$\endgroup$
8
Here is an explanation that needs no explicit equations.
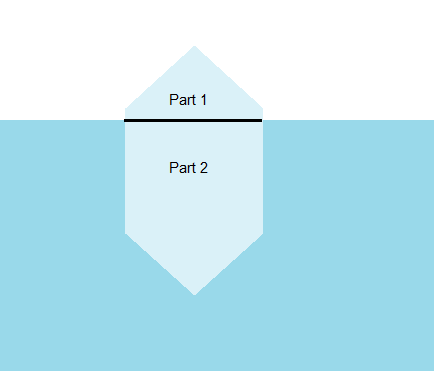
Consider the following diagram, in which part1 and part2 represent the ice.
The displaced water volume equals part2 volume and has as much mass as (part1+part2)
Now look at what happens when both part1 and part2 melt:
- their mass does not change, it is (part1+part2)
- it becomes water.
And we just said that part1+part2 mass water has part2 volume.
-
1$\begingroup$ You mention "The displaced water volume equals part2 volume and has as much mass as (part1+part2)". Why does the displaced water, which has
part2's volume, have as much mass aspart1 + part2? The black line that separatespart1frompart2seems to be arbitrarily drawn. $\endgroup$ Commented May 2, 2014 at 14:09 -
5$\begingroup$ The line is not arbitrary, part2 is immersed, and therefore it is the part directly responsible for displacing the water. $\endgroup$ Commented May 2, 2014 at 14:35
-
1$\begingroup$ Yeah, I think I get that part. But why is the mass of the displaced water equal to the mass of
part1 + part2? That water's volume is definitely the volume ofpart2, but I'm not intuiting why it's equal to the mass of both parts. $\endgroup$ Commented May 2, 2014 at 14:44 -
11$\begingroup$ That is Archimedes' principle in application. Keep in mind that the ice density is less than that of water, therefore, a lesser amount of water is required to balance the whole ice body. $\endgroup$ Commented May 2, 2014 at 15:21
-
3$\begingroup$ The whole ice block has to be balanced, so the upwards force (gravity times density of water times volume of ice) must equal downward force, which is gravity times mass of ice. Canceling gravity, we get (mass of water that part 2 occupies) = (total mass of block). $\endgroup$ Commented Jul 28, 2015 at 1:53
$\begingroup$
$\endgroup$
0
Brandon, above, gets right to the point. Frozen water displaces its own mass in the rest of the water, which means in effect it displaces an amount equal to itself. While frozen it is larger in volume, and thus less dense, because of hydrogen bonding -- that's why it floats -- and when it melts it returns to the liquid state (surprise!) at essentially the same density as the surrounding water. A given quantity of water, temporarily larger in volume but correspondingly less dense because it has frozen, returning to the liquid state will thus not raise the overall level of water (assuming here no evaporation, mosquitoes stopping in to have a sip, etc etc) --
$\begingroup$
$\endgroup$
I've seen this question some years ago. Note that the water level doesn't change as the ice melts ONLY if the ice is melting in pure water. If you melt ice cubes in salt water, the water level will increase as the ice melts.
answered Jul 28, 2015 at 3:33
$\begingroup$
$\endgroup$
Consider an ice cube of 10cm, assume that the density of water and ice is 10:9. At first, the ice has 9 cm in the water, when it melts, it becomes water with the volume 10*10*9. Just fills the melted ice original in the water.
answered Apr 30, 2014 at 15:00
$\begingroup$
$\endgroup$
The displacement answers of mew and kal are spot on.
This is about the chemistry of the displacement.
Water is the only substance with solid density less than the liquid. (For the record there is one other, namely the element Gallium).
As you cool a liquid and it settles in and get more dense. As it settles to solid it typically just settles in more.
Water is very a interesting molecule in that it is very stable and still polar. Two hydrogen and one oxygen in a triangle. The oxygen is slightly negative and the hydrogen positive. When they settle into a solid they form a lattice that is less dense than the liquid state. Water has a maximum density as 4 Celsius.
If ice was more dense than water we would be on a much much different earth. We would probably not be on this earth.
$\begingroup$
$\endgroup$
3
Another non-math way to look at it.
- The water level in a container is going to be determined by:
the volume of water already in the container, which we can assume to be constant, plus
how much water's displaced by anything we float in it, or any extra water we add to it (the displaced volume). If we can show that stays constant as ice turns to meltwater, then the water level must be constant.
Freezing and melting water doesn't make it gain or lose any extra atoms or other mass. Melting 1kg of ice results in 1kg of water. Only the volume changes.
The volume of water displaced by a buoyant object depends only on the mass of the object, not on its volume or density.
Since freezing doesn't change the mass, it doesn't change the volume of displaced water, so the water level doesn't change.
-
$\begingroup$ "The volume of water displaced by a buoyant object depends only on the mass of the object, not on its volume or density." This is the point that would need math however. This is far from trivially obvious $\endgroup$– CruncherCommented Dec 5, 2022 at 14:55
-
$\begingroup$ @Cruncher I think I'd expect Archimedes' "principle of flotation" to be intuitively obvious, or at least my own understanding of it doesn't seem to have needed math to arrive at. What am I missing? But given that you don't consider it obvious, and you do consider it a math problem, it suggests I've missed something important. What'm I missing? $\endgroup$ Commented Dec 5, 2022 at 23:32
-
$\begingroup$ Love this answer for a general public, thanks. $\endgroup$ Commented Sep 15, 2023 at 18:29
$\begingroup$
$\endgroup$
4
While the two existing answers offer some good science, on why the water level does not change, they are both slightly over optimistic. While the level will not rise, it will not remain the same either. Evaporation will occur simultaneously with melting, and the water level in the glass will actually lower slightly.
Even if the ice remains frozen the process of Sublimation will allow the ice to evaporate. You have may seen this occur in your freezer, or with snow on your porch
-
2$\begingroup$ You are neglecting possible condensation of water vapor from the air on the ice, which would have the opposite effect. $\endgroup$– OldcatCommented Apr 30, 2014 at 22:03
-
$\begingroup$ @Oldcat, There are several variables, container design, humidity, air temp & water temp. Evaporation occurs with all the variables, I was assuming the condensation would occur in about half the scenarios, so left it out for simplicity. You are welcome to add it in to my answer if you believe I was mistaken on the ratio of condensation scenarios. $\endgroup$ Commented May 1, 2014 at 10:32
-
2$\begingroup$ Evaporation? That is weak. Clearly the intent of the question is to ignore evaporation. $\endgroup$ Commented Jul 28, 2015 at 1:20
-
2$\begingroup$ @Oldcat And the intent of the question is clearly to ignore condensation. $\endgroup$ Commented Jul 28, 2015 at 1:24
$\begingroup$
$\endgroup$
1
It does, it lowers it. Frozen water takes up more space that liquid water (you'll notice it floats -that is because there is less mass per volume). As it melts it takes less space per area thus the water level goes down.
-
1$\begingroup$ You're forgetting that the mass of the ice is the same mass as the water that froze to make it. Floating objects only displace their mass worth of fluid. $\endgroup$ Commented Apr 30, 2014 at 23:29