6
$\begingroup$

Suppose a wheel is rolling smoothly on a horizontal plane i.e., it is rolling without slipping.

Now let's take the two cases of the horizontal plane:

  1. It has friction

  2. It is frictionless

In the first case, as of my knowledge friction doesn't come into play when there is no slipping. So, will the wheel roll forever? If it comes to rest is the answer friction? If not what brings it to rest?

In the second case, there is no friction. So, here also will the wheel roll forever? And if it comes to rest what brings it to rest?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
8
$\begingroup$

When the wheel is rolling without slipping, rolling resistance brings the wheel to rest. Here friction is not the right answer let me explain you how.

This friction is not the answer why it comes to rest because friction comes into play to oppose any slippage. The concept of friction most books provide are deficient. The term "rolling friction" is also a misnomer.

The correct explanation for it is rolling resistance for which the word "rolling friction" is often used creating confusions.

Let me explain you rolling resistance and how consideration of friction gives wrong conclusions (I have provided one link explaining rolling motion and correct concept of friction at the end of this answer):

Consider a wheel rolling smoothly. what is the direction of friction force? We might think it must be opposite to the direction of motion thats why it will stop after some time. But, this friction force is providing a torque also making its angular velocity to increase. So, we might think we took the direction of friction force wrongly. So, we take the direction of friction force to be in the same direction of motion which again gives wrong result. Here is the paradox!

Here comes the role of normal reaction. A perfect rigid body doesn't exist. Rolling dynamics in the real world of non-rigid elastic materials is a complex interplay of contact forces due to deformation, and consideration of friction alone can lead to contradictory and unrealistic conclusions. The contact between the wheel and the surface on which it is moving is a surface. This surface is formed due to deformed shape of either the wheel or the surface on which it is moving or both. Because the deformations are not symmetric since the wheel is moving forward, on that surface the reaction forces on the forward portion of the surface is more than that on the backward portion, giving a torque to the wheel in counter-clockwise direction.

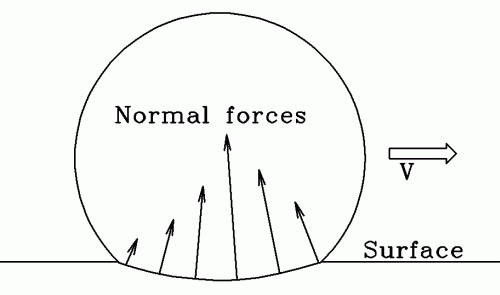
enter image description here

Wheel rolling to the right, with surface deformation. The deformation is greatly exaggerated. Normal force components across the deformed region are not uniform in size. They are greater on the forward side, producing a counterclockwise net torque.

This results in slowing down the speed and eventually comes to rest. There are other two cases when the wheel deforms but the surface doesn't and the wheel doesn't deform but the surface does. Please read the content in the link provided.

In the 2nd case if friction is not present the wheel will simply slide forward on the surface. This will no longer be rolling.

Please read this for more details: http://lockhaven.edu/~dsimanek/scenario/rolling.htm

Improve this answer
$\endgroup$
2
  • $\begingroup$ the link is really helpful $\endgroup$
    – zeal
    Commented Apr 25, 2014 at 8:15
  • $\begingroup$ this is a nice answer, in high school physics students live in an ideal version of the world where the ground is non-deformable :) $\endgroup$
    – Aditya Garg
    Commented Sep 17, 2020 at 5:53
1
$\begingroup$

In the first case the wheel will keep rolling if the Rolling resistance is zero. But it will come to rest soon if there is a rolling resistance.

The second case: You are misinformed a bit. For rolling we need static friction because the bottom of the rolling object must be instantaneously be at rest(acc. to the definition of rollin). For this there has to be a the static friction.

Improve this answer
$\endgroup$
1
  • $\begingroup$ It is not true that for rolling without slipping there has to be static friction. If the velocitiy of the center of mass and the angular velocity are perfectly balanced as $v=\omega R$, the object can roll without slipping with no need for friction. $\endgroup$
    – thedude
    Commented Aug 9, 2018 at 13:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.