0
$\begingroup$

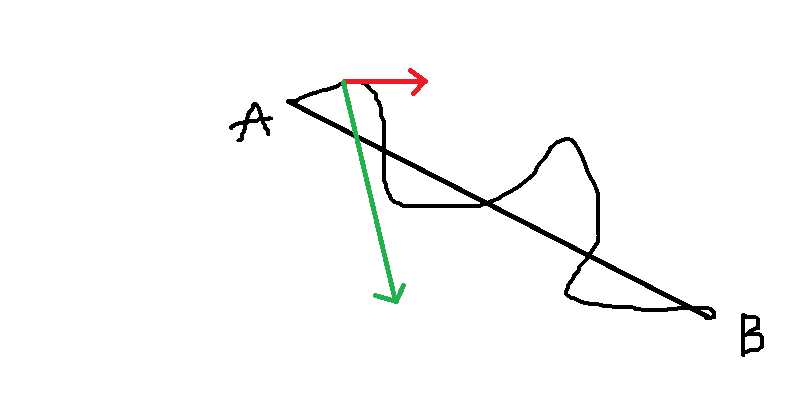
enter image description here

Sorry for the ugly picture but it makes my question more understandable. The $\Delta V$ from $A$ to $B$ is calculated by$$\int_A^B E \, \mathrm{d}r$$ where $r$ is the distance between $A$ and $B$. The curve line is the real path the charge take going from $A$ to $B$. Suppose that the green arrow is the $\vec E$ and the red arrow is $dl$ at some arbitrary point on the path the charge move. From what I learned from class $$\int_A^B E \, \mathrm{d}r = \int_A^B E \, \mathrm{d}l$$ This is the point I don't understand and make me reconsider what I learn about work in physics. Can someone explain why $\int_A^B E \, \mathrm{d}r = \int_A^B E \, \mathrm{d}l$

Does the calculation of the work involve vector and $E\, \mathrm{d}l$ here is the dot product of $\vec E$ and $\vec {\mathrm{d}l}$ or it is calculated by projecting all the force exert to the object to the axis created by start point and end point?

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ You are missing a dot product. This throws in a cosine of the angle between the electric field and your direction vector. This will make it that your integral is really are only summing the amount of the electric field in the direction of integration. Try googling line integrals for more info. $\endgroup$
    – jerk_dadt
    Commented Mar 20, 2014 at 4:43
  • $\begingroup$ so the the work here is the sum of all dot products of the $\vec E$ and the Infinitesimal "distance" (sorry I don't know what word to describe $\vec {dl}$) at all point on the path the charge take to move from A to B. That means it is $\vec E \vec {dl}$. It is hard to get the full detailed formula of Work. $\endgroup$
    – aukxn
    Commented Mar 20, 2014 at 4:52
  • $\begingroup$ People often use "differential path element" or "infinitesimal path element" for $\mathrm{d}\vec{l}$, and also sometimes drop the "element". $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Mar 20, 2014 at 4:57

1 Answer 1

Reset to default
1
$\begingroup$

The dot product is missing. The integral must also be multiplied by a Cosine of the angle between the vectors. $dl$ and $dr$ are the same thing. It's just an infinitesimally small distance on the direction of the field.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.