Function for curved line in log-log-plot

Question

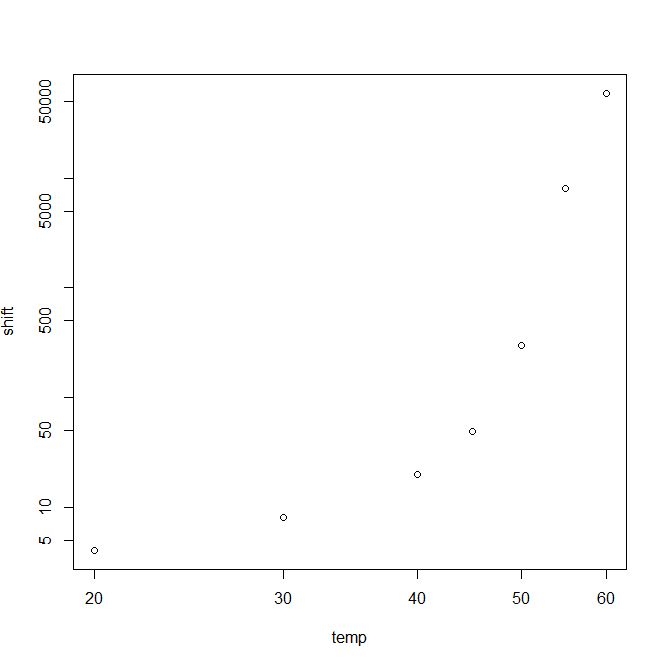
I've done experiments on the thermo-viscoelasticity of liver tissue and the following results

temp shift
1   20     4
2   30     8
3   40    20
4   45    49
5   50   300
6   55  8000
7   60 60000

_{(source: npage.de)}
.

show a curved line in a loglog-plot, which means I can't use a power-law function to approximate my data.

Do you have any idea what kind of function might work for my data? I've tried superlinear functions of the type y(x) = a^x^b but that doesn't seem to lead to a good fit.

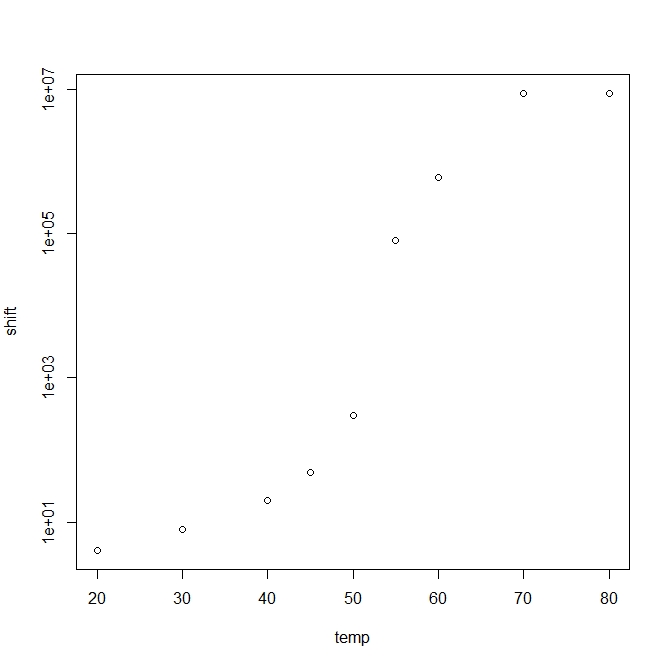
I'm adding a plot of the same data plus 2 additional temperatures (70 and 80).

temp shift

1 20 4

2 30 8

3 40 20

4 45 49

5 50 300

6 55 80000

7 60 600000

8 70 9000000

9 80 9000000

_{(source: npage.de)}
.

Answer 1

I think you have two distinct thermally activated processes going on here. If I do a log-linear plot of your data I get:

It looks to me as if below 45°C the points lie on a straight line and above 45°C the points lie on a steeper straight line. If I do a linear regression of the points below 45°C and above 45°C I get the fits:

Below 45°C:

$$ S = 0.771 e^{0.0805 T} $$

Above 45°C:

$$ S = 1.16 \times 10^{-9} e^{0.530 T} $$

So over the whole temperature range the shift is given by:

$$ S = 0.771 e^{0.0805 T} + 1.16 \times 10^{-9} e^{0.530 T} $$

If I graph the data and the fit together I get:

and this looks a pretty good fit to me.

Answer 2

What happens at higher temperatures? Is there an upper asymptote? There appears to be an inflection point around temp=53. This is visible on the log-linear plot. I would try fitting a logistic function http://en.wikipedia.org/wiki/Generalised_logistic_curve as the relationship looks more sigmoidal than exponential (assuming the data is accurate).