You do not give any indication of your physics background so I will answer in simple terms.
Are they saying, for example, that a source which has a flux emanating from it can be treated as a point particle, even if it's spherical?
How can spatially extended objects behave like point particles?
A simple example is the solar system. To first order the force controlling the orbits is gravity.
We have a simple gravitational equation about the gravitational field from a source and the position of a point particle in this field, an inverse square law.
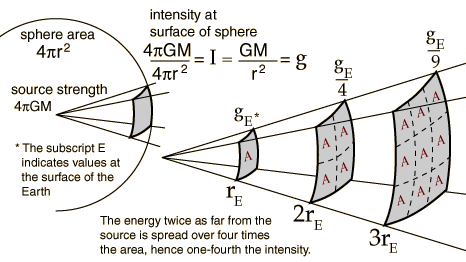
This assumes that the force from the source comes also from a point. This is possible if the distances examined are very much larger, orders of magnitude, than the dimensions of the masses producing the field. This is the case for the planets and the solar system. More so if one is thinking of stars. It is an approximation which works very well for calculating the orbits of the planets or the moon. The "point" used is the center of mass of the objects under study.
Even though the inverse square law holds for all gravitational attraction, the gravitational field differs when the dimensions are small with respect to a large mass generating the field. Here are the differences of the earth's gravitational field, for example, where one can no longer assume point locality of the source and would have to model the field by the map, integrating over a distribution of point sources from the earth.

The fact that gravity is a very weak force for dimension of objects around us, also allows us to calculate from the center of mass of a falling object , a brick, or a ball, except that we have to take into account kinematics of rotation and of friction and lift,( in case of a balloon): this will depend on the shape of the body and the type of mass and integrations will have to take place before a trajectory can be defined with small errors. All in all the center of mass is a good first order approximation to the behavior under gravitational fields.
The same is true for charge. When far away from the dimensions of the charge which produces the electric field, the center of mass is a very good approximation. When one works close to the charged bodies one has to integrate over charge distributions. Electromagnetism though is a stronger force and the charge distributions are more important than the gravitational distributions for similar masses. You could say that these distribution introduce a non locality, calculable though.


