0
$\begingroup$

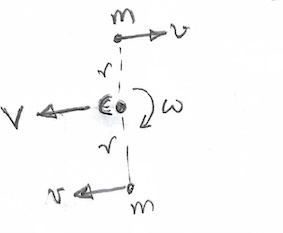
I saw the formula relating the kinetic energy of the system, which is $$K \ \text{(of system)} = K \ \text{(of centre of mass)} + K \ \text{(about centre of mass)},$$ where $K$ is the kinetic energy.

If I consider a disc rotating about its centre with some angular velocity and moving with linear velocity $v$, the kinetic energy of the centre of mass is $1/2 \ Mv^2$.

If we go into the centre of mass frame (which is similar to the case that we are sitting on the centre of mass and seeing the motion of all the particles of the disc), we see that nothing is moving, so the kinetic energy about the centre of mass is zero. But it is written in the books that it is $1/2 \ I \omega^2$. Why?

I think I am wrong about thinking about the centre of mass frame telling where I am (I'm just analysing the motion by sitting on the centre of mass so that I am rotating with it).

Also, tell me what are the expressions for the kinetic energy about centre of mass frame and the kinetic energy of centre of mass frame by correcting me.

Tell me if I am wrong anywhere and misunderstanding the concept. Please explain to me clearly.

Improve this question
$\endgroup$
1
  • $\begingroup$ Your first equation should be the sum of rotational and translational kinetic energy, it's not just $\frac12 M v^2$. And the center of mass frame does not mean you are rotating with the object and it's unclear why you think that is so. $\endgroup$
    – Triatticus
    Commented Oct 11 at 13:35

3 Answers 3

Reset to default
2
$\begingroup$

If we go into the centre of mass frame (which is similar to the case that we are sitting on the centre of mass and seeing the motion of all the particles of the disc), we see that nothing is moving,

That’s not correct. We see the particles in circular motion about the COM with a collective rotational kinetic energy of $\frac{1}{2}I_{com}\omega ^2$.

By definition the COM is a dimensionless point so it doesn’t rotate along with the particles around it.

Hope this helps.

Improve this answer
$\endgroup$
7
  • $\begingroup$ In my case consider two particles which are at different distances from centre now I am going to see one particle from the other one . I am consider myself rotating with that point i will see other particle to not have any angular velocity with respect to me but if i consider myself not rotating then i will find that the other particle rotating with same angular velocity as that of disc relative to me . And I asked a question regarding this that whether the relative angular velocity between the particles is 0 or not they told it is 0 . So I considered myself rotating $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:51
  • $\begingroup$ See the question I had asked on Physics Stack. physics.stackexchange.com/questions/830555/… $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:51
  • $\begingroup$ I am really confused to take that reference frame rotating or not please help me understand $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:54
  • $\begingroup$ @JyothiSrivalli the center of mass is a geometric point. A point does not rotate. You are not a point. You rotate $\endgroup$
    – Bob D
    Commented Oct 11 at 17:28
  • $\begingroup$ Yes I understand it . but see the other question i asked on physics stack exchange physics.stackexchange.com/questions/830555/… $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 17:31
2
$\begingroup$

Just to illustrate where the various formulae come from consider the following example. enter image description here

Two point masses, $m$, are rotating at angular speed $\omega$ clockwise about their centre of mass $C$ which is at a distance $r$ from each of them.
The centre of mass is moving at a speed $V$ to the left.

Noting that the speed of each of the masses relative to their centre of mass is $v=r\,\omega$, the kinetic energy of the system of two masses is $\frac 12 m(V-v)^2 + \frac 12 m(V+v)^2 = m\,V^2 + m\,v^2$.

Another way of evaluating the kinetic energy of the system is to all the rotational kinetic energy of the two masses in the frame of the centre of mass, $2\times \frac 12 I\,\omega^2= 2\times \frac 12 \,(2mr^2)\,\omega^2= m\,v^2$, and the kinetic energy of the centre of mass, $\frac 12 \,2m\ V^2 = m\,V^2$ to obtain the same result as before.

Here is a series of images for a more complex system (spanner) to show the translation of the centre of mass of the spanner (red dot) and the rotation of the spanner about its centre of mass.

enter image description here

Improve this answer
$\endgroup$
6
  • $\begingroup$ I am confused that the CM frame should taken rotating or without rotating and reason to take like that $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 15:54
  • $\begingroup$ Imagine that you are sitting at the centre of mass of the two masses. You will observe them rotating about you with an angular speed $\omega$. At the same time you and the centre of mass of the two masses are moving with speed $V$ to the left relative to the ground. $\endgroup$
    – Farcher
    Commented Oct 11 at 15:57
  • $\begingroup$ In my case consider two particles which are at different distances from centre now I am going to see one particle from the other one . I am consider myself rotating with that point i will see other particle to not have any angular velocity with respect to me but if i consider myself not rotating then i will find that the other particle rotating with same angular velocity as that of disc relative to me . And I asked a question regarding this that whether the relative angular velocity between the particles is 0 or not they told it is 0 . So I considered myself rotating $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:53
  • $\begingroup$ See the question I had asked on Physics Stack Exchange physics.stackexchange.com/questions/830555/… $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:54
  • $\begingroup$ I am really confused to take that reference frame rotating or not please help me understand $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:54
0
$\begingroup$

Firstly the total energy of a system is conserved in any non relativistic frame. Secondly kinetic energy of any system of particles is also conserved when measured from a inertial frame of reference. (Assuming no work is being done on the system.)

Now the problem of with what you had assumed was that when you spin along with the disk you are no longer in a inertial frame of reference hence the kinetic energy of the system will no longer be the same as before from your new frame of reference. What will be conserved here is the total energy of the system as a whole, the kinetic energy that you seemingly lost will now appear in the form of potential energy gained by the system due to the pseudo force that is the centrifugal force. So when we say to see things from the frame of reference of the axis or in your case the center of mass then we are not spinning along with the disc but rather just eliminated the effects of any translational motion from our frame of reference. Assuming it was non accelerated. So indeed you were wrong to assume that. The key point to remember here is that the frame of reference you pick must be inertial. So thats really it for your question.

The correct equations of kinetic energy is the same as what you wrote in the beginning of your question.

Improve this answer
$\endgroup$
5
  • $\begingroup$ In my case consider two particles which are at different distances from centre now I am going to see one particle from the other one . I am consider myself rotating with that point i will see other particle to not have any angular velocity with respect to me but if i consider myself not rotating then i will find that the other particle rotating with same angular velocity as that of disc relative to me . And I asked a question regarding this that whether the relative angular velocity between the particles is 0 or not they told it is 0 . So I considered myself rotating $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:45
  • $\begingroup$ See the question I had asked on Physics Stack Exchange physics.stackexchange.com/questions/830555/… $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:47
  • $\begingroup$ I am really confused to take that reference frame rotating or not please help me understand $\endgroup$
    – Jyothi Srivalli
    Commented Oct 11 at 16:48
  • $\begingroup$ Since your frame of reference is from one particles perspective then assuming that you don't spin the relative angular velocity of the particles should be the same as that from the centre. $\endgroup$
    – Ryan
    Commented Oct 12 at 12:17
  • $\begingroup$ However something interesting to note here is that the kinetic energy will no longer be the same $\endgroup$
    – Ryan
    Commented Oct 12 at 12:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.