Does a large uncertainty in a given value justify a large uncertainty in the result?

Question

I'm working on a pre-lab for my Physics 1 lab session, and I had a debate with the person I carpool with (who is taking the algebra-based Physics 1 lab). We seem to be unsure about uncertainties, and how they play out when doing calculations.

The given question was as follows:

When the falling mass is $0.250\text{ kg}$, a student obtains an acceleration from part 2.1 of $0.3±0.1\ \mathrm{m/s^2}$ and the radius of the shaft from part 2.2.1 of $0.015\text{ m}$.

a.) What is the expected angular acceleration as for part 2.2.2? The measured angular acceleration from part 2.4 is $16.1±0.3\ \mathrm{rad/s^2}$.

The given formulas were:

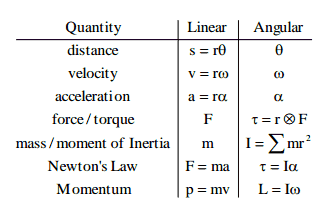
I used the acceleration formula, and came up with:

$$\begin{align} a &= \alpha r_\text{shaft} \\ (0.3\pm0.1) &= \alpha\cdot(0.015) \\ \frac{(0.3\pm0.1)}{(0.015)} &= \alpha \\ 13.33\;\mathrm{\frac{rad}{s^{2}}} &\leq \alpha \leq \; 26.67 \;\mathrm{\frac{rad}{s^{2}}} \\ \alpha &= 20.00 \pm 6.67 \;\mathrm{\frac{rad}{s^{2}}} \end{align}$$

My friend says that is incorrect (although I am fuzzy on his reasoning), but he mainly says you can immediately tell because the uncertainty is so high (30%+ from the value). My argument to that is, the original given uncertainty (±0.1) is 30% of the original value, so why can't the result's uncertainty be 30% of the calculated value?

My friend does make a good point, the uncertainty for the value is very high. But does the large uncertainty in the given acceleration make it okay for the final uncertainty to be that high?

Answer 1

When you divide numbers with uncertainties, the relative uncertainties of the two numbers add in quadrature (pdf). If one of the relative uncertainties is much lower than the other, than you can ignore it. Given the wording of the problem that you quote, it appears that you can treat the radius of the rod as having a negligible uncertainty. So your reasoning is correct; the relative uncertainty of the result will be exactly the same as the relative uncertainty of the input variable.

The general case is complicated, but your intuition is correct. In general, larger uncertainties in the inputs causes a larger uncertainty in the output. "Large" depends on the exact context, and sometimes some uncertainties simply do not matter.

Answer 2

In brief, input uncertainty does not in general lead to equal output uncertainty. How uncertainty translates from measured inputs to outputs is really a matter of what the transfer function is. For the simple problem you have you should be able to show how input errors translate to output ones. Achieving acceptable output uncertainty from input uncertainty is one of many of the challenges of designing a good experiment.