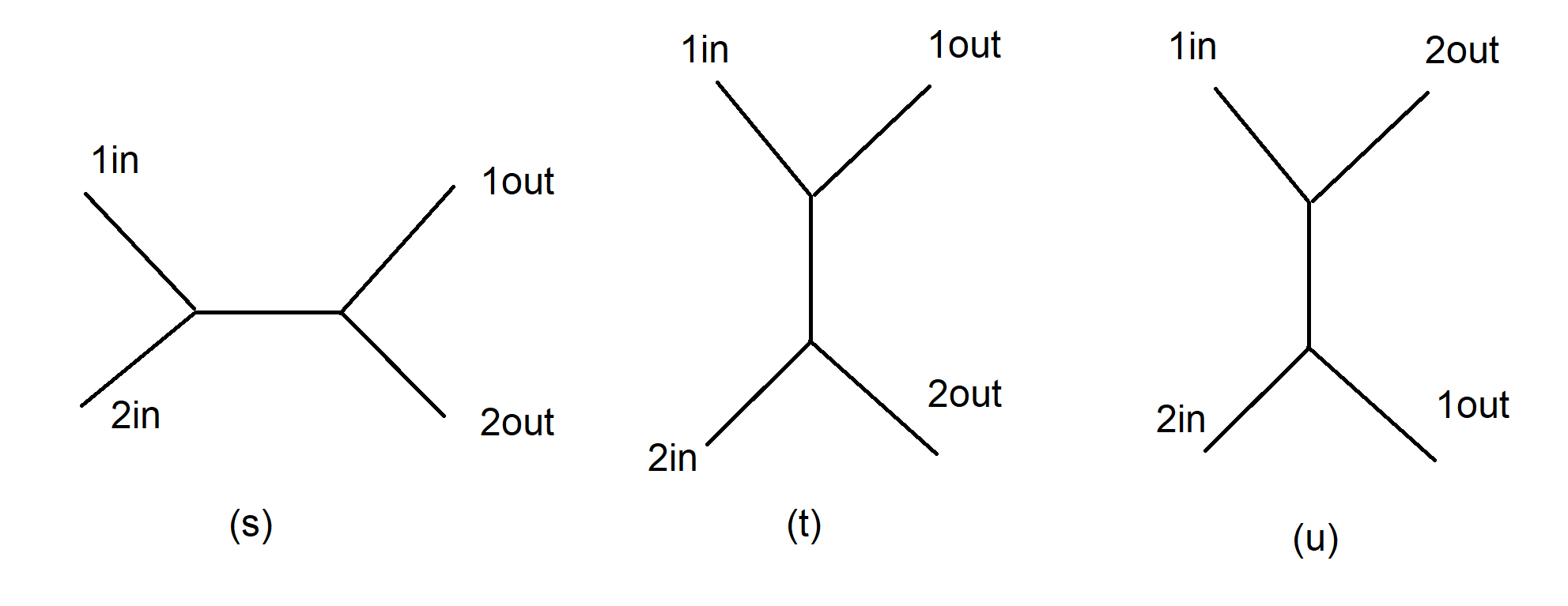
Considering the Yukawa interaction $-g\phi\bar{\psi}\psi$ and $e^{-}e^{-} \rightarrow e^{-}e^{-}$ scattering, the 2nd order term in the numerator of the gell man low formula is
$\frac{(-ig)^2}{2!}$ <0|$T\psi\psi\bar{\psi}\bar{\psi}\psi\bar{\psi}\phi\psi\bar{\psi}\phi$|0>.
In order to get the $t$-channel diagram for the process, we’d have to contract a $\psi$ from an incoming particle to one of the $\bar{\psi}$ from the first vertex. However we’d need to contract the other $\psi$ from the incoming particle to the first vertex (otherwise we’d get the u channel diagram). But the only other fields to contract with are $\phi$ and $\psi$, which give 0 if I’m correct. So how does the t-channel for electron-electron scattering in this theory exist?