How does Planck's Constant solve the ultraviolet catastrophe?

Question

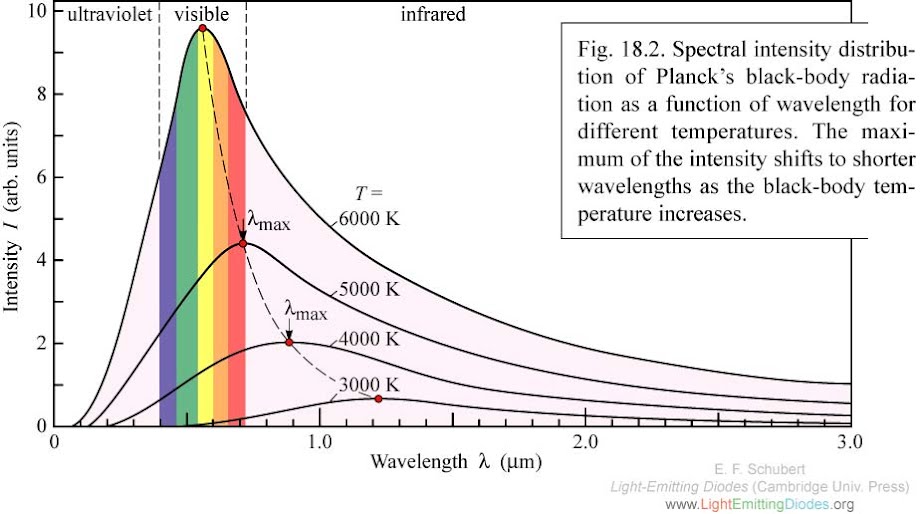
I am studying black body radiation and a high school level for a class. My understanding of it is this:

There is a finite frequency light and therefore amount of ultraviolet light objects can emit. That's when Max Planck made the assumption that "what if there was a limit to how small a wavelength can get.

However, I still get confused when I analyze a black body radiation graph. At the leftmost part of any curve (towards the origin), why is the intensity approaching zero? Is it because the molecules in whatever light can’t get enough quantized energy to lower is frequency?

Any help, especially in a simply-conveyed way would be great

Answer 1

The idea behind the Planck constant is that the energy $E$ carried away by radiation is quantized. Mathematically, the relation is

$$E = h \nu$$ where h is Planck's constant, and $\nu$ is the frequency of light. To connect this to your graph, we know that $\nu = c/\lambda$, so for a small $\lambda$, the corresponding $\nu$ would be very large.

Therefore, If we want to emit radiation in higher frequencies, we need large chunks of energy available at a given $\nu$, which becomes increasingly unlikely as $\nu$ increases.