How can we visualize electromagnetic radiation?

Question

In so many classes I’ve been told that light is nothing more than an electromagnetic wave. While this makes sense, it’s never been nearly as clear as imagining a static charge. At every point in space surrounding the static charge we can assign a vector representing the electric field. What about light? I know Maxwell's equations for $\rho=0$ give us something like $E=A\cos(kx-\omega t)$, but I feel there is something not being explicitly stated. It’s not like $x$ can assume any value, correct? I’d assume $x$ would be confined to the photon's trajectory. Otherwise, what does this equation even give us? It would imply that there’s an electric field present everywhere always. I’ve seen animations of electromagnetic waves orthogonal to each other on an axis, but I couldn’t imagine that the entire axis has an electric field, and also have trouble thinking about how the waves are shown in two dimensions.

So to quickly state my question, if we took snapshots of a photon and could see the electromagnetic wave at all points in space in each, what would we see? Would the field only exist at the singular point the photon resides at, would there be a field surrounding the photon (in the same way the electric field of a static charge does)? Does the field trail behind the photon as it travels? Etc.

If I’m not wrong, Maxwell's equations and the equation for electromagnetic radiation predated quantum theory, so how was this equation interpreted then, with the assumption the particle would always assume a definite position?

Answer 1

EM radiation in the range of visible light arises from transitions to lower energy levels of electrons around the atomic nucleus. This happens - for which Einstein was awarded the Nobel Prize - quantised. The quanta were later called photons. However, the spectrum of EM radiation is much larger and also includes nuclear processes (shorter-wave radiation). Long-wave radiation is also assigned to the EM spectrum, which produces EM waves through the joint periodic excitation of electrons in antennas.

The photons contained in all EM radiation travel forward at the speed of light until they encounter an obstacle (another electron). While mechanical waves (sound, water waves) require a medium, this is not necessary for photons and is even counterproductive.

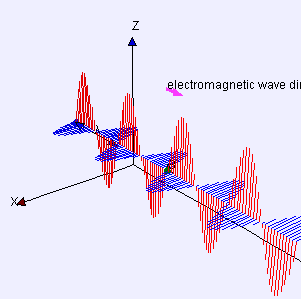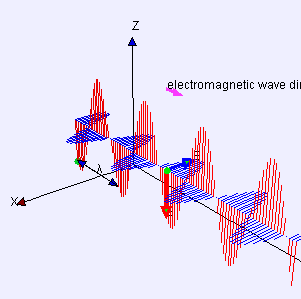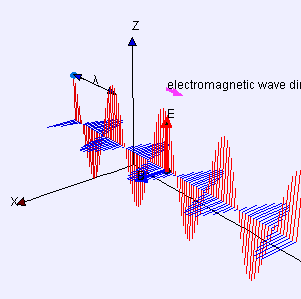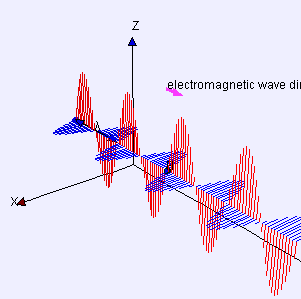
The periodic and synchronous acceleration of electrons in an antenna rod creates a radio wave (which is modulated and can thus transmit information to the receiver). Hertz generated and measured radio waves in the laboratory. He found that these waves have a magnetic field and an electric field component that periodically merge into each other.

Hertz did not know anything about photons. Today we know that the radio waves observed by Hertz consist of zillions of photons that are created by the excitation of electrons. While thermally excited radiation (which makes up the majority of the radiation surrounding us) produces photons chaotically, this happens for radio waves through the acceleration of electrons in the same direction. This creates a polarised field of photons whose total electric and magnetic field components are perpendicular to each other.

The macroscopic field components of the radio wave are the sum of the field components of the photons generating it.

In so many classes I’ve been told that light is nothing more than an electromagnetic wave.

This is not precise enough. Light is EM radiation. Search for the source of the radiation and you end up with the excitation of electrons. The filament in the light bulb emits photons because electrons are moved through the wire by a potential difference. Through multiple collisions at the atomic level, the electrons chaotically accelerate and by this chaotically radiate photons of different frequencies. No one can observe a wave from this, not to mention measure it directly. The only thing invoked is the phenomenon of wavelike intensity distribution of light behind slits. However, this represents a deduction and is not a direct measurement of the wave property as Hertz could measure it for radio waves.

So to quickly state my question, if we took snapshots of a photon and could see the EM wave at all points in space in each, what would we see?

The snapshots of a photon will show the periodic transition of its magnetic field component into its electric field component and back into its magnetic field component and so on. This will be the case for further photons in each spatial direction in which the source emits photons.

This is also true for radio waves. Only that here the photons are polarized (their electric field components are parallel to the antenna rod and their magnetic ones are perpendicular to it) and the number of emitted photons varies periodically (which is based on the fact that the wave generator must be operated with alternating voltage).

I apologize for this long answer, but I could not encompass the facets of the question in a shorter way.

Answer 2

The equation $E=Acos(kx-wt)$ describes a, plane wave, meaning one that extends infinitely in the $y$ and $z$ directions and propagates in the $x$ direction. And each plane of constant $x$ has an identical field value $\vec E$.

This equation is this a simplified model that never exists in practice, but it exists approximately if you restrict your inquiry to a small enough region – say, a 10 cm x 10 cm square (in the y and z directions) that is about 100 km (x direction) from a radio antenna source.

This is probably the best visualization of a classical EM wave

From Wiki

But keep in mind the red and blue peaks and troughs are not physically extended in space. They are showing the magnitude and direction of the field vector at a point on the x axis.