Trick question: why do dielectrics and isolated conductors affect capacitance differently?

Question

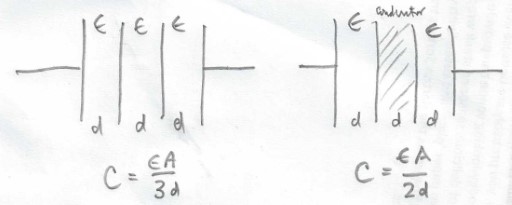
This question confused me a bit, but I figured out the answer. I just want to share this trick question here.

Trick question: Dielectric material produces net surface charge when placed in electric field. Isolated conductor also produces net surface charge when placed in electric field. However, dielectric increases capacitance when placed in-between plates of capacitor while isolated conductor seems to decreases capacitance. Why is that?

Increasing the dielectric constant allow the dielectric material to carry more surface charge that acts against the external field, which means that there needs to be more free charge on capacitor plates in order to maintain the same electric field and voltage difference. This would INCREASE capacitance. In contrast, having two capacitors in series (it's like an isolated conductor in-between), which will DECREASE the total capacity. Why one increases while the other decrease?

Answer 1

Answer to my own question:

Capacitor in series indeed have lower total capacitance than the individual component. However, adding an isolated conductor in-between two plates with fixed spacing will increase its capacitance.

When you put two capacitor in series, you are essentially doubling the spacing. Since C=permitivity*area/spacing the doubling of spacing will reduce total capacity. However, when you fix the distance between the two plates and add an isolated conductor in-between, you are effectively reducing the non-conducting spacing between the plates at the two end, so capacitance indeed increases (just like adding dielectric material).

The reason why people don't add isolated conductor in-between two plates to increase capacitance, is because when the spacing between two conductor is too small, there will be charge leakage.. and this is also why people need dielectric to further increase capacitance instead of keep shrinking the spacing, since dielectric's surface charge is bound charge that can't leak.

Answer 2

I think that the two situations you are comparing are illustrated in the diagram below?

Replacing some of the dielectric with a conductor increases the capacitance.
Whereas when a dielectric is placed in an electric field the charges on the surface of a dielectric reduce the electric field within the dielectric doing the same thing to a conductor results in the surface charges being such that the electric field inside the conductor is zero.

For static electric fields the permittivity of a conductor appears to be infinite. However ideal conductors cannot store charge between two sides so are no good as charge storing devices in a parallel plate type arrangement unless some insulting material is added (right-hand diagram) and then it is the properties of the insulating material which controls the capacitance.