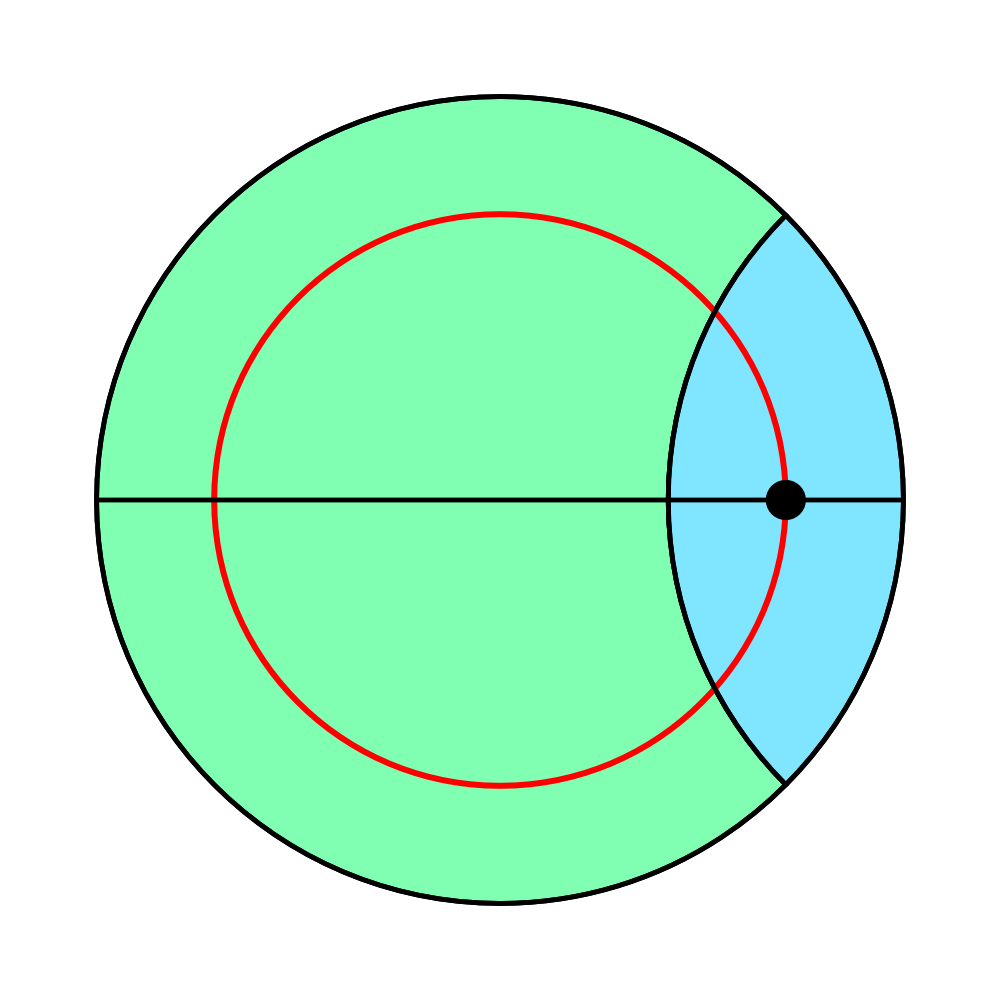
 Consider a uniformly dense perfectly spherical planet. At any point inside the planet, the gravitational attraction is only due to the mass below the point. (red circle) according to shell theorem.
Consider a uniformly dense perfectly spherical planet. At any point inside the planet, the gravitational attraction is only due to the mass below the point. (red circle) according to shell theorem.
Now onto the thought experiment, as per Newtons law of gravitation, every particle attracts every other particle with $\frac{G m_1 m_2}{r^2}$
By symmetry, the particles in the blue region should nullify their(net) attraction at the point. Similarly, all particles in the green region would have a component of the force along the -ve X-axis. Even the particles in the green region but not inside the red circle would have a small component of attraction along -ve X-axis at the point.(contradicting shell theorem) Again, their Y-axis components would nullify each other by symmetry. The same would hold true by spinning this cross-section along the X-axis to a 3d planet.
Now what's wrong with this explanation?
