How to understand this diagram, and is it relevant to the renormalizability of a theory?

Question

I'm having trouble understanding this diagram from my lecture note:

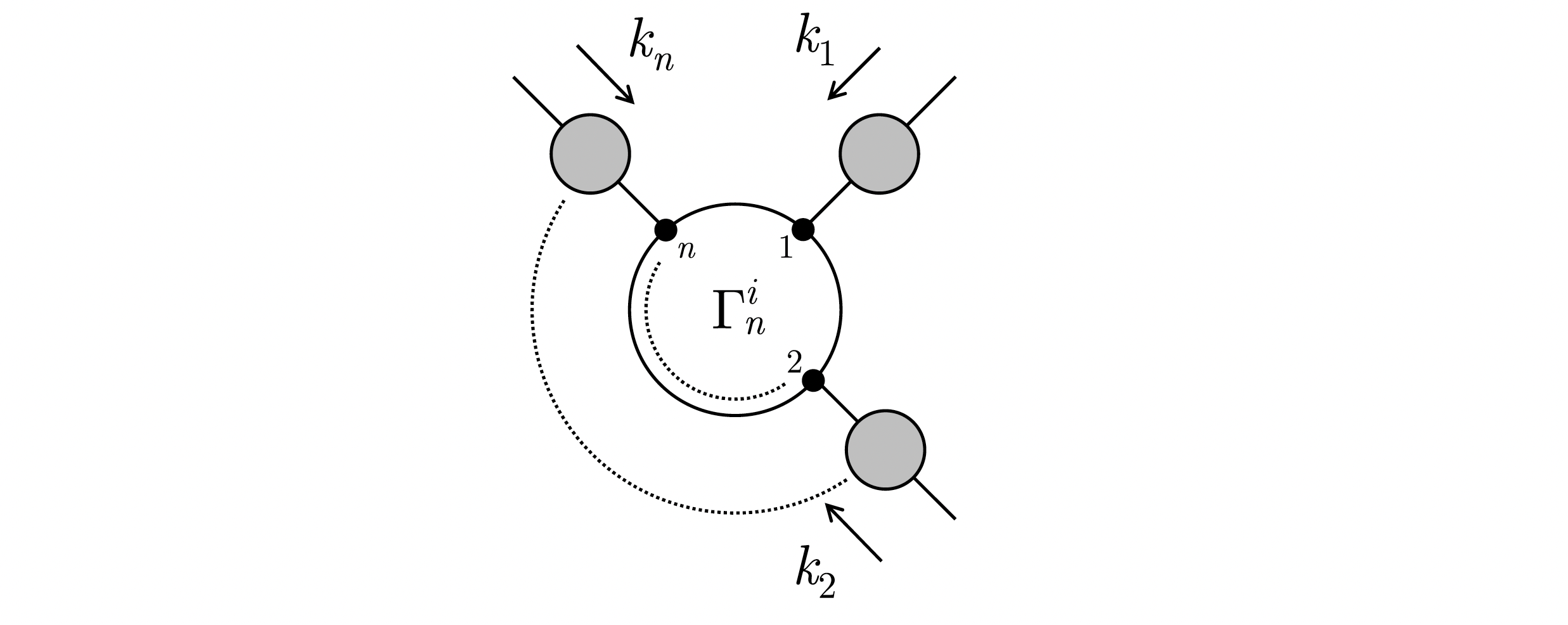
Each grey-shaded circle represents the diagrams for the two-point function $D(k)$. In equation, the diagram reads$$ \bar G_n(k_1,...,k_n) = \Gamma_n(k_1,...,k_n)\prod_{i=1}^nD(k_i)$$ However, I'm still unclear on how to understand this diagram and equation. Are we considering a scattering process involving $n$ particles?

Suppose we have a Lagrangian: $$ \mathcal{L} = \frac{1}{2}\partial_\mu\phi\partial^\mu\phi-\frac{1}{2}m^2\phi^2+\bar\psi(i\not\partial-M)\psi-g\phi\bar\psi\psi. $$ We can draw all the divergent Feynman diagrams using the superficial degree of divergence. Are those grey circles representing those divergent graphs, such as a 1-loop correction diagram? We can find that the Lagrangian also needs additional terms $\phi^4$ and their counterterms to be normalizable (which are not 2-point functions). Why don't we consider them in this diagram?

Answer 1

This diagram is meant to represent an $n$-point correlation function (or Green's function), $G^{(n)}$. It does not itself represent a scattering amplitude, but could be related to a scattering amplitude via the LSZ formula. It could also represent an internal part of a Feynman diagram for a more complicated amplitude.

This formula computes the correlation function by uses pieces derived from the quantum effective action, which is related to the generation functional by a Legendre transform. Going through the details of this is beyond the scope of a stack exchange answer, but is covered in many QFT texts.

The quantum effective action will involve an infinite number of terms with all powers of the fields and derivatives consistent with the symmetries. With schematic notation, \begin{equation} S_{\rm quantum} = \int d^4 x -\frac{Z}{2}(\partial \phi)^2 - \frac{m^2}{2} \phi^2 + \sum_{n_\phi, n_\partial} C_{n_\partial, n_\phi} \partial^{n_\partial} \phi^{n_\phi} \end{equation}

There are two components of this diagram. The first are the propagators with filled-in circles. These represent exact propagators and correspond to the factors $D(k)$ in the expression for $G^{(n)}$. The exact propagator can be derived from the quantum effective action by inverting the linearized equations of motion that follow from the quantum effective action.

The circle labeled $\Gamma^i_n$ represents an $n$-point vertex which follows from one of the terms in the quantum effective action with $n_\phi=n$.

Finally, while the expression for the Green's function is formally exact, in practice $D$ and $\Gamma$ (the propagator and vertex factor) can only be computed perturbatively.