10
$\begingroup$

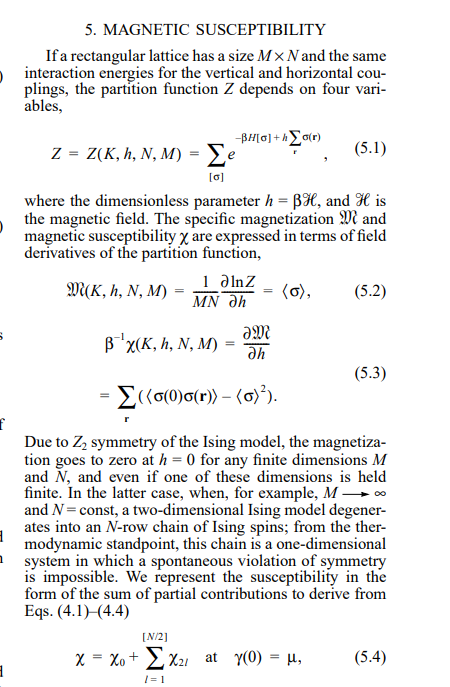
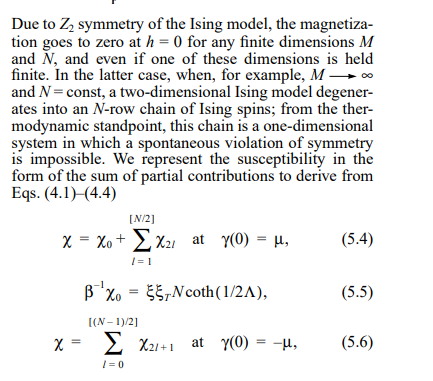
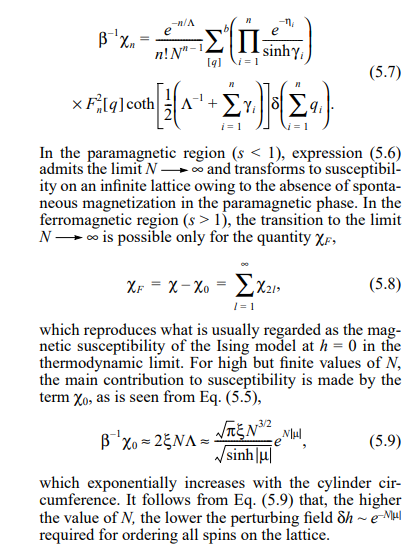
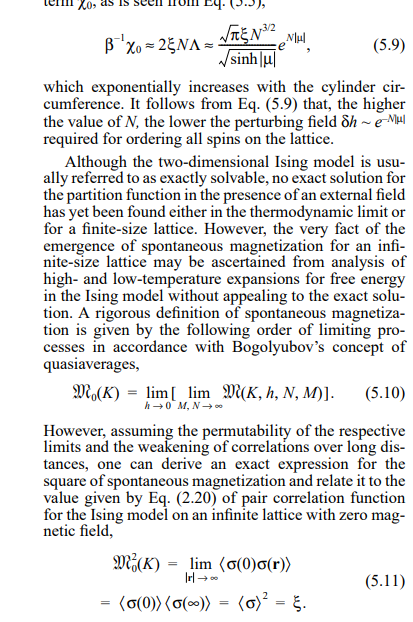
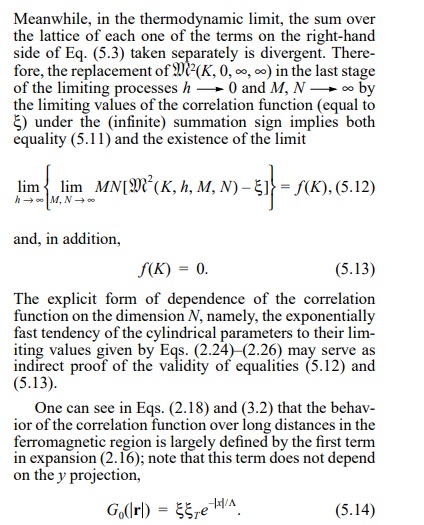
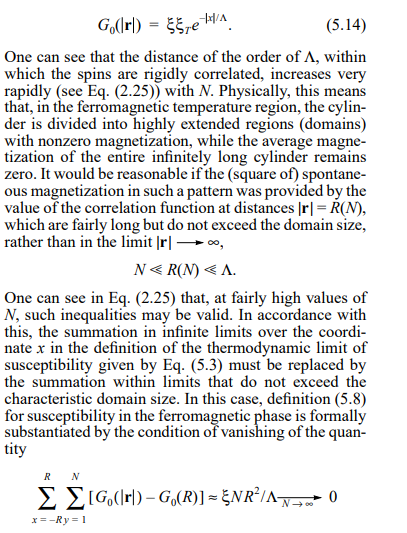
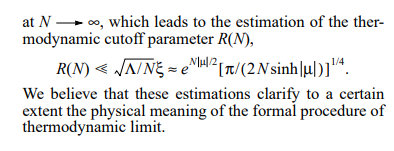
The Onsager solution for the 2-D Ising model allows us to find (among other things) complicated expressions for the internal energy of the system (in the thermodynamic limit and in zero magnetic field): $$ u \equiv \frac{U}{JN} = - \coth \frac{2}{t} \left\{ 1 + \frac{2}{\pi} \left[ 2 \tanh^2 \left( \frac{2}{t} \right) - 1 \right] K\!\left[4 \, \text{sech}^2 \left( \frac{2}{t} \right) \tanh^2 \left( \frac{2}{t} \right) \right] \right\} $$ where $t \equiv kT/J$ is the dimensionless temperature and $K(x)$ is a complete elliptic integral of the first kind. We can then (in principle) find a closed-form expression $C = \partial U/\partial T$.

Further, the net mean magnetization is known to be $$ m = \begin{cases} \left[ 1 - \text{csch}^4 (2/t) \right]^{1/8} & t < 2/\ln(1 + \sqrt{2}) \\ 0 & t > 2/\ln(1 + \sqrt{2}) \end{cases} $$ The question is then:

Is there a known closed-form expression for the magnetic susceptibility $\chi$ of the 2-D Ising model at zero field?

My (limited) intuition tells me that there should be, because energy and heat capacity are related to the first and second derivatives of the partition function with respect to $\beta$, and we have closed-form expressions for both of those quantities. Similarly, the magnetization and susceptibility are related to the first and second derivatives of the partition function with respect to the external field—but I have not been able to find a source that discusses a closed-form expression for $\chi$, only for $m$. Am I just looking at the wrong sources, or is there not actually a known expression for $\chi$ at zero field?

Improve this question
$\endgroup$
1
  • 3
    $\begingroup$ Quite interesting. I naively assumed that it should be quite simple to write down $\chi$. Suprpsingly, it is very complicated problem. It seems that some insights can be found in B. Nickel papers (doi.org/10.1088/0305-4470/32/21/303) $\endgroup$
    – Artem Alexandrov
    Commented Oct 11, 2022 at 20:31

2 Answers 2

Reset to default
7
+100
$\begingroup$

There are no explicit expressions, as far as I know, only expressions in the form of (complicated) infinite series, originating from expressing the magnetic susceptibility as a sum over 2-point correlation functions and using the exact expressions known for the latter. These have been used to analyze the remarkable analytic properties of the magnetic susceptibility.

The resulting expressions being very complicated, it seems pointless to reproduce them here. You can find them (together with links to the relevant literature) in McCoy's 2009 book; see Section 10.1.9 therein. You may also have a look at his article on scholarpedia.

In addition, a 2010 review of the history of this problem by some of its main investigators can be found here.

My (limited) intuition tells me that there should be, because energy and heat capacity are related to the first and second derivatives of the partition function with respect to β, and we have closed-form expressions for both of those quantities. Similarly, the magnetization and susceptibility are related to the first and second derivatives of the partition function with respect to the external field—but I have not been able to find a source that discusses a closed-form expression for χ, only for m.

Note that there are no known expressions for the free energy as a function of the magnetic field. This prevents the computation of the susceptibility by differentiating the free energy, which is the reason the available computations rely instead on correlation functions. (This is actually also the typical way the spontaneous magnetization is computed: $m^2=\lim_{n\to\infty} \langle \sigma_{(0,0)}\sigma_{(n,0)}\rangle$.)

Improve this answer
$\endgroup$
1
  • $\begingroup$ I have added a relatively recent (2010) review on the history of this problem, written by experts (since I am not very familiar with the literature on exact computations). It confirms that no closed-form solution was known as recently as 2010. I strongly doubt that this has changed in the meantime. $\endgroup$
    – Yvan Velenik
    Commented Oct 15, 2022 at 14:02
1
$\begingroup$

As far as I know, there is no closed form for the partition function in the presence of a field. Therefore there is no closed form for the susceptibility. The closest I know of can be found here. Below are some screenshots of the relevant part of the paper. If you cannot get your hands on it, give me your email, I will send it to you.

enter image description here enter image description here enter image description here enter image description here enter image description here enter image description here enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ My logic in thinking there should be a closed form for the susceptibility is that while we don't have $Z$ as a function of $B$, we can still find closed-form expressions for $M = \left. \frac{\partial(\ln Z)}{\partial B} \right|_{B = 0}$. This gave me hope that there might be some trick to get the susceptibility as well. As pointed out in the other answer, though, the result for $M$ is derived in a different way. $\endgroup$
    – Michael Seifert
    Commented Oct 21, 2022 at 17:23
  • $\begingroup$ I have added some screenshots of the paper. I think it contains what you are looking for. $\endgroup$
    – Shaktyai
    Commented Oct 22, 2022 at 9:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.