What is the intuitive interpretation of the transfer function of this system?

Question

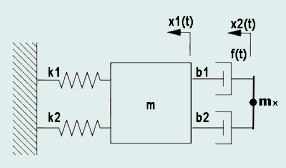
If I have the system that could be observed in the next Image:

I want to know the transfer function, where the external force $f$ is the entry and $x_1$ is the output. The direction and positive sense of movement and force is right to left, as the image shows.

Assume that the mass $m_x$ is zero, the initial conditions are zero, and the effects of gravity are null.
Moreover, the two springs are taken as a single equivalent ($k=k_1+k_2$) and the same with the shock absorbers ($b=b_1+b_2$), since it is assumed that each end of them suffers the same displacement, speed and acceleration.

My transfer function is:

$$\frac{X_1(s)}{F(s)}=\frac{\frac{1}{m}}{s^2+\frac{k}{m}}$$

It contains a null damping factor, that is, it is pure oscillatory. However, I know that I have a mass that acquires kinetic energy, a spring that acquires potential energy, and there will be an exchange of energy between them during the oscillations, but I also have a damper dissipating energy, which I don't see in my transfer function. How can I interpret this?

Attached is my development.

Answer 1

(Please write equations using MathJax; photos of equations aren’t generally acceptable. Handwritten equations with crossouts and slashes without context are especially hard to interpret.)

You’ve found that transfer functions don’t directly express energy dissipation in systems. Here, the dampers immediately assume a contraction or expansion speed proportional to the applied force; moreover, it’s assumed that they never reach a limit (e.g., bottom out). They also instantaneously translate that force to their other end. Disregarding the motion of the point of force application, it’s as if the force is directly applied to the mass via a rigid link. That’s why the dampers don’t appear in the transfer function.