A question On the deviation of the magnetic field in a solenoid using ampere's law and why we chose a specific ampereian loop

Question

When we derive the magnetic field in a solenoid using ampere's law, we usually consider an ampereian loop such that it's bisected by one side of the transverse section of the solenoid

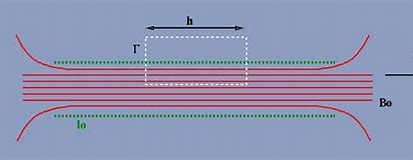
(like in the picture above)

I also understand from Question on Ampere's Law and Solenoid that we don't draw a loop that encloses the entire solenoid as ampere's law doesn't give us the magnetic field, but the line integral of the dot product of B and l.

however, I'm unable to understand why we don't consider two loops(each one enclosing the current on each side).such that these loops intersect at the center of the loop . I do understand that ampere's law is a line integral and the sum of the line integrals(when we add up the contributions of both loops is zero). however, I'm unable to understand why if the line integral each loop , taken individually gives us the magnetic field, why doesn't the sum of both loops give us the same?

Moreover, leaving ampere's law aside, as we're only considering half the current passing via the solenoid, shouldn't the field be 2μnI

where μ is the permeability of free space, n is the number of turns per unit length and I is the current passing through the circuit.

Answer 1

In order to apply Ampère's law, we use a single loop. Ampère's law tells us that the line integral of the magnetic field around that single loop is proportional to the current that passes through that loop. Ampère's law will hold regardless of the size of the loop. It will hold even though there might be other currents in the system that are not enclosed by the loop.

For this reason, when you have a loop that is only on one side of the solenoid's axis, as in the diagram, and you apply Ampère's law, it is possible to deduce the total magnetic field along the axis of the solenoid—not merely the magnetic field sourced by one half of the solenoid.

It seems that you find this a bit counterintuitive. Why is it that Ampère's law can tell you something about the total magnetic field around the loop, even though there are contributions to the magnetic field from currents that don't pass through the loop? The answer is that if there is any current that doesn't pass through the loop, then its contributions to the magnetic field will cancel out of Ampère's law, in the sense that their line integral around the loop will be zero. Only currents that pass through the loop contribute a nonzero amount to this integral.

If you decide to add in a second loop, you must apply Ampère's law individually to each loop. And in both cases, you will just get the same result for the total magnetic field along the axis of the solenoid, which shows that applying Ampère's law to the the second loop does not provide any additional information compared with the first loop.

You do not use two loops and then add up the magnetic fields that you deduced from applying Ampère's law to the two loops individually. The result of that would be twice the actual magnetic field, because each individual application of Ampère's law already tells you information about the total magnetic field.