2
$\begingroup$

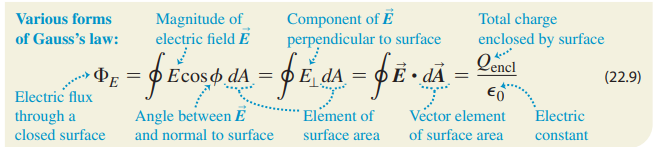
How do I prove that Gauss's Law works and is valid for a sphere with a circular disk on top?

I'm given the radius of the sphere R$(\theta_0,\pi)$, radius of the disc $(R \ sin \ \theta_0)$, the center of the circular disc:

$$z=z_{0}=R \ sin \ \theta _0$$

and the differential area element for both the sphere ($e_{r}R \ sin \ \theta _{0}$) and the circular disc ($e_{z} s \ ds d\phi$).

Where:

$$s = \sqrt{r^{2}-z^{2}} \ \ \ \ \ for \ \ \ \ s(0, e_{r}R \ sin \ \theta _{0})$$

With $\phi(0,2\pi)$ and $e_{r}e_{z}=cos \ \theta$.

So far, I have only placed the integral equations regarding Gauss's Law:

enter image description here

I also consulted other resources and they said that the equation for the sphere should be utilized, so that everything is simplified. I also saw sources using the steradian method, however, my professor strictly wants me to use the differentials provided. I'm at a dead end, because most sources together with my lectures only cover examples using symmetric figure.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Use the infinitesimal expression to show that the components of integral of the flat cap (Notice that on the flat cap it was not "perpendicular outwards") was the same as the components on the sphere if extended, i.e. the infinitesimal integral of the "flat cap" surface and the and the curved sphere surface, at given $d\theta$. (Technically you could just make it a sphere, and since the empty area had no charge. etc. But the question was to prove the Gauss's law) $\endgroup$
    – ShoutOutAndCalculate
    Commented Jul 3, 2022 at 14:55
  • $\begingroup$ Have you considered solid angle? The concept of solid angle is so powerful that it can directly prove Gauss' law, so it will be easy to use solid angle for this relatively simple surface. See here: 1. physics.stackexchange.com/questions/646752/… 2. socratic.org/questions/… 3. ldeo.columbia.edu/~richards/webpage_rev_Jan06/… $\endgroup$
    – Andy Chen
    Commented Jul 3, 2022 at 17:33

0

Reset to default