2
$\begingroup$

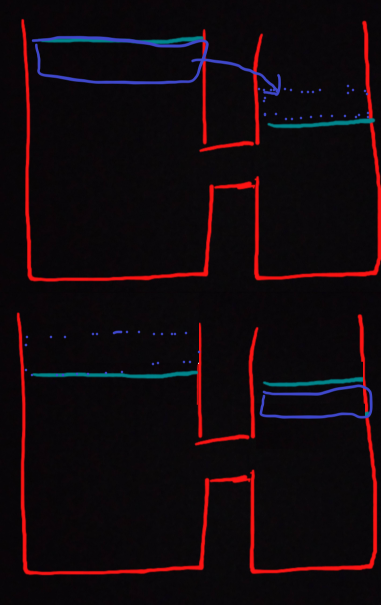
enter image description here

Imagine combining 2 water tanks (with equal cross section areas) with different water levels.

I'll call them A (tank with the higher water level) & B.

When water is flowing from A to B, what happens to its potential energy? Does it decrease? If so, what happens to that energy?

I would also like to know what happens to the center of gravity of this whole water volume? Does it also lower when water is flowing?

Edit: I'll tell what I am thinking. In A, some amount of water is going down. Hence the potential energy decreases. And in B the same amount of water is pushed up the same hight. So the potential energy increases. So as the mass and the change of hight is equal the decrease and increase of potential energy is also equal. Doesn't that mean the net change of potential energy is zero?

I have seen so many explanations similar to the answers below. And that seems correct. But still I can't get my head off from the above explanation. Can anyone show me what is wrong in my explanation?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ The question about the center of gravity is easily answered using Newton's laws. $\endgroup$
    – NDewolf
    Commented May 23, 2022 at 11:42
  • $\begingroup$ In order for the water levels to be different, the opening between the tanks needs to be closed. $\endgroup$
    – Bob D
    Commented May 23, 2022 at 11:56
  • 2
    $\begingroup$ If there were no friction, the system could do oscillations infinitely with transforming gravitational potential energy into kinetic energy of liquid flow and reverse. But due to friction part of energy will be lost in the end, when both levels become steady. $\endgroup$
    – Ivan Kaznacheyeu
    Commented May 23, 2022 at 12:17
  • $\begingroup$ @BobD I know. Just forget about those practical problems. I meant they are separated by telling that I'm combining them. Sorry, if I created any confusion. $\endgroup$
    – Binuka Perera
    Commented May 23, 2022 at 14:00

2 Answers 2

Reset to default
2
$\begingroup$

Looking at the following image, it's pretty obvious that the centre of mass will be lower, because the final state will be, just taking a portion of water (blue rectangle) and lowering it.

And obviously, the potential energy also is reduced (lowering mass centre, lowers total potential), in an ideal world the water would just oscillate, going up and down in both tanks, but in reality, the kinetic energy of the water flowing will disperse very quick through friction and heat.

enter image description here

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ Yes. I understand what you are telling. I can understand that it is correct. But I have another idea. In A, some amount of water is going down. Hence the potential energy decreases. And in B the same amount of water is pushed up the same hight. So the potential energy increases. So as the mass and the change of hight is equal the decrease and increase of potential energy is also equal. Doesn't that mean the net change of potential energy is zero ? $\endgroup$
    – Binuka Perera
    Commented Jun 1, 2022 at 17:22
  • $\begingroup$ Ohh yes, I see what confuses you, seems a bit counter intuitive doesn't it? Hehe. Well, the thing is that if you really imagine a droplet (a small square if you want) of water going from up top of the 1st container passing through the pipe, to the top of the 2n container, you see it actually goes a lot of way down and then only a bit up. $\endgroup$
    – Guillermo Abad Lopéz
    Commented Jun 2, 2022 at 18:13
  • $\begingroup$ In another way that I think focuses more on your point of view, if you really think of it as a square of water that goes down only to next layer. And in the opposite tank a square of water that comes up from the layer under, they seem they should increase the same energy. But that is not a correct way of thinking it. When that square goes down to the next layer, there was water there already. So you also push that down and that pushes the next, and so on until you reach the pipe when the same proces occurs from botton to top. $\endgroup$
    – Guillermo Abad Lopéz
    Commented Jun 2, 2022 at 18:22
  • $\begingroup$ And there, you see each process transmits the same potential energy, but in the higher one, you have to do the process more times, you have more layer to keep pushing squares, while in the 2nd tank you have less layers then to takenthe square to the top, and the quantity of times you have to do this discreete process innthe limit to continous is proportional to the height. As you would really need to imagine younare moving actually the hole column under that droplet to the pipe and then a hole colum comes up in the other tank. If you think about it, this is related to what @Luke Pritchett said $\endgroup$
    – Guillermo Abad Lopéz
    Commented Jun 2, 2022 at 18:24
  • 1
    $\begingroup$ It is exactly what I wanted. I realized what I got wrong. Thank you $\endgroup$
    – Binuka Perera
    Commented Jun 7, 2022 at 12:55
0
$\begingroup$

You have made two incorrect assumptions:

  1. You assumed that the height changes by the same amount in each tank. That is incorrect. It is only correct when the areas of the two tanks are equal. In general the volume of each tank changes by the same amount, not the height
  2. You assumed that equal changes in height lead to equal changes in potential energy. That is also incorrect.

The potential energy in a tank of water is proportional to the height of the water squared. That's because the height of the center of mass of the water is $h/2$ and the mass of the water is $\rho A h$, so the potential energy is $\frac{1}{2} \rho A g h^2$. Because the potential energy has this non-linear relationship, equal changes in height don't lead to equal changes in energy. Just calculate

$$(h_1 +\Delta h)^2 - h_1^2 = \Delta h^2+ 2\Delta h h_1$$

See how the change in the square of the height depends on the initial height $h_1$ as well as the change in height? That means the two tanks can change their potential energies by different amounts and hence the total change in potential energy can be non-zero.

Improve this answer
$\endgroup$
1
  • $\begingroup$ 1. I have clearly stated that the cross section areas are the same. ( I did it to make the explanations much simpler. ) 2. I took the fluid as few pieces, not as a whole body. So that's why I got that confusion. I have added my initial thought and what went wrong as an answer. Now I understand where I got wrong. Thanks for helping. $\endgroup$
    – Binuka Perera
    Commented Jun 2, 2022 at 7:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.