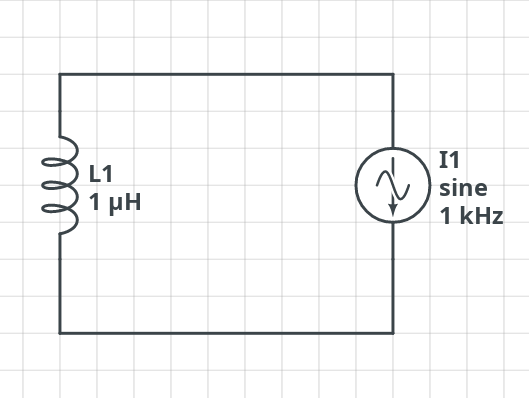
When we talk about lumped circuit model, I get confused about the terminology that is generally used. For a loop, we do know that the total flux through the loop can be written as $\Phi = \Phi_{ext} + L_sI$, where $\Phi$ is the total flux through the loop, $\Phi_{ext}$ is the flux generated by external sources and $I$ is the current through the loop. My question is whether $L_s$ is the geometric self-inductance of the loop or the summation of the geometric self-inductance of the loop plus the inductor branch?
Consider this example shown in the figure, let us start with the lumped circuit model. This implies that the the equation $\oint\bar{E}.\bar{dl}=0$ is valid. Assuming that $L_s$ in the above equation is the total inductance then (assuming there are no external sources for flux), the equation becomes
$$ \Phi = L_1I_1. $$
If the current source is time dependent with the equation $I_1 = I_0 \sin(\omega t)$ (here $\omega$ is considered to be 'slow' so that the dimensions of the circuit are much smaller than the associated wavelength of the EMWs, maybe like $10 Hz$), then the total flux is changing, which implies that from Faraday's law
$$ \oint \bar{E}.\bar{dl} = -\frac{d\Phi}{dt} = -L_1 I_0 \omega \cos(\omega t). $$ But, this violates the lumped circuit model we started with according to which $\oint \bar{E}.\bar{dl} = 0$. Thus, to save the lumped element model the initial assumption that the $L_s$ in the equation $\Phi = \Phi_{ext} + L_sI$ is the total inductance is wrong. Then what is that $L_s$? I was assuming that the $L_s$ is the geometric inductance of the entire loop excluding the branches. Is that correct?