0
$\begingroup$

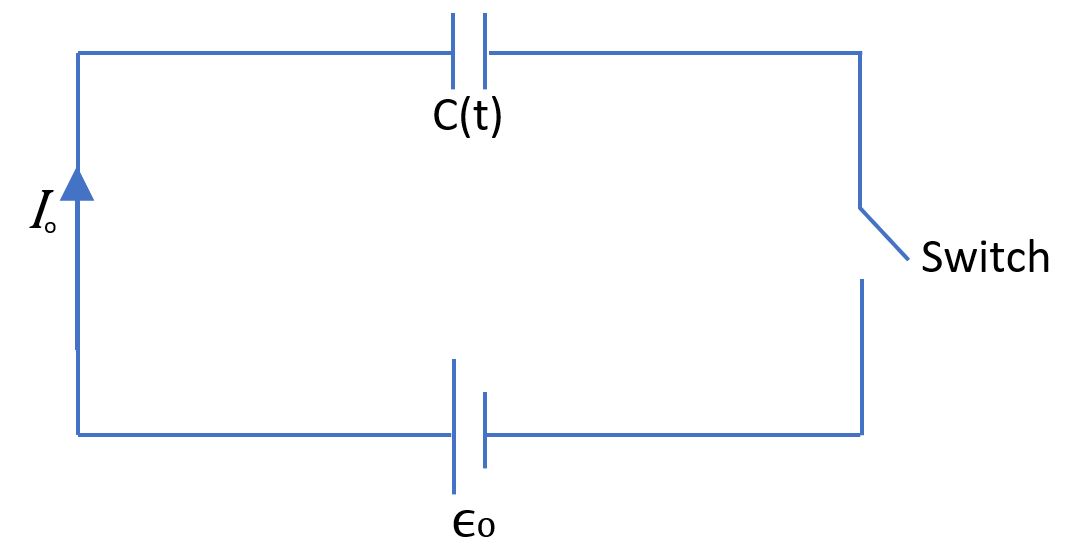
The simple circuit below, consists of a battery with constant electromotive force (efm), $\epsilon_0$, a capacitor which capacity varies with time $C(t)$ and ,to make it simple, ideal wires (zero resistance).

When the switch is closed, the capacity of the capacitor changes with time in a way to maintain current $I_o\;$ constant.

As shown below, the stored energy power in the capacitor is half of the discharge energy of the battery (which I will prove it below). What happens to the other half? enter image description here

Battery discharge power at any time: $P_{battery}=\epsilon_oI_o$

Stored energy in the capacitor: $U_{stored}=\frac{1}{2}C(t)\; \epsilon_o^2\;$. So, by diffentiating with regards to $t$, we obtain the stored power in the capacitor: $P_{stored}=\frac{1}{2}\; \frac{dC(t)}{dt}\; \epsilon_o^2\;\;$ (eq.1).

On the other hand, for the capacitor: $q=C(t) \;\epsilon_o$, by differentiating with respect to $t$, it gives $I_o= \frac{dC(t)}{dt}\;\epsilon_o\;\;\;$(eq.2).

By substituting $\frac{dC(t)}{dt}$ from (eq.2) in (eq.1), we have: $P_{stored}=\frac{1}{2} \epsilon_oI_o\;$

So, $P_{stored}=\frac{1}{2}P_{battery}$. Where has the other half of $P_{battery}\;$ gone?

Note: This question is not duplicate of A problem of missing energy when charging a second capacitor because in that question, energy is lost due to radiation damping of oscillating charges or other ways, while in my question here that is not the case, if you add a resistance to this circuit, it still can be shown the energy lost in the resistance plus the energy stored in the capacitor is half of the discharge energy of the battery.

Improve this question
$\endgroup$
7
  • 3
    $\begingroup$ Hint: how much work is extracted from the capacitor by the mechanism that changes its capacitance? $\endgroup$
    – Ján Lalinský
    Commented Apr 21, 2022 at 20:02
  • $\begingroup$ When the switch is closed, the current in the circuit is NOT constant. $\endgroup$
    – David White
    Commented Apr 21, 2022 at 20:13
  • 1
    $\begingroup$ physics.stackexchange.com/questions/187774/… $\endgroup$
    – BowlOfRed
    Commented Apr 21, 2022 at 20:17
  • $\begingroup$ Does this answer your question? A problem of missing energy when charging a second capacitor $\endgroup$
    – Solomon Slow
    Commented Apr 22, 2022 at 12:23
  • $\begingroup$ @SolomonSlow no, that is a different concept. In the question you refered to, the energy is actually wasted due to the loss. In this question here, as Ján Lalinský pointed, the missing energy is used to resist against the change of the capacity of the capacitor. $\endgroup$
    – Ebi
    Commented Apr 22, 2022 at 12:28

1 Answer 1

Reset to default
0
$\begingroup$

The formula that you used for the energy on a capacitor, (1/2)C$V^2$, is based on the fact that when you are charging a (fixed) capacitor, the voltage on the capacitor starts at zero and goes up as the capacitor is charged. That is not true in the situation you describe. (Note: the $ε_o$ is normally used to symbolize something other than voltage.)

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.