I can only think of this particular diagram, though there must be more as I believe the amplitude is supposed to be equal to 0, as it is used to highlight renormalisation in QED.
Which other processes are possible up to order $e^{2}$ which are connected, amputated 1PI diagrams?
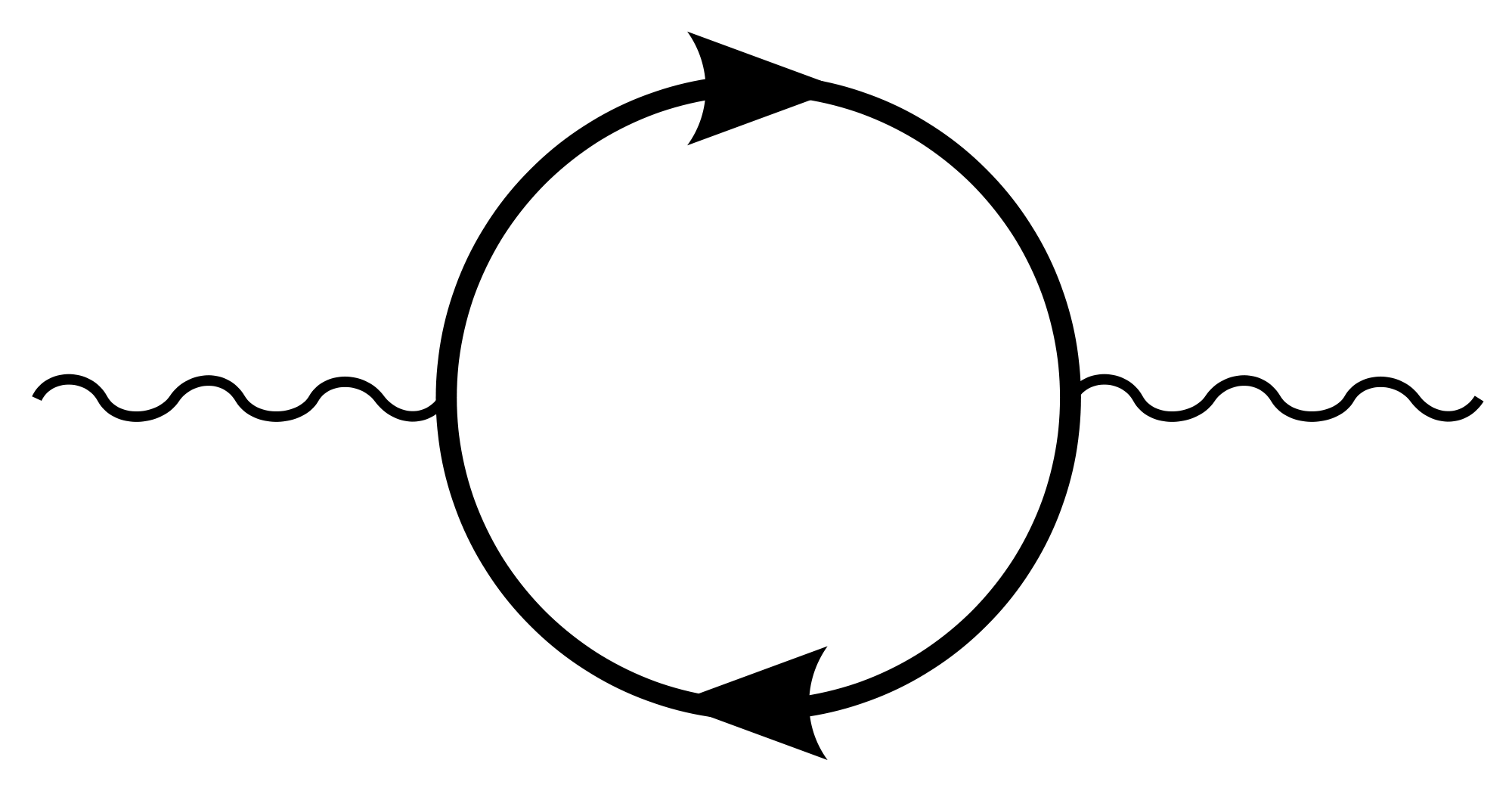
Apart from the propagator (which is order $e^0$), this is the only one. Notice that at order $e^2$ you can only use two vertices. Furthermore, you already have two photons on the outer legs. The only possibilities to include vertices with two outer photons is for each of these photons to be part of a vertex, and once we used the two possible vertices we can only connect the remaining legs.
As you noticed, the amplitude does not vanish. In fact, it diverges. That is pretty much one of the points of renormalization: the fact that this diagram does not vanish will lead to important physical predictions which can be experimentally tested. This particular diagram is often referred to as the vacuum polarization diagram and it allows us to understand how the vacuum does have polarization effects once quantum corrections are taken into account, similar to how polarization affects the propagation of electromagnetic phenomena on dielectrics, for example.
