What is the significance of Power factor?

Question

I was studying the power in Alternating current. The formula to calculate the power in alternating circuit is $$P = VI \cos{\Phi} $$

where, $V$ and $I$ are RMS values of voltage and current and $\Phi$ is the phase difference between voltage and current. Let's take a simple AC circuit connected to a resistor (or any appliance).

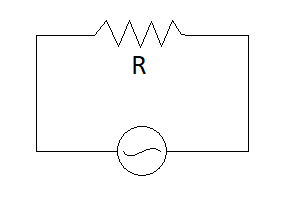
Here The power through the resistor is $$P = VI$$

as the impedance is equal to $R$ and the value of power factor is 1.
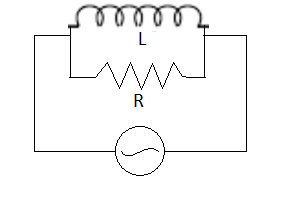
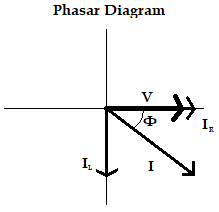
But, if we connect an inductor in parallel, the value of power factor will decrease due to increase in $\Phi$. and the current will increase (Overall, Power will remain constant).

Does that mean that we can reduce power factor to get more current? I'm confused about this. Is there also any value of power factor?

Answer 1

Your formula for power, $P = VI \cos{\Phi}$, gives the average (over a period) rate at which electrical energy is converted into heat.

The difference between a resistor and an inductor is that over a whole period a resistor is taking electrical energy from a voltage source and converting it into heat whereas for a quarter of a period an inductor is taking electrical energy from a voltage source and converting it into stored energy in a magnetic field and then for the next quarter cycle the inductor is giving back the energy to the voltage source as its magnetic field (stored energy) is reducing so on average over half a period there is no net transfer of energy between voltage source and inductor.

In terms of averages over a period, $\langle P\rangle_{\rm resistor, period} = V_{\rm RMS}I_{\rm RMS}$ and $\langle P\rangle_{\rm inductor, period} = 0$

So in this situation $P=V\times I\cos \Phi = V I_{\rm R}$ as $V$ and $I_{\rm R}$ are in phase with one another, and the $I_{\rm L}$ plays no part in the average power dissipation as $V$ and $I_{\rm L}$ are $\pi/2$ out of phase.

So power is only dissipated if there is a projection of current onto the $V$ axis.

Answer 2

Does that mean, that we can reduce power factor to get more current?

Yes, you will get more current for the components in parallel, it's similar to resistances in parallel, the overall impedance is reduced.

Is there also any value of power factor?

The power factor will be between 0 and 1 for the circuit shown.

Answer 3

Here's how you can get a feel of power factor in an ac circuit.....

In a circuit when a current 'I' flows across two points having potential differences 'V' between them the electric potential energy change of the charges flowing per unit time is 'VI' now we know that total energy remains conserved so this energy change leads to energy in another form (for example in a resistor we get this energy change in electric energy as heat and in an ideal inductor it takes form of magnetic field energy).

Now in a sinusoidal circuit both V and I changes sinusoidally. Now to have more and more power output ( i.e. another form of energy that we have discussed above) we must have more and more value of 'VI' thus if V and I both attain their maximum values together ( or we can say they are inphase) we will get high 'VI'.

So the power factor represents mutually how voltage and current are changing since they both contribute to the power output. If they both compliment each other we will get high output but if they do not go well with each other then power output will be low.

It is like when you push a child on the swing you pushes in sync with the motion of swing to increase the amplitude and if you disturb this sync it slows down. It is this sync that power factor represents.