I have the following problem:
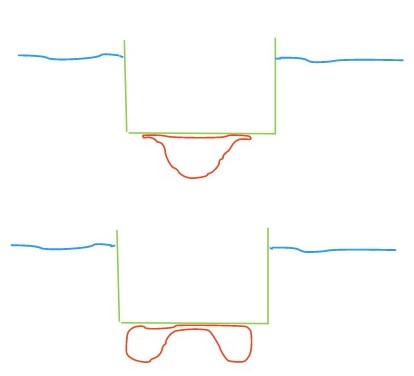
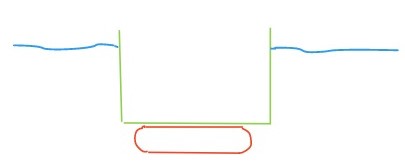
An inflatable beach ball is made of a thin, inelastic, but bendable material. When fully inflated, it has a radius $R$. In an experiment, the ball is filled half full with air, and is then continuously pushed underwater by a large, flat surface. What shape does the ball assume when fully underwater? If possible, determine the numerical values of the relevant sizes of the shape.
My initial guess to this problem was that the shape is going to be a hemisphere of radius $R$. I tried integrating the water's pressure's vertical component on the surface, and equating it with the force exerted by the flat surface on the ball. Then dividing this force by the area gives the inner pressure of the air, which turns out is always larger than or equal to the pressure of the water. This means the ball would have to exert pressure on the air, which seems to make sense, since if it's a hemisphere, it's fully streched.
However, this doesn't prove at all that the shape is indeed going to be a hemisphere, and I don't even know if that's the correct answer to the problem.