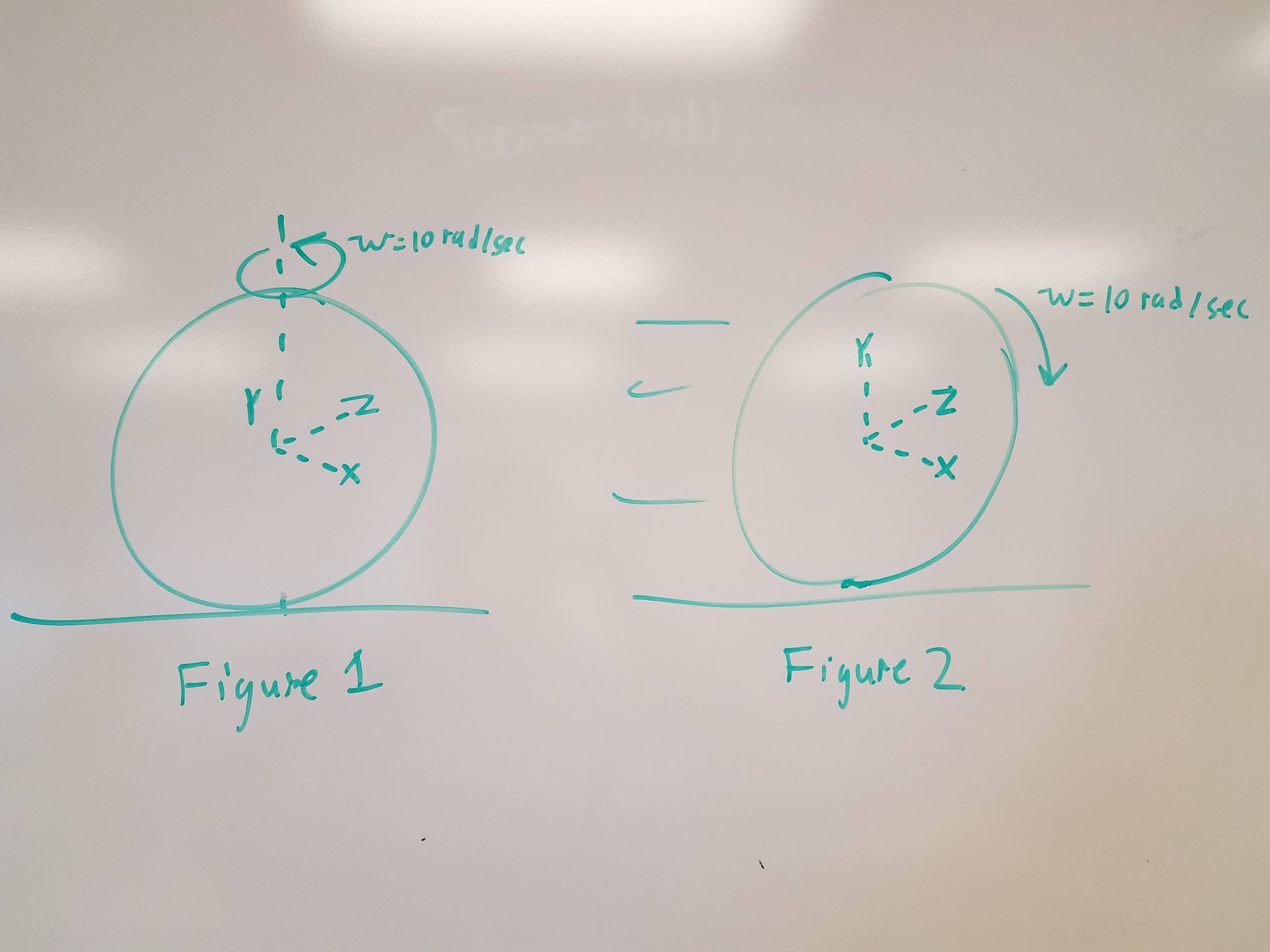
I just thought of a mind-boggling question about angular velocity and friction.
Here is the picture:
The first figure shows a ball spinning around the y-axis, and the seconds shows another ball that rotates about the z-axis.
In which figure would the ball spin the longest until its angular velocity will be zero radians/second?
The force of friction and gravity, the mass and the radius of the balls, and the initial angular velocity for both scenarios are the same. Both balls are on a flat surface. Assume air resistance is negligible. Assume the ball maintains a solid shape at all times.
Many of my friends say that it's the second figure. My physics teacher and I say they will stop in the same time. Who has right?