Why does something round roll down faster than something square?

Question

Why does something round roll down faster than something square?

That's a question given to me by my five year old son.

^{So let's not get into detailed discussion about what is 'square' and what is 'round' or what is 'rolling down'.}

I thought that this might be interesting enough to ask here.

The question relates to the simple observation that 'round' objects roll down a 'hill' or 'slope' very fast.

Can we say something insightfull or intuitive about this? For instance, I can imagine that different objects might roll down faster on a straight slope, or different objects do better on a slope with bumps. What makes an object roll/fall down fast?

Answer 1

If I was answering a 5 year old, I would probably say something like this:

Because the corners get in the way, but round things don't have corners.

Also, this example comes to mind:

When you lie down on a hill and roll down, if you stick out your elbows away from your body, they will get in the way (hit the ground) and slow you down.

Answer 2

There's an interesting subtlety here - the difference between rolling fast and rolling at all.

The second question is easier. For a polygon to start rolling down the hill, we need only one thing:

$\theta > \phi$, i.e. the object's center of mass (think of it as the "middle" of an object) is past the furthest corner of the polygon.

Take an assembly of shapes on a hill:

In this situation, the square is stable, the circle is unstable and will roll, while the octagon is just on the verge of moving. In general, an $n$-sided regular polygon has $\phi_n = \frac{180^\circ}{n}$ - as $n\to\infty, \phi_n \to 0$, i.e. by adding more sides and making the shape rounder, you decrease the angle needed to get it moving.

EDIT: a comment pointed out that the first condition was not needed. Even if the static friction is weak and the square begins sliding, it still will not roll unless the center of mass (black dot) is to the right of the support point (black line).

However, this analysis assumed that the shape started at rest, flat side down. If the octagon were given a push to get it started, it would also roll, but in a jagged "bumping" motion that would dissipate kinetic energy with each corner impact. This is the more difficult, first question, "Once rolling, which one accelerates faster?", which was already thoroughly answered in the linked question.

Answer 3

Something will roll down a slope when its centre of gravity is not directly above a part of it in contact with the ground. With a circle on an even slope, that is always true, so the circular object will always roll.

A square object will slide down a slope, but it will topple if the slope is greater than 45 degrees and if it is prevented from sliding (eg by friction or by encountering a small obstruction.

Answer 4

Because the square spends time converting kinetic energy to potential (and vice versa), and the circle doesn't.

Consider a perfectly elastic square rolling along a frictionless horizontal surface with horizontal and angular momentum (so it has no way to shed its energy and stop moving). Think about the square in this position:

Think about how it can "hover" at this spot for a long time if it has just barely enough leftover kinetic energy to topple it forward and keep it moving. Most of the energy of its movement has converted to potential energy, and as it falls it will get converted back to kinetic energy, mostly in the form of angular momentum.

That energy will carry it back up to that same point, where it again hovers and just barely makes it over the hill. As you increase the starting energy, getting over this hill becomes easier and easier and the rolling speed approaches the circle's. On the other hand, you can decrease the energy to add more and more "barely"s to just barely making it over the hill, and make it hover at the top of each hill arbitrarily long.

A circle doesn't spend any time going up and down this "potential energy hill"; its total energy just stays in the form of kinetic energy the whole time.

Answer 5

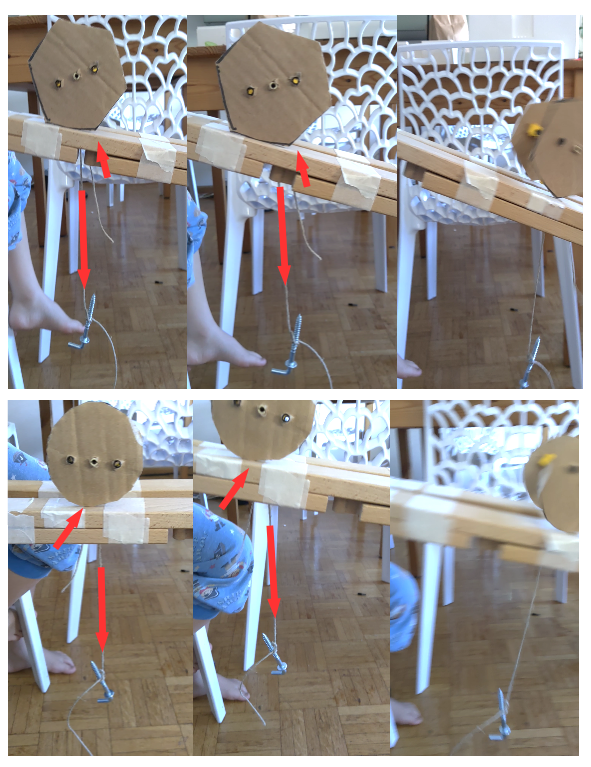
Based on some tips we have done some experiments

Experiment 1, the tipping over

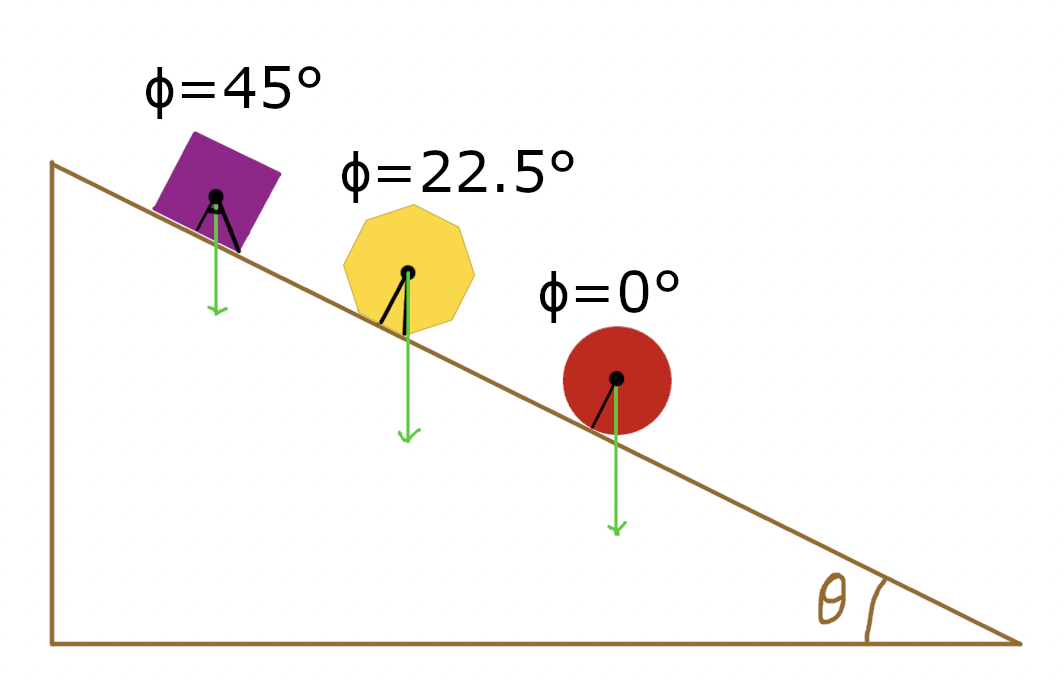
The answer by catalogue_number mentions that a circle, or shapes with a small base (small edges) will tip easier.

Because the concept of forces as arrows, e.g. arrows depicting forces acting on the center of mass might be too abstract, we made some toy model with lego and cardboard as in the image below.

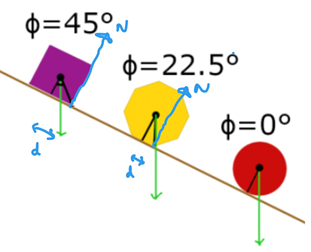
To represent the gravitational force we took a rope with some weight added to it. This looks almost the same as the arrows in the schematic pictures. What we see is that relative to the point where the object touches the surface the object does not tip when the force(/rope) is not 'pulling beyond' it.

(actually, our construction is a bit bad because the hexagon will eventually slide and when it is hitting the bump from the tape it starts to tip)

For the round object, we noticed that the rope is pulling in a different position relative to the point where the object touches the surface. The round object is tipping over very easily.

Experiment 2, energy in rotational motion

Changing the construction as above we used two weights. These weights will also move up and down when the object rotates. This motion makes that the gravitational energy is not only going into the motion along the direction of the slope, but also in up and down motions from the weights.

So despite the shape being round, we saw that there is a way to make it move slower. This is by having part of the motion/energy go into the up and down motions of the weights.

Experiment 3, energy dissipation

In the comments, Ciprian Tomoiagă mentioned a very interesting link to a lecture of Dr. Tadashi Tokieda. We did his experiment with the rice inside the objects.

Like in the second experiment we have again the same shape for the objects. One of the tubes is filled for one-third with rice. Will this change the speed?

Yes it will. The rice inside apparently slows down the motion. It does this without having an effect on the shape of the tube. The rice slows down the tube because it will be pushed up, picking up potential energy, and when it falls down it dissipates the energy.

How does something get to move faster or slow down, which force does this?

The example with the rice made me a bit confused for a moment.

In terms of energy, it can be understood easily. When an object rolls down, gravitational energy is converted into kinetic energy. In two ways we can see differing speeds for the object

• The kinetic energy can be split up into components of the translational movement/speed of the object (along the direction of the slope) and into rotational motion and traverse motions.
• The kinetic energy is lost due to friction and turned into heat.

But how about conservation of momentum? In the third example, the rice gives rise to friction internally, so energy is dissipated. But why should these internal forces make the object slow down? How do the forces between the object and earth and between the object and the surface of the earth change because of this?

I imagine something like the image below.

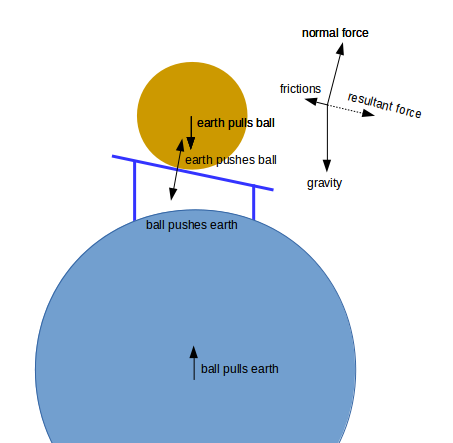
Earths pulls and pushes the ball and vice versa. There will be some resultant net force that makes the ball move relative to earth.

So if something inside the ball causes friction, like the rice, or if something inside the ball takes up some of the energy, like rotational and traverse motions, then why should the ball move slower? What force causes this?

Did these frictions or rotational motions change the resultant force (due to changes in friction or normal force), even when it is the same shape? Well, it must be. We can also see it when the rice example is placed in a static state.

Below we see the forces acting on the object with the rice inside when it is in balance, not moving. There is no torque because the center of mass is above the point of contact. There is a resultant force from the combination of gravity and normal force. If there would be no friction then the object would start to slip. So this static friction force will now be present and it makes the net force zero.

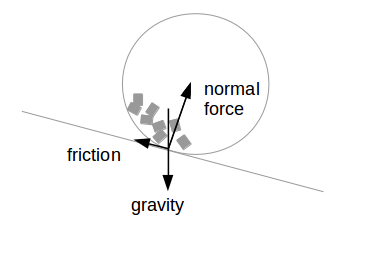
Why would this static friction be gone or smaller when the center of mass is not above the point of contact?

The reason is because now the object can tipple.

We can see this when we imagine doing the experiment in zero gravity and replace the resultant force (from normal force and gravity) by pulling on the object.

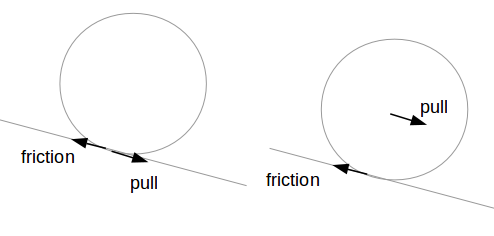
When we pull the sphere in the bottom then we get that the pull force and friction balance each other. When we pull at some higher point then due to the torque the object will start tipping/rolling. But can it accelerate when the net forces are zero? No, but the net forces won't be zero. If the object starts rolling (and accelerates) then the friction will be lower than the force by which we pull.

The friction force is originating from the object pushing the earth/surface, and when it is rolling then it is pushing the earth's surface less. (So this is a more complicated version explaining Walter's quick and intuitive answer)

TL;DR

Round objects roll faster because round objects can actually roll.

By rolling the object is pushing less against the surface, which would create friction and slow it down.

Objects with flat surfaces, even if the center of mass is beyond the tipping point, will have contact with the surface without rolling. Due to inertia the object will be lifted quickly and most of the time the object with flat surfaces is only touching the surface in one point, but this upwards motion is pressing the point of contact of the cube against the surface which will cause friction.

Answer 6

The very premise is dependent on the specific circumstances, in particular the materials involved. Let's ignore the problem investigated by the good answer of catalogue_number about how to get started, and assume we have conditions that make the cube roll, like a sufficiently steep slope or an initial push, identical for ball and cube.

The problem is material dependent because the only reasons either object would be slower is because potential energy is transformed into heat and noise instead of kinetic energy. Without friction or noise, all potential energy is converted into kinetic energy (both rotational and translational), and both objects would have the same speed at the same point on the slope.^1,2 If we imagine a silicone elastomer cube on a slope of the same material we can imagine that it will indeed bounce downhill at a high speed. I have chosen silicone elastomers because they are very elastic, i.e., their deformation creates little heat which is why they bounce very well. It is actually conceivable that a bouncing object loses less energy to friction than a rolling sphere because it doesn't touch the surface as much, especially if we imagine soft surfaces, like fabrics.

With a hard slope a shape with planar surfaces may also lose energy through a process that is not friction: When the surface happens to fall flat on the slope a sound is produced, similar to a book that falls flat on the floor. The sound energy will be lost to the motion. With an elastic, light, bouncy surface like an elastic fabric we can avoid much of that: The slope surface will give and not be flat any more, and the missing resistance and the lack of trapped air will prevent loud noises from emerging.

Bottom line: The losses of a non-spherical shape are through friction and sound and may not be larger than those of the sphere if the materials and circumstances are chosen carefully.

---

¹ The cube would bounce in an irregular fashion and may be at times rotating a bit faster and at other times rotate slower and instead move forward faster, so that statement is referring to the average.

² The physics of bouncing balls are quite interesting, see http://www.physics.usyd.edu.au/~cross/Gripslip.pdf ; it's possible that bouncing objects rotate faster than a rolling ball with the same translational velocity, which would make a bouncing ball generally slower than a rolling one, especially with silicone or similar "superballs". The reason is in the dynamics of the elastic deformation on the area of impact: The force vector during the bounce does not go through the center of mass and thus rotates the ball, in addition to bouncing it. The kinetic energy stored in the rotation would be missing from the translational velocity, making bouncing objects move downhill slower (while rotating faster). But the rotational speed changes from bounce to bounce, indeed the direction reverses, so the translation may just be more erratic and not slower on average. Anybody who has thrown a superball forward towards the ground has seen how the first bounce is slower than expected while the second bounce is faster, owing to the spin after the first bounce; an on-off pattern that continues through subsequent bounces.

Answer 7

To supplement catalogue_number's answer. The first question of 'rolling fast' depends on forces slowing down the motion.

For the cube the line of the normal contact force (from each impact) has a distance $d$ further from the centre than for the octagon and the decelerating torque would be greater. This would cause a rolling cube to be decelerated more quickly than a rolling octagon. For the sphere there is no decelerating torque at all as the line of action of the normal force is through the centre.

You could show your 5 year old about torque or moments, by asking him to push open a door, by pushing near the handle and then by pushing near the hinge. When pushing near the hinge it's much more difficult to get the door to swing open.

Then explain to him, that in the same way, the normal force finds it difficult to slow the object as it's 'pushing near the hinge' for the octagon, but pushing further away for the square.

Answer 8

As a cubeoid and similar object has a large area in contact with the inclined surface or any other surface on which it is kept when an external force is applied from the side to make it topple, due to inertia the object resist change in its state and to balance the torque generated by the external force the normal force shifts across the length of the base and if the magnitude of the force is increased the normal force shifts even further. If the force is increased to such extent that normal force shifts to the extreme and still does not cancel out the torque of the force the object will topple and fall. Now let's talk about disc as in ideal condition the disc is contact with the surface at one point only so there is no length left for the normal to shift so torque of the force instantly make the the object move. Force of magnitude greater then friction can cause linear and rotational motion. In case of square and other similar objects the force has to be greater than friction and torque due to force has to be greater than maximum torque due to shifting of normal to the extreme length of base on contact with surface. As compared to disc this require more force hence it is difficult to roll.

This is the real reason but might not be apt for a 5 year old.

Answer 9

Why does something round roll down faster than something square?

It doesn't necessarily. In particular, a big round object will tend to roll slower than a small square object, assuming the geometry is such that square object rolls. In practice for a cubic object, friction and tumbling effects are going to be significant and slow down the cube extra, but let's ignore those for a minute.

These problems can be effectively calculated and understood by considering conservation of energy. At the start of the rolling, an object of mass $m$ has only gravitational potential energy, approximately $E=U=mgh$. At the bottom of a slope, the object will have both translational and rotational kinetic energy, $E=\frac{1}{2}mv^2+\frac{1}{2}I\omega^2$. $\omega$, the angular rotational speed can be related to $v$ based on the object and restriction that we are undergoing rotation with no sliding. $I$ is the moment of inertia of the object, which varies significantly depending on it's size and geometry.

The main point here, is that different objects (such as spheres vs cubes) have different moments of inertia given the same mass.

The prototypical examples for these types of problems are cylinders vs. spheres, and hollow objects vs. full objects, since all roll smoothly and are therefore easier to visualize and test than squares and octagons. Cylinders and hollow objects have larger moments of inertia given a similar mass.

Similarly, a hollow sphere filled with something dense like water has a larger smaller moment of inertia than a hollow sphere filled with air, and will roll faster as a result. This is why something small and dense like a marble will roll faster than something large and less dense like a beach ball.

So to rigorously answer your question:

What makes an object roll/fall down fast?

Having a relatively small moment of inertia.

At least if we ignore friction and all that other stuff.