1
$\begingroup$

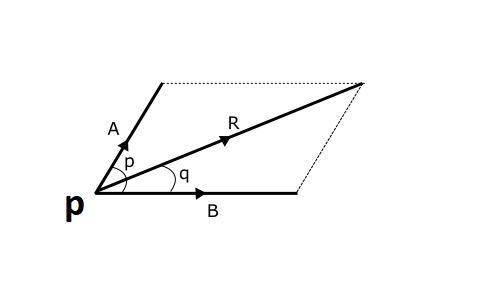
enter image description here

As we all know the basic principle of vector addition. construct a parallelogram as shown, and the diagonal would be the resultant vector. As a mathematical method or formula, it is perfect to define the sum of two vectors in this way, because it is nothing but a binary operation.

But when it comes to practical life, say an object is at $P$, and two force vector $A$ and $B$ is applied on the object. How would you know that the resultant force would act on the object in the direction of vector R? even if so, how do you even know its magnitude is going to be equal to the diagonal length of this parallelogram?

Improve this question
$\endgroup$
3
  • 3
    $\begingroup$ I changed the title of your question to summarize what you ask in the body. You'll be more likely to get helpful responses that way. $\endgroup$
    – Solomon Slow
    Commented Sep 16, 2021 at 20:14
  • 1
    $\begingroup$ P.S., You're kind of looking at the question through the wrong end of the telescope. Mathematicians may have adopted vectors, and constructed a formal algebra around them, but vectors were invented by physicists in order to describe how forces and moving particles actually seem to behave. $\endgroup$
    – Solomon Slow
    Commented Sep 16, 2021 at 20:23
  • 1
    $\begingroup$ That should be an easy experiment. $\endgroup$
    – my2cts
    Commented Sep 16, 2021 at 21:52

3 Answers 3

Reset to default
5
$\begingroup$

As far as I see, it is an experimental fact. Applying the forces on an object through springs, it is possible to know the modulus of the forces by the spring displacements. Knowing also the direction of them, the forces can be modelled as vectors.

It happens that adding them using the parallelogram rule works either for a body at rest (where the vectorial sum of all forces must be zero), or for an accelerated one, where $\mathbf F = m\mathbf a$ holds.

Improve this answer
$\endgroup$
1
$\begingroup$

As usual with forces, the answer depends on which definition of force one is using.

In an approach close to Mach's ideas, forces is defined as $\vec F = m \vec a$. Therefore, the vector character of the force is directly inherited from the acceleration being a vector.

In an approach where forces are considered primitive entities, the equality of forces can be judged by the equilibrium condition. As a consequence, in such an approach the parallelogram rule can be experimentally verified if, in the case of equilibrium in the presence of three forces, each of them is equal and opposiute to the sum of the remaining two.

Improve this answer
$\endgroup$
0
$\begingroup$

Provided that displacement (the measured distance and direction between two points) is a vector, it follows implicitly that force must be a vector.

Given some vector $\vec s$ with some parameter $t$

$m\frac {d^n\vec s}{dt^n}$ is a vector for any scalar m, whole number n.

addendum:

Since unnecessary appeals to Newton are being made, note

$\vec F:=d \vec P/dt$ where $\vec P$ is momentum, and

$\vec P :=m d\vec s/dt$ where

$m = \gamma m_0 = E/c^2$, a scalar, despite its velocity dependence in the Einsteinian formulation.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ That argument also assumes Newton's Second Law, of course. $\endgroup$
    – Michael Seifert
    Commented Sep 16, 2021 at 20:21
  • $\begingroup$ It'll work fine as long as F is the time derivative of momentum, too, momentum being a vector $\endgroup$
    – g s
    Commented Sep 16, 2021 at 20:46

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.