0
$\begingroup$

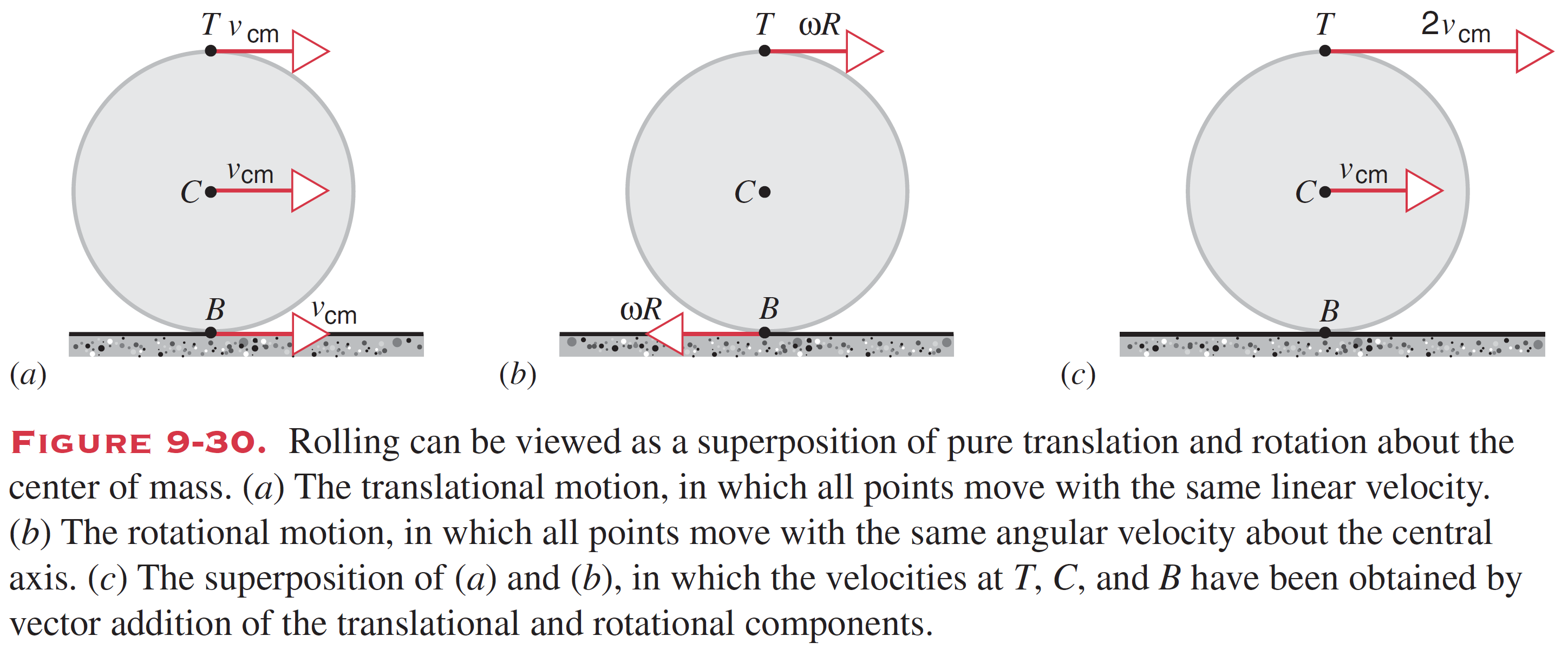
The following picture illustrates the situation of rolling without slipping. I noticed that this is achieved as the direction of $v_{cm}$ is the opposite of $\omega R$, at where the wheel is in contact with the ground. enter image description here

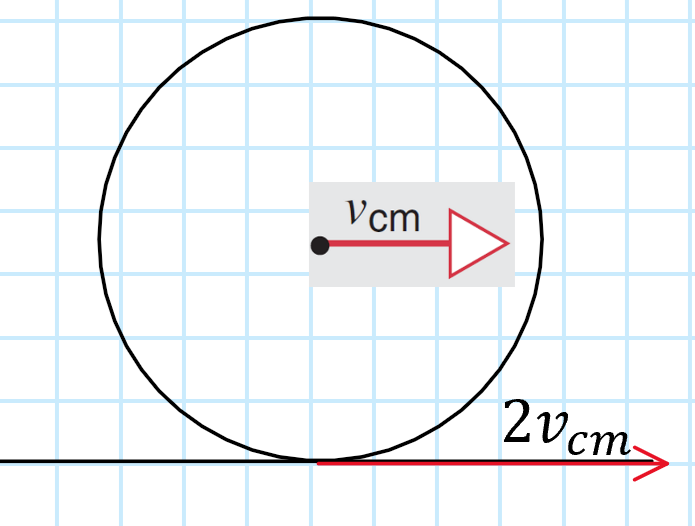
However, I am confused why can't we, for example, rotate the wheel in picture b) in the counterclockwise direction? Then, if we take the superposition of picture a and picture b as we did in picture c, wouldn't the velocity of each part of the wheel look like the following instead? enter image description here

Is the above option viable in real life? If not, why not?

Improve this question
$\endgroup$
3
  • $\begingroup$ Your question is rather unclear. But I will point out that in (a) the velocities are relative to point B, whereas in (b) everything is relative to point C. $\endgroup$
    – DKNguyen
    Commented Jul 17, 2021 at 22:13
  • $\begingroup$ @DKNguyen Hi! I believe the velocities in figure a) are relative to the Earth though as that figure is just showing a wheel set into linear motion? Am I missing anything? $\endgroup$
    – Claire
    Commented Jul 17, 2021 at 22:32
  • $\begingroup$ Is wheel performing moonwalk? :) $\endgroup$
    – ACB
    Commented Jul 19, 2021 at 12:35

2 Answers 2

Reset to default
1
$\begingroup$

If the wheel were turning counterclockwise instead of clockwise, then figures a, b, and c would be the same except that all the directional arrows would be reversed. To be otherwise you could not have rolling without slipping.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Hi! But why can't figure a stays as what it is right now while the wheel is turning ccw? What's preventing this from happening? Or is this also possible in real life, it's just that this wouldn't be called as rolling without slipping? $\endgroup$
    – Claire
    Commented Jul 17, 2021 at 20:45
  • $\begingroup$ @Cheryl If the wheel was moving to the right but rotating to the CCW that would be a case of extreme slipping. Not even like a car tire slipping on a dirt road but more like a grinding wheel against a block of steel where the wheel moves actually moves in the complete opposite direction that the wheel's rotation would otherwise roll it in. $\endgroup$
    – DKNguyen
    Commented Jul 17, 2021 at 22:19
  • $\begingroup$ @DKNguyen I see, Thanks! So the situation that I described actually can happen in the real world? $\endgroup$
    – Claire
    Commented Jul 17, 2021 at 22:30
  • $\begingroup$ @Cheryl Yeah but we don't call it any semblance of rolling since the spinning motion of the wheel is utterly irrelevant to the actual translational motion of the wheel; Something else is pushing the wheel in the other direction, hard. $\endgroup$
    – DKNguyen
    Commented Jul 17, 2021 at 22:32
0
$\begingroup$

Rolling without slipping in that would not not possible on a stationary platform, however on a platform moving with 2v, rolling without slipping can still take place. The whole point of rolling without slipping is that the relative velocity of the bottom most point of the ball and the platform should be zero.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.